Exam 5: Discrete Probability Distributions

According to geologists, the San Francisco Bay Area experiences five earthquakes with a magnitude of 6.5 or greater every 100 years. What is the probability that no earthquakes with a magnitude of 6.5 or greater will strike the San Francisco Bay Area in the next 40 years?

For a particular clothing store, a marketing firm finds that 16% of $10-off coupons delivered by mail are redeemed. Suppose six customers are randomly selected and are mailed $10-off coupons. What is the probability that three of the customers redeem the coupon?

The number of cars sold by a car salesperson during each of the last 25 weeks is the following: 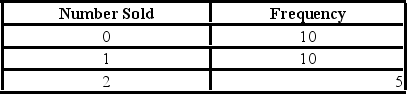What is the standard deviation of the number of cars sold by the salesperson during a week?

Forty-four percent of consumers with credit cards carry balances from month to month. Four consumers with credit cards are randomly selected. What is the probability that fewer than two consumers carry a credit card balance?

Consider the following probability distribution. 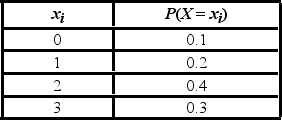The standard deviation is ________.

Due to turnover and promotion, a bank manager knows that, on average, she hires four new tellers per year. Suppose the number of tellers she hires is Poisson distributed. A) What is the probability that in a given year, the manager hires exactly five new tellers? B) What is the average number of tellers the manager hires in a six-month period? C) What is the probability that the manager hires at least one new teller in a given six-month period?

A professor has learned that three students in his class of 20 will cheat on the final exam. He decides to focus his attention on four randomly chosen students during the exam. What is the probability that he finds at least one of the students cheating?

A binomial random variable is defined as the number of successes achieved in n trials of a Bernoulli process.

You have inherited a lottery ticket may be a $10,000 winner. You have a 0.25 chance of winning the $10,000 and a 0.75 chance of winning $0. You have an opportunity to sell the lottery ticket for $2,500. What is your expected return and what should you do if are risk averse?

We can think of the expected value of a random variable X as ________.

Consider the following probability distribution. 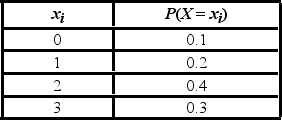The variance is ________.

The expected value of a random variable X can be referred to or denoted as ________.

To illustrate the possible outcomes and their associated probabilities we can construct a probability ________.

Consider the following discrete probability distribution. What is the probability that X is negative?

A consumer who is risk neutral is best characterized as ________.

An investor owns a portfolio consisting of two mutual funds, A and B, with 35% invested in A. The following table lists the inputs for these funds. The expected return for the portfolio return is ________.

A random variable is a function that assigns numerical values to the outcomes of a random experiment.

There are currently 18 pit bulls at the pound. Of the 18 pit bulls, four have attacked another dog in the last year. Joe, a member of the staff, randomly selects six of the pit bulls for his group. What is the probability that at least one of the pit bulls in Joe's group attacked another dog last year?

Consider the following cumulative distribution function for the discrete random variable X. What is the probability that X is less than or equal to 2?

Which of the following can be represented by a discrete random variable?