Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 18: Time Series and Forecasting

arrow
Exam 1: Statistics and Data102 Questionsadd course
Exam 2: Tabular and Graphical Methods123 Questionsadd course
Exam 3: Numerical Descriptive Measures152 Questionsadd course
Exam 4: Introduction to Probability148 Questionsadd course
Exam 5: Discrete Probability Distributions158 Questionsadd course
Exam 6: Continuous Probability Distributions143 Questionsadd course
Exam 7: Sampling and Sampling Distributions136 Questionsadd course
Exam 8: Interval Estimation131 Questionsadd course
Exam 9: Hypothesis Testing116 Questionsadd course
Exam 10: Statistical Inference Concerning Two Populations131 Questionsadd course
Exam 11: Statistical Inference Concerning Variance120 Questionsadd course
Exam 12: Chi-Square Tests120 Questionsadd course
Exam 13: Analysis of Variance120 Questionsadd course
Exam 14: Regression Analysis140 Questionsadd course
Exam 15: Inference With Regression Models125 Questionsadd course
Exam 16: Regression Models for Nonlinear Relationships118 Questionsadd course
Exam 17: Regression Models With Dummy Variables130 Questionsadd course
Exam 18: Time Series and Forecasting125 Questionsadd course
Exam 19: Returns, Index Numbers, and Inflation120 Questionsadd course
Exam 20: Nonparametric Tests120 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

Which of the following is a causal forecasting method?

Free
(Multiple Choice)
4.9/5
(36)
Question 1
Correct Answer:
Verified

D

Prices of crude oil have been steadily rising over the last two years (The Wall Street Journal, December 14, 2010). The monthly data on price per gallon of unleaded regular gasoline in the United States from January 2009 to December 2010 were available. Three trend models were created starting with t = 1 and the following output was generated. Prices of crude oil have been steadily rising over the last two years (The Wall Street Journal, December 14, 2010). The monthly data on price per gallon of unleaded regular gasoline in the United States from January 2009 to December 2010 were available. Three trend models were created starting with t = 1 and the following output was generated. Using the cubic trend equation, which of the following would be the forecast for the price of regular unleaded gasoline for January 2011? Using the cubic trend equation, which of the following would be the forecast for the price of regular unleaded gasoline for January 2011?

Free
(Multiple Choice)
4.9/5
(32)
Question 2
Correct Answer:
Verified

C

Which of the following is not true of a time series with a cyclical component?

Free
(Multiple Choice)
4.8/5
(34)
Question 3
Correct Answer:
Verified

D

Based on quarterly data collected over the last five years, the following regression equation was found to forecast the quarterly demand for the number of new copies of a business statistics textbook: Based on quarterly data collected over the last five years, the following regression equation was found to forecast the quarterly demand for the number of new copies of a business statistics textbook: <sub>t</sub> = 2,298 - 335Qtr1 - 1,446Qtr2 + 303Qtr3 + 26t, where Qtr1, Qtr2, and Qtr3 are dummy variables corresponding to Quarters 1, ,2 and 3, and t = time period starting with t = 1. For a given year, the demand in Quarter 4 is on average ________. t = 2,298 - 335Qtr1 - 1,446Qtr2 + 303Qtr3 + 26t, where Qtr1, Qtr2, and Qtr3 are dummy variables corresponding to Quarters 1, ,2 and 3, and t = time period starting with t = 1. For a given year, the demand in Quarter 4 is on average ________.

(Multiple Choice)
4.7/5
(27)
Question 4

When the model yt = Tt × St × It is assumed, to identify the ________, we have to find the seasonally adjusted series.

(Multiple Choice)
4.8/5
(45)
Question 5

The past monthly demands are shown below. The naïve method, that is, the one-period moving average method, is applied to make forecasts. The past monthly demands are shown below. The naïve method, that is, the one-period moving average method, is applied to make forecasts. 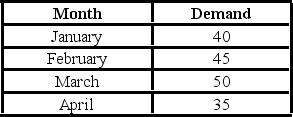If the demand in May appears to be 35, what is the residual (error) for May? If the demand in May appears to be 35, what is the residual (error) for May?

(Multiple Choice)
4.8/5
(33)
Question 6

Given the estimated model Given the estimated model <sub>t</sub> = b<sub>0</sub> + b<sub>1</sub>t, the one-step-ahead and multistep-ahead forecasts are possible, if we know or can predict, future values of the ________. t = b0 + b1t, the one-step-ahead and multistep-ahead forecasts are possible, if we know or can predict, future values of the ________.

(Multiple Choice)
4.8/5
(38)
Question 7

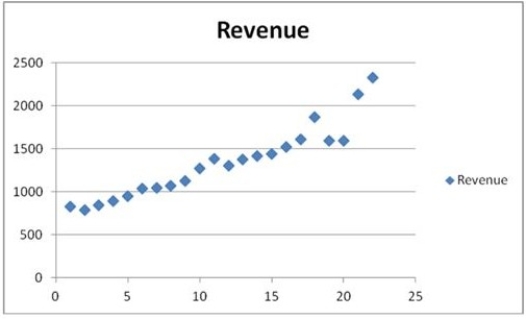The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. Which of the following is a revenue forecast for 2012 found by the exponential trend equation? 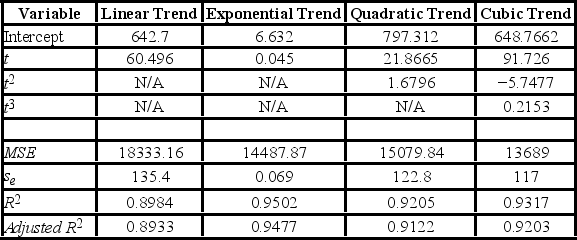The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. Which of the following is a revenue forecast for 2012 found by the exponential trend equation? The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. Which of the following is a revenue forecast for 2012 found by the exponential trend equation? Which of the following is a revenue forecast for 2012 found by the exponential trend equation?

(Multiple Choice)
4.8/5
(38)
Question 8

________ patterns are caused by the presence of a random error term.

(Short Answer)
4.8/5
(30)
Question 9

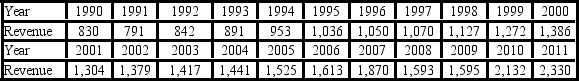
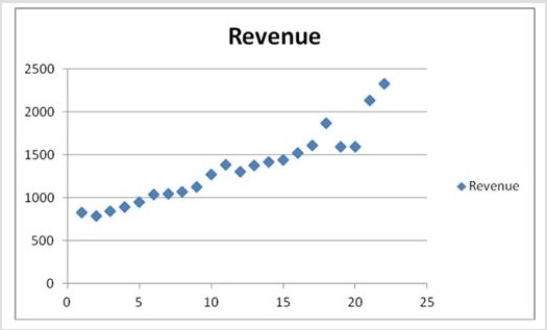The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. Which of the following is an exponential trend equation? 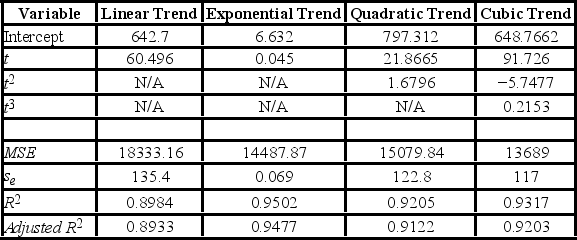The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. Which of the following is an exponential trend equation? The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The scatterplot indicates that the annual revenues have an increasing trend. Linear, exponential, quadratic, and cubic models were fit to the data starting with t = 1, and the following output was generated. Which of the following is an exponential trend equation? Which of the following is an exponential trend equation?

(Multiple Choice)
4.8/5
(24)
Question 10

The linear trend model, The linear trend model, <sub>t</sub> = 660 + 2t starting with t = 1, for seasonally adjusted monthly sales was estimated using monthly data from for five years. Furthermore, the seasonal index for October was found to be 0.92. Which of the following is the forecast for the month of October next year? t = 660 + 2t starting with t = 1, for seasonally adjusted monthly sales was estimated using monthly data from for five years. Furthermore, the seasonal index for October was found to be 0.92. Which of the following is the forecast for the month of October next year?

(Multiple Choice)
4.7/5
(40)
Question 11

When a time series is analyzed by the model yt = Tt × St × It, which of the following is valid?

(Multiple Choice)
4.9/5
(36)
Question 12

When comparing which of the following trend models is the adjusted R2 not used?

(Multiple Choice)
4.9/5
(38)
Question 13

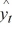When the exponential trend model is used to make forecasts, the forecasts are given by When the exponential trend model is used to make forecasts, the forecasts are given by = exp(b<sub>0</sub> + b<sub>1</sub>t + /2). = exp(b0 + b1t + 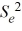When the exponential trend model is used to make forecasts, the forecasts are given by = exp(b<sub>0</sub> + b<sub>1</sub>t + /2). /2).

(True/False)
4.8/5
(35)
Question 14

When comparing polynomial trend models, we use adjusted R2, which imposes a penalty for ________.

(Short Answer)
4.7/5
(32)
Question 15

The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The autoregressive models of order 1 and 2, yt = β0 + β1yt - 1 + εt, and yt = β0 + β1yt - 1 + β2yt - 2 + εt, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. 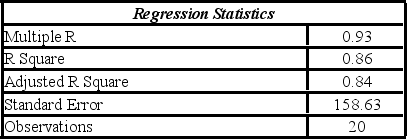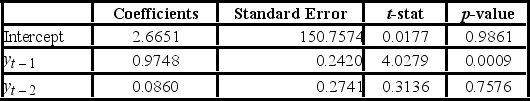Model AR(1): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Model AR(2): The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. When for AR(1), H0: β0 = 0 is tested against HA: β0 ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model yt = β1yt-1 + εt might be an alternative to the AR(1) model yt = β0 + β1yt-1 + εt. Partial output for this simplified model is as follows: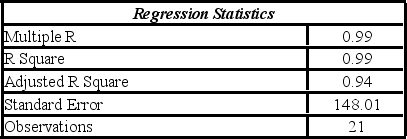 The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. The following table shows the annual revenues (in millions of dollars) of a pharmaceutical company over the period 1990-2011. The autoregressive models of order 1 and 2, y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + ε<sub>t</sub>, and y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t - </sub><sub>1</sub> + β<sub>2</sub>y<sub>t - 2</sub> + ε<sub>t</sub>, were applied on the time series to make revenue forecasts. The relevant parts of Excel regression outputs are given below. Model AR(1): Model AR(2): When for AR(1), H<sub>0</sub>: β<sub>0</sub> = 0 is tested against H<sub>A</sub>: β<sub>0</sub> ≠ 0, the p-value of this t test shown the output as 0.9590. This could suggest that the model y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1 </sub>+ ε<sub>t</sub> might be an alternative to the AR(1) model y<sub>t</sub> = β<sub>0</sub> + β<sub>1</sub>y<sub>t-1</sub> + ε<sub>t</sub>. Partial output for this simplified model is as follows: Find the revenue forecast for 2012 through the use of y<sub>t</sub> = β<sub>1</sub>y<sub>t</sub><sub>-1</sub> + ε<sub>t</sub>. Find the revenue forecast for 2012 through the use of yt = β1yt-1 + εt.

(Essay)
4.8/5
(40)
Question 16

In the quadratic trend model, yt = β0 + β1t 2 + εt, which coefficient determines if the trend is going to be U-shaped or inverted U-shaped?

(Multiple Choice)
4.8/5
(28)
Question 17

Ideally, the chosen model is best in terms of its in-sample predictability and its ________ forecasting ability.

(Short Answer)
4.8/5
(22)
Question 18

Which of the following R functions is used to do the exponential smoothing method?

(Multiple Choice)
4.7/5
(43)
Question 19

Which of the following is a criticism made of qualitative forecasts?

(Multiple Choice)
4.7/5
(35)
Question 20
Showing 1 - 20 of 125
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)