Exam 30: Applications and Models
Exam 1: Rectangular Coordinates69 Questions
Exam 2: Graphs of Equations63 Questions
Exam 3: Linear Equations in Two Variables61 Questions
Exam 4: Functions53 Questions
Exam 5: Analyzing Graphs of Functions56 Questions
Exam 6: A Library of Parent Functions50 Questions
Exam 7: Transformations of Functions32 Questions
Exam 8: Combinations of Functions Composite Functions58 Questions
Exam 9: Inverse Functions59 Questions
Exam 10: Mathematical Modeling and Variation49 Questions
Exam 11: Quadratic Functions and Models61 Questions
Exam 12: Polynomial Functions of Higher Degree63 Questions
Exam 13: Polynomial and Synthetic Division76 Questions
Exam 14: Complex Numbers59 Questions
Exam 15: Zeros of Polynomial Functions49 Questions
Exam 16: Rational Functions96 Questions
Exam 17: Nonlinear Inequalities56 Questions
Exam 18: Exponential Functions and Their Graphs59 Questions
Exam 19: Logarithmic Functions and Their Graphs64 Questions
Exam 20: Properties of Logarithms57 Questions
Exam 21: Exponential and Logarithmic Equations51 Questions
Exam 22: Exponential and Logarithmic Models56 Questions
Exam 23: Radian and Degree Measure52 Questions
Exam 24: Trigonometric Functions The Unit Circle50 Questions
Exam 25: Right Triangle Trigonometry56 Questions
Exam 26: Trigonometric Functions of Any Angle53 Questions
Exam 27: Graphs of Sine and Cosine Functions37 Questions
Exam 28: Graphs of Other Trigonometric Functions51 Questions
Exam 29: Inverse Trigonometric Functions50 Questions
Exam 30: Applications and Models52 Questions
Exam 31: Using Fundamental Identities60 Questions
Exam 32: Verifying Trigonometric Equations46 Questions
Exam 33: Solving Trigonometric Equations54 Questions
Exam 34: Sum and Difference Formulas62 Questions
Exam 35: Multiple Angle and Product to Sum Formulas50 Questions
Exam 36: Law of Sines43 Questions
Exam 37:Law of Cosines43 Questions
Exam 38:Vectors in the Plane50 Questions
Exam 39:Vectors and Dot Products67 Questions
Exam 40: Trigonometric Form of a Complex Number104 Questions
Exam 41: Linear and Nonlinear Systems of Equations58 Questions
Exam 42: Two Variable Linear Systems49 Questions
Exam 43: Multivariable Linear Systems54 Questions
Exam 44: Partial Fractions48 Questions
Exam 45: Systems of Inequalities50 Questions
Exam 46: Linear Programming50 Questions
Exam 47: Matrices and Systems of Equations65 Questions
Exam 48: Operations With Matrices59 Questions
Exam 49: The Inverse of a Square Matrix59 Questions
Exam 50: The Determinant of a Square Matrix52 Questions
Exam 51: Applications of Matrices and Determinants54 Questions
Exam 52: Sequences and Series68 Questions
Exam 53: Arithmetic Sequences and Partial Sums52 Questions
Exam 54: Geometric Sequences and Series67 Questions
Exam 55: Mathematical Induction48 Questions
Exam 56: The Binomial Theorem67 Questions
Exam 57: Counting Principles55 Questions
Exam 58: Probability47 Questions
Exam 59: Lines50 Questions
Exam 60: Introduction to Conics Parabolas124 Questions
Exam 61: Ellipses68 Questions
Exam 62: Hyperbolas62 Questions
Exam 63: Rotation of Conics52 Questions
Exam 64: Parametric Equations50 Questions
Exam 65: Polar Coordinates50 Questions
Exam 66: Polar Equations of Conics50 Questions
Exam 67: Graphs of Polar Equations49 Questions
Select questions type
A Global Positioning System satellite orbits a = 13,000 miles above Earth's surface (see figure).Find the angle of depression from the satellite to the horizon.Assume the radius of Earth is 4000 miles.Round your answer to two decimal places. 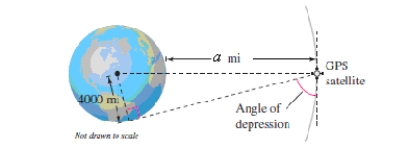
Free
(Multiple Choice)
4.8/5  (28)
(28)
Correct Answer:
D
If and ,determine the value of B.Round to two decimal places. 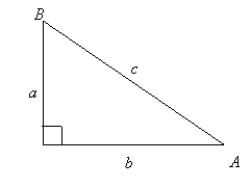
Free
(Multiple Choice)
4.9/5  (42)
(42)
Correct Answer:
B
A police department has set up a speed enforcement zone on a straight length of highway.A patrol car is parked parallel to the zone,a = 210 feet from one end and b = 130 feet from the other end (see figure). 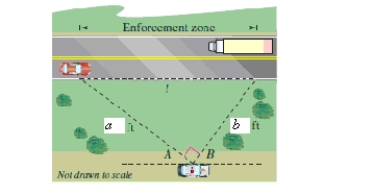 Find the length l of the zone.Round your answer to two decimal places.
Find the length l of the zone.Round your answer to two decimal places.
Free
(Multiple Choice)
4.7/5  (40)
(40)
Correct Answer:
D
If the sides of a rectangular solid are as shown,and ,determine the angle,?,between the diagonal of the base of the solid and the diagonal of the solid.Round answer to two decimal places. 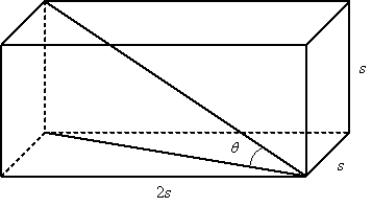
(Multiple Choice)
4.8/5  (42)
(42)
A land developer wants to find the distance across a small lake in the middle of his proposed development.The bearing from A to B is .The developer leaves point A and travels 74 yards perpendicular to to point C.The bearing from C to point B is .Determine the distance,AB,across the small lake.Round distance to nearest yard. 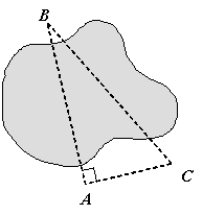
(Multiple Choice)
4.8/5  (45)
(45)
The designers of a water park are creating a new slide and have sketched some preliminary drawings.The length of the ladder is a = 24 feet,and its angle of elevation is 60º (see figure). 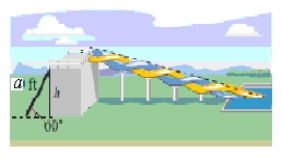
Find the height of the slide.Round your answer to two decimal places.
Find the height of the slide.Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (45)
(45)
The sun is 20º above the horizon.Find the length of a shadow cast by a park statue that is 30 feet tall.Approximate the answer to two decimal places.
(Multiple Choice)
4.8/5  (28)
(28)
A cellular telephone tower that is 150 feet tall is placed on top of a mountain that is 1200 feet above sea level.What is the angle of depression from the top of the tower to a cell phone user who is 6 horizontal miles away and 400 feet above sea level? Round your answer to two decimal places.
(Multiple Choice)
4.7/5  (34)
(34)
A point on the end of a tuning fork moves in simple harmonic motion described by? ? Find ? given that the tuning fork for middle C has a frequency 270 of vibrations per second.
?
(Multiple Choice)
4.9/5  (41)
(41)
Find the altitude of the isosceles triangle shown in the figure.Round your answer to two decimal places.? 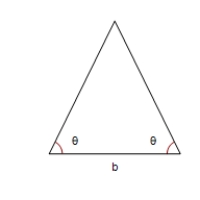 ?
?
(Multiple Choice)
4.7/5  (31)
(31)
Find the altitude of the isosceles triangle shown below if and .Round answer to two decimal places. 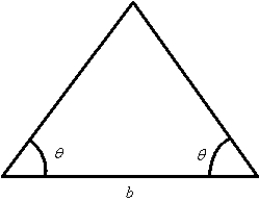
(Multiple Choice)
4.9/5  (27)
(27)
A ball that is bobbing up and down on the end of a spring has a maximum displacement of 3 inches.Its motion (in ideal conditions)is modeled by? where y is measured in feet and t is the time in seconds. What is the period of the oscillations?
?
(Multiple Choice)
4.8/5  (29)
(29)
After leaving the runway,a plane's angle of ascent is 17o and its speed is 278 feet per second.How many minutes will it take for the airplane to climb to a height of 11,500 feet? Round answer to two decimal places.
(Multiple Choice)
4.9/5  (35)
(35)
For the simple harmonic motion described by the trigonometric function,find the maximum displacement.? ?
(Multiple Choice)
4.8/5  (30)
(30)
The sun is 25º above the horizon.Find the length of a shadow cast by a building that is feet tall (see figure).Approximate the answer to two decimal places.? 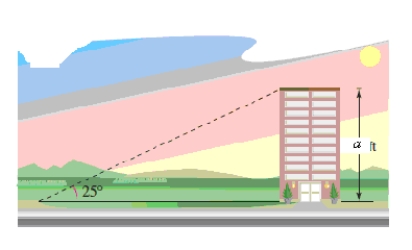 ?
?
(Multiple Choice)
4.9/5  (43)
(43)
Find a model for simple harmonic motion satisfying the specified conditions. ?
Displacement (t = 0): 0
Amplitude: 5 centimeters
Period: 2 seconds
?
(Multiple Choice)
4.8/5  (34)
(34)
For the simple harmonic motion described by the trigonometric function,find the least positive value of t for which d = 0. ?? ?
(Multiple Choice)
4.8/5  (46)
(46)
The height of an outdoor basketball backboard is feet,and the backboard casts a shadow feet long. Find the angle of elevation of the sun.Round your answer to one decimal place.
?
(Multiple Choice)
4.9/5  (42)
(42)
For the simple harmonic motion described by the trigonometric function,find the maximum displacement.? ?
(Multiple Choice)
4.9/5  (44)
(44)
For the simple harmonic motion described by the trigonometric function,find the maximumvalue of d when .? Round your answer to nearest whole number. ?
(Multiple Choice)
4.8/5  (24)
(24)
Showing 1 - 20 of 52
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)