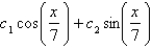Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 17: Second-Order Differential Equations

arrow
Exam 1: Functions and Limits117 Questionsadd course
Exam 2: Derivatives151 Questionsadd course
Exam 3: Applications of Differentiation153 Questionsadd course
Exam 4: Integrals95 Questionsadd course
Exam 5: Applications of Integration120 Questionsadd course
Exam 6: Inverse Functions127 Questionsadd course
Exam 7: Techniques of Integration124 Questionsadd course
Exam 8: Further Applications of Integration86 Questionsadd course
Exam 9: Differential Equations67 Questionsadd course
Exam 10: Parametric Equations and Polar Coordinates72 Questionsadd course
Exam 11: Infinite Sequences and Series158 Questionsadd course
Exam 12: Vectors and the Geometry of Space60 Questionsadd course
Exam 13: Vector Functions93 Questionsadd course
Exam 14: Partial Derivatives132 Questionsadd course
Exam 15: Multiple Integrals124 Questionsadd course
Exam 16: Vector Calculus137 Questionsadd course
Exam 17: Second-Order Differential Equations63 Questionsadd course
Exam 18: Final Exam44 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

Suppose a spring has mass M and spring constant k and let Suppose a spring has mass M and spring constant k and let . Suppose that the damping constant is so small that the damping force is negligible. If an external force is applied (the applied frequency equals the natural frequency), use the method of undetermined coefficients to find the equation that describes the motion of the mass. . Suppose that the damping constant is so small that the damping force is negligible. If an external force Suppose a spring has mass M and spring constant k and let . Suppose that the damping constant is so small that the damping force is negligible. If an external force is applied (the applied frequency equals the natural frequency), use the method of undetermined coefficients to find the equation that describes the motion of the mass. is applied (the applied frequency equals the natural frequency), use the method of undetermined coefficients to find the equation that describes the motion of the mass.

Free
(Multiple Choice)
4.9/5
(36)
Question 1
Correct Answer:
Verified

A

Solve the initial-value problem. Solve the initial-value problem.

Free
(Multiple Choice)
4.8/5
(25)
Question 2
Correct Answer:
Verified

B

Solve the differential equation. Solve the differential equation.

Free
(Essay)
4.9/5
(40)
Question 3
Correct Answer:
Verified

Solve the differential equation using the method of variation of parameters. Solve the differential equation using the method of variation of parameters.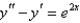

(Multiple Choice)
5.0/5
(39)
Question 4

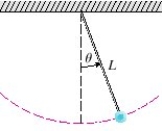
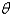The figure shows a pendulum with length L and the angle The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation from the vertical to the pendulum. It can be shown that The figure shows a pendulum with length L and the angle from the vertical to the pendulum. 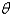It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation , as a function of time, satisfies the nonlinear differential equation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation where The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where we can use the linear approximation The figure shows a pendulum with length L and the angle from the vertical to the pendulum. It can be shown that , as a function of time, satisfies the nonlinear differential equation where 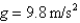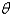we can use the linear approximation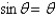

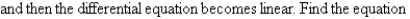
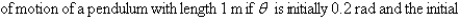
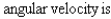
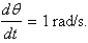
(Multiple Choice)
4.8/5
(30)
Question 5

Use power series to solve the differential equation. Use power series to solve the differential equation.

(Multiple Choice)
4.8/5
(33)
Question 6

Solve the differential equation using the method of variation of parameters. Solve the differential equation using the method of variation of parameters.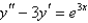

(Essay)
4.9/5
(44)
Question 7

Find a trial solution for the method of undetermined coefficients. Do not determine the coefficients. Find a trial solution for the method of undetermined coefficients. Do not determine the coefficients.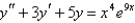

(Multiple Choice)
4.9/5
(33)
Question 8

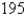A spring with a mass of A spring with a mass of kg has damping constant 28 and spring constant . Find the damping constant that would produce critical damping. kg has damping constant 28 and spring constant A spring with a mass of kg has damping constant 28 and spring constant . Find the damping constant that would produce critical damping. . Find the damping constant that would produce critical damping.

(Multiple Choice)
4.9/5
(26)
Question 9

Solve the differential equation using the method of undetermined coefficients. Solve the differential equation using the method of undetermined coefficients.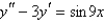

(Multiple Choice)
4.8/5
(39)
Question 10

Solve the differential equation. Solve the differential equation.

(Multiple Choice)
4.8/5
(40)
Question 11

Use power series to solve the differential equation. Use power series to solve the differential equation.

(Multiple Choice)
4.8/5
(41)
Question 12

Solve the differential equation. Solve the differential equation.

(Multiple Choice)
4.9/5
(26)
Question 13

Solve the differential equation using the method of undetermined coefficients. Solve the differential equation using the method of undetermined coefficients.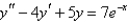

(Essay)
4.9/5
(39)
Question 14

Solve the differential equation using the method of variation of parameters. Solve the differential equation using the method of variation of parameters.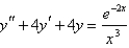

(Multiple Choice)
4.9/5
(29)
Question 15

Solve the initial-value problem. Solve the initial-value problem. . .

(Multiple Choice)
4.8/5
(33)
Question 16

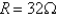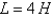A series circuit consists of a resistor A series circuit consists of a resistor , an inductor with , a capacitor with , and a -V battery. If the initial charge is 0.0008 C and the initial current is 0, find the current I(t) at time t. , an inductor with A series circuit consists of a resistor , an inductor with , a capacitor with , and a -V battery. If the initial charge is 0.0008 C and the initial current is 0, find the current I(t) at time t. 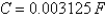, a capacitor with A series circuit consists of a resistor , an inductor with , a capacitor with , and a -V battery. If the initial charge is 0.0008 C and the initial current is 0, find the current I(t) at time t. 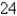, and a A series circuit consists of a resistor , an inductor with , a capacitor with , and a -V battery. If the initial charge is 0.0008 C and the initial current is 0, find the current I(t) at time t. -V battery. If the initial charge is 0.0008 C and the initial current is 0, find the current I(t) at time t.

(Multiple Choice)
4.9/5
(30)
Question 17

Solve the differential equation. Solve the differential equation.

(Essay)
4.7/5
(33)
Question 18

Solve the differential equation using the method of undetermined coefficients. Solve the differential equation using the method of undetermined coefficients.

(Essay)
4.7/5
(43)
Question 19

Solve the differential equation using the method of variation of parameters. Solve the differential equation using the method of variation of parameters.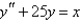

(Essay)
4.9/5
(41)
Question 20
Showing 1 - 20 of 63
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)