Exam 1: Functions and Limits
Exam 1: Functions and Limits117 Questions
Exam 2: Derivatives151 Questions
Exam 3: Applications of Differentiation153 Questions
Exam 4: Integrals95 Questions
Exam 5: Applications of Integration120 Questions
Exam 6: Inverse Functions127 Questions
Exam 7: Techniques of Integration124 Questions
Exam 8: Further Applications of Integration86 Questions
Exam 9: Differential Equations67 Questions
Exam 10: Parametric Equations and Polar Coordinates72 Questions
Exam 11: Infinite Sequences and Series158 Questions
Exam 12: Vectors and the Geometry of Space60 Questions
Exam 13: Vector Functions93 Questions
Exam 14: Partial Derivatives132 Questions
Exam 15: Multiple Integrals124 Questions
Exam 16: Vector Calculus137 Questions
Exam 17: Second-Order Differential Equations63 Questions
Exam 18: Final Exam44 Questions
Select questions type
Many physical quantities are connected by inverse square laws, that is, by power functions of the form 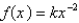 . In particular, the illumination of an object by a light source is inversely proportional to the square of the distance from the source. Suppose that after dark you are in a room with just one lamp and you are trying to read a book. The light is too dim and so you move
. In particular, the illumination of an object by a light source is inversely proportional to the square of the distance from the source. Suppose that after dark you are in a room with just one lamp and you are trying to read a book. The light is too dim and so you move 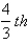 the distance to the lamp. How much brighter is the light?
the distance to the lamp. How much brighter is the light?
(Multiple Choice)
4.8/5  (39)
(39)
Find the domain and sketch the graph of the function. What is its range? 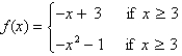
(Multiple Choice)
4.8/5  (35)
(35)
Classify the function as a Polynomial function, a Trigonometric function, an algebraic function, or other. 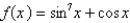
(Multiple Choice)
4.7/5  (22)
(22)
By graphing the function  and zooming in toward the point where the graph crosses the y-axis, estimate the value of
and zooming in toward the point where the graph crosses the y-axis, estimate the value of 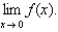
(Essay)
4.8/5  (41)
(41)
Consider the following function. 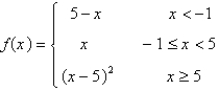 Determine the values of a for which
Determine the values of a for which 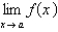 exists.
exists.
(Essay)
4.7/5  (39)
(39)
Complete the table by computing 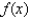 at the given values of x, accurate to five decimal places. Use the results to guess at the indicated limit, if it exists, to three decimal places.
at the given values of x, accurate to five decimal places. Use the results to guess at the indicated limit, if it exists, to three decimal places. 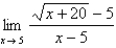
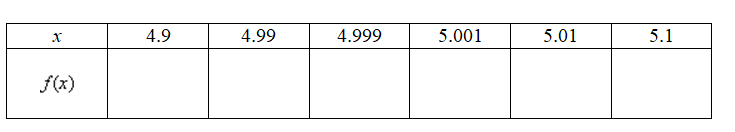
(Essay)
4.9/5  (32)
(32)
If a rock is thrown upward on the planet Mars with a velocity of 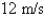 , its height in meters t seconds later is given by
, its height in meters t seconds later is given by 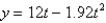 . Find the average velocity over the time interval
. Find the average velocity over the time interval 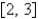 .
.
(Multiple Choice)
4.8/5  (32)
(32)
A machinist is required to manufacture a circular metal disk with area 1000 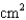 . If the machinist is allowed an error tolerance of
. If the machinist is allowed an error tolerance of 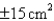 in the area of the disk, how close to the ideal radius must the machinist control the radius? Round your answer to the nearest hundred thousandth.
in the area of the disk, how close to the ideal radius must the machinist control the radius? Round your answer to the nearest hundred thousandth.
(Multiple Choice)
4.9/5  (38)
(38)
Graph the function by hand, not by plotting points, but by starting with the graph of one of the standard functions and then applying the appropriate transformations. 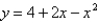
(Multiple Choice)
4.7/5  (32)
(32)
Showing 101 - 117 of 117
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)