Exam 5: Probability

Messenger Service: Three messenger services deliver to a small town in Oregon. Service A has 60% of all the scheduled deliveries, service B has 30%, and service C has the remaining 10%. Their on-time rates are 80%, 60%, and 40% respectively. Define event O as a service delivers a package on time. -If a package was delivered 40 minutes late, what is the probability that it was service C?

If P(A) = 0.25 and P(B) = 0.65, then P(A and B) is:

Club Members: A survey of a club's members indicates that 50% own a home, 80% own a car, and 90% of the homeowners who subscribe also own a car. -What is the probability that a club member owns a car or a house, or both?

If two equally likely events A and B are mutually exclusive and collectively exhaustive, what is the probability that event A occurs?

Financial Consultants: A Financial Consultant has classified his clients according to their gender and the composition of their investment portfolio (primarily bonds, primarily stocks, or a balanced mix of bonds and stocks). The proportions of clients falling into the various categories are shown in the following table: 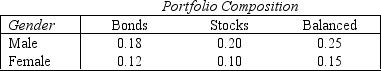One client is selected at random, and two events A and B are defined as follows: A: The client selected is male. B: The client selected has a balanced portfolio. -Find the following probabilities: A. P(A) B. P(B)

If A and B are two independent events with P(A) = 0.9 and P(B|A) = 0.5, then P(A and B) = 0.45.

Which of the following statements is correct if the events A and B have nonzero probabilities?

If A and B are independent events with P(A) = .40 and P(B) = .50, then P(A and B) = .20.

Gender and Marital Status: An insurance company has collected the following data on the gender and marital status of 300 customers. Suppose that a customer is selected at random. -Is marital status independent of gender? Explain using probabilities.

If two events are collectively exhaustive, what is the probability that both occur at the same time?

If the outcome of event A is not affected by event B, then events A and B are said to be

Prior probability of an event is the probability of the event before any information affecting it is given.

Construction Bids: A construction company has submitted bids on two separate state contracts, A and B. The company feels that it has a 60% chance of winning contract A, and a 50% chance of winning contract B. Furthermore, the company believes that it has an 80% chance of winning contract A if it wins contract B. -What is the probability that the company will win neither contract?

GPA and Class: A college professor classifies his students according to their grade point average (GPA) and their class rank. GPA is on a 0.0-4.0 scale, and class rank is defined as the under class (freshmen and sophomores) and the upper class (juniors and seniors). One student is selected at random. -What is the probability that the student is in the lower class and has GPA over 3.0?

Drunk Drivers: Five hundred accidents that occurred on a Saturday night were analyzed. Two items noted were the number of vehicles involved and whether alcohol played a role in the accident. The numbers are shown below: 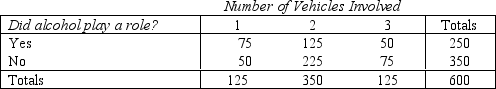-What proportion of accidents involved alcohol and single vehicle?

Prior probabilities can be calculated using the multiplication rule for mutually exclusive events.

Two or more events are said to be independent when the occurrence of one event has no effect on the probability that another will occur.

A random experiment is an action or process that leads to one of several possible ____________________.

If two events are mutually exclusive, what is the probability that both occur at the same time?

The probability of the union of two mutually exclusive events A and B is 0.