Exam 6: Random Variables and Discrete Probability Distributions

An auto insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for automobile insurance. The number of claims a person has made in the last 3 years is an example of a(n) ____________________ random variable.

The monthly sales at a Gas Station have a mean of $50,000 and a standard deviation of $6,000. Profits are calculated by multiplying sales by 40% and subtracting fixed costs of $12,000. Find the mean and standard deviation of monthly profits.

Shopping Outlet: A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below. 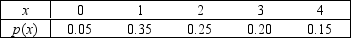-Find the expected value of the number of stores entered.

The time required to drive from New York to New Mexico is a discrete random variable.

Shopping Outlet: A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below. -What did you notice about the mean, variance, and standard deviation of Y = 2X + 1 in terms of the mean, variance, and standard deviation of X?

In each trial of a binomial experiment, there are ____________________ possible outcomes.

Number of Motorcycles: The probability distribution of a discrete random variable X is shown below, where X represents the number of motorcycles owned by a family. -Find the expected value of X.

Sports Fans: Suppose that past history shows that 5% of college students are sports fans. A sample of 10 students is to be selected. -Find the probability that more than 1 student is a sports fan.

The expected number of heads in 100 tosses of an unbiased coin is

For a random variable X, E(X + 2) - 5 = E(X) -3, where E refers to the expected value.

Which of the following is not a characteristic of a binomial experiment?

In Poisson experiment, the probability of more than one success in an interval approaches ____________________ as the interval becomes smaller.

If the probability of success p remains constant in a binomial distribution, an increase in n will increase the variance.

The Poisson distribution is applied to events for which the probability of occurrence over a given span of time, space, or distance is very small.

Given a Poisson random variable X, where the average number of successes occurring in a specified interval is 1.8, then P(X = 0) is:

Unsafe Levels of Radioactivity: The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year. -Find the variance of the number of incidents in one year.

Find the mean $\mu$ and the standard deviation $\sigma$ of this distribution.

Montana Highways: A recent survey in Montana revealed that 60% of the vehicles traveling on highways, where speed limits are posted at 70 miles per hour, were exceeding the limit. Suppose you randomly record the speeds of ten vehicles traveling on US 131 where the speed limit is 70 miles per hour. Let X denote the number of vehicles that were exceeding the limit. -Find P(3 $\le$ X $\le$ 6).

Number of Horses: The random variable X represents the number of horses per family in a rural area in Iowa, with the probability distribution: p(x) = 0.05x, x = 2, 3, 4, 5, or 6. -Find the expected number of horses per family.

Montana Highways: A recent survey in Montana revealed that 60% of the vehicles traveling on highways, where speed limits are posted at 70 miles per hour, were exceeding the limit. Suppose you randomly record the speeds of ten vehicles traveling on US 131 where the speed limit is 70 miles per hour. Let X denote the number of vehicles that were exceeding the limit. -What is the distribution of X?