Exam 6: Applications of the Definite Integral
Exam 1: Preliminaries143 Questions
Exam 2: Limits and Continuity125 Questions
Exam 3: Differentiation150 Questions
Exam 4: Applications of the Derivative143 Questions
Exam 5: Integration154 Questions
Exam 6: Applications of the Definite Integral113 Questions
Exam 7: Integration Techniques95 Questions
Exam 8: First-Order Differential Equations72 Questions
Exam 9: Infinite Series111 Questions
Exam 10: Parametric Equations and Polar Coordinates129 Questions
Exam 11: Vectors and the Geometry of Space107 Questions
Exam 12: Vector-Valued Functions103 Questions
Exam 13: Functions of Several Variables and Partial Differentiation112 Questions
Exam 14: Multiple Integrals92 Questions
Exam 15: Vector Calculus67 Questions
Exam 16: Second Order Differential Equations38 Questions
Select questions type
Is the function 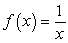 a probability density function on the interval
a probability density function on the interval 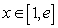 ?
?
(Multiple Choice)
4.9/5  (20)
(20)
Given the pdf for a particular exam ![Given the pdf for a particular exam , identify the cut-off for students scoring in the top 30%. [Hint: If the solution is difficult to obtain analytically, set up the expression to be solved and substitute the various choices below.]](https://storage.examlex.com/TB2342/11eaa948_cd0c_aa5e_84bc_2d1ab35c8e36_TB2342_11.jpg) , identify the cut-off for students scoring in the top 30%. [Hint: If the solution is difficult to obtain analytically, set up the expression to be solved and substitute the various choices below.]
, identify the cut-off for students scoring in the top 30%. [Hint: If the solution is difficult to obtain analytically, set up the expression to be solved and substitute the various choices below.]
(Multiple Choice)
4.9/5  (26)
(26)
Compute the volume of a solid formed by revolving the region bounded by 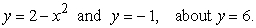
(Multiple Choice)
4.8/5  (39)
(39)
Use cylindrical shells to compute the volume of the solid formed by revolving the region bounded by 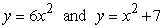 about x = 13.
about x = 13.
(Multiple Choice)
4.9/5  (32)
(32)
Find the length of the line segment described by the function of 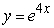 from
from 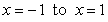 . Round your answer to three decimal places.
. Round your answer to three decimal places.
(Multiple Choice)
4.8/5  (27)
(27)
A football (American) has a circular cross section with radius approximately  for
for 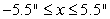 . Compute the volume of the football.
. Compute the volume of the football.
(Multiple Choice)
4.9/5  (25)
(25)
What is the minimum speed a ball must be thrown upward if it is to reach a height of 11 feet?
(Multiple Choice)
4.8/5  (31)
(31)
Find the value of c which will make the function 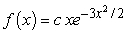 a pdf on the interval
a pdf on the interval 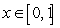 .
.
(Multiple Choice)
4.8/5  (32)
(32)
Identify the graph and the area bounded by the curves 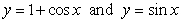 on the interval
on the interval  .
.
(Multiple Choice)
4.9/5  (40)
(40)
Set up the integral for the surface area of the surface of revolution and approximate the integral with a numerical method. Round answers to two decimal places. 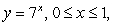 revolved about the x-axis
revolved about the x-axis
(Multiple Choice)
4.7/5  (35)
(35)
In a baseball game a base-runner can attempt to advance when a fielder catches a hit ball before it reaches the ground, so long as the runner doesn't leave base before the fielder catches the ball. The fielder then attempts to throw the ball to the base ahead of the runner. If a base-runner can run from 3rd base to home plate in 4.0 sec, how fast must the centerfielder's throw leave his hand if he makes the catch then immediately throws the ball 350 ft from home plate? Assume the base-runner leaves his base at the same instant the centerfielder throws the ball, and that the ball reaches the catcher standing over home plate at the same height from which it was thrown and at the same time the runner would reach the plate. [Hint: This is basically a problem of determining how much initial vertical velocity the ball needs to stay in the air long enough to reach the plate.]
(Multiple Choice)
4.8/5  (36)
(36)
A 2-person tent has a rectangular base 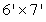 and rises to a single point 5' up. Compute the volume of the tent.
and rises to a single point 5' up. Compute the volume of the tent. 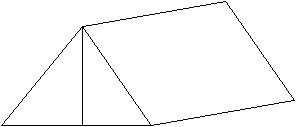 6 ft 7 ft
6 ft 7 ft
(Multiple Choice)
4.8/5  (31)
(31)
Identify the graph and area of the region bounded by the curves 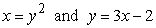 . Use only one integral. The figures below are not necessarily to scale.
. Use only one integral. The figures below are not necessarily to scale.
(Multiple Choice)
4.7/5  (31)
(31)
Compute the volume of the solid formed by revolving the region bounded by 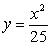 and
and 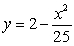 about the x-axis.
about the x-axis.
(Multiple Choice)
4.7/5  (27)
(27)
Find the area between the following curves on the given interval. 
(Multiple Choice)
4.9/5  (36)
(36)
A person 6' tall wants to buy a jump rope. If , when the rope is at its lowest point, his hands will be 2' apart and 3' above the ground, and if the rope will take on the shape of a parabola just barely hitting the ground, how long must the rope be? [Consider the form of the rope to be described by the equation ![A person 6' tall wants to buy a jump rope. If , when the rope is at its lowest point, his hands will be 2' apart and 3' above the ground, and if the rope will take on the shape of a parabola just barely hitting the ground, how long must the rope be? [Consider the form of the rope to be described by the equation , with the origin being a spot on the ground underneath the jumper.]](https://storage.examlex.com/TB2342/11eaa948_cd07_0488_84bc_ab64d22e93c9_TB2342_11.jpg) , with the origin being a spot on the ground underneath the jumper.]
, with the origin being a spot on the ground underneath the jumper.]
(Multiple Choice)
4.9/5  (30)
(30)
Probability density functions can be weighted so that different properties of the distribution can be enhanced or diminished. For instance, if a set of test scores is to be used in comparing the highest performers in a class, one might want to use a pdf, 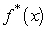 , in which the probability of finding a certain score, f(x), is multiplied by that score:
, in which the probability of finding a certain score, f(x), is multiplied by that score: 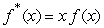 . A mean found using the weighted pdf might be called a score-weighted mean. Find the score-weighted mean for an exam for which the probability of finding a score
. A mean found using the weighted pdf might be called a score-weighted mean. Find the score-weighted mean for an exam for which the probability of finding a score 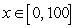 is given by the function
is given by the function 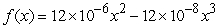 . You will need to construct the weighted pdf then use it to find the mean score.
. You will need to construct the weighted pdf then use it to find the mean score.
(Multiple Choice)
4.8/5  (31)
(31)
A projectile passes overhead, and you attempt to shoot it down with a cannon. When it passes overhead it is traveling horizontally at 100 m/s at an elevation of 1500 m. Your cannon launches shells at 200 m/s, and in this instance you fire just as the projectile passes overhead and at an angle of 60o. In this scenario the horizontal velocities of the two projectiles are the same. At what elevation will the cannon shell and the other projectile collide?
(Multiple Choice)
4.7/5  (40)
(40)
A given sample of a particular polymer will have molecules with a range of molecular weights, MW. If the molecular weight pdf is 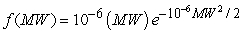 , what fraction of the molecules will have a molecular weight less than 500?
, what fraction of the molecules will have a molecular weight less than 500?
(Multiple Choice)
4.8/5  (33)
(33)
Show that the volume of a solid formed by revolving the region bounded by x = y2 and x = r about x = r is 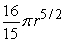 .
.
(Essay)
4.8/5  (44)
(44)
Showing 21 - 40 of 113
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)