Exam 10: B: Inference From Small Samples
Exam 1: Describing Data With Graphs134 Questions
Exam 2: Describing Data With Numerical Measures235 Questions
Exam 3: Describing Bivariate Data57 Questions
Exam 4: A: probability and Probability Distributions107 Questions
Exam 4: B: probability and Probability Distributions157 Questions
Exam 5: Several Useful Discrete Distributions166 Questions
Exam 6: The Normal Probability Distribution235 Questions
Exam 7: Sampling Distributions231 Questions
Exam 8: Large-Sample Estimation187 Questions
Exam 9: A: large-Sample Tests of Hypotheses154 Questions
Exam 9: B: large-Sample Tests of Hypotheses106 Questions
Exam 10: A: Inference From Small Samples192 Questions
Exam 10: B: Inference From Small Samples124 Questions
Exam 11: A: The Analysis of Variance136 Questions
Exam 11: B: The Analysis of Variance137 Questions
Exam 12: A: linear Regression and Correlation131 Questions
Exam 12: B: linear Regression and Correlation171 Questions
Exam 13: Multiple Regression Analysis232 Questions
Exam 14: Analysis of Categorical Data158 Questions
Exam 15: A:nonparametric Statistics139 Questions
Exam 15: B:nonparametric Statistics95 Questions
Select questions type
Snowmobile Speeds Narrative
A customer was interested in comparing the top speed (in kilometres per hour) of two models of snowmobiles. The customer selected two independent random samples of the snowmobiles and calculated the following summary information:  Assume the distribution of top speeds is approximately normal.
-Refer to Snowmobile Speeds Narrative. State the appropriate null and alternative hypotheses to test whether there is a significant difference between the two models of snowmobiles in average top speed.
Assume the distribution of top speeds is approximately normal.
-Refer to Snowmobile Speeds Narrative. State the appropriate null and alternative hypotheses to test whether there is a significant difference between the two models of snowmobiles in average top speed.
(Essay)
4.9/5  (36)
(36)
Snowmobile Speeds Narrative
A customer was interested in comparing the top speed (in kilometres per hour) of two models of snowmobiles. The customer selected two independent random samples of the snowmobiles and calculated the following summary information:  Assume the distribution of top speeds is approximately normal.
-Refer to Snowmobile Speeds Narrative. Calculate the value of the test statistic.
Assume the distribution of top speeds is approximately normal.
-Refer to Snowmobile Speeds Narrative. Calculate the value of the test statistic.
(Essay)
4.8/5  (35)
(35)
Cigarette Tar Content Narrative
Ten measurements of the tar content of a certain brand of cigarette are 13.5, 14.0, 13.9, 14.2, 15.1, 14.6, 13.8, 14.0, 14.1, and 14.7 in milligrams per cigarette. Assume these measurements were taken from a population with a normal distribution.
-Refer to Cigarette Tar Content Narrative. Construct a 90% confidence interval for the mean tar content of any cigarette of this brand.
(Essay)
4.8/5  (30)
(30)
Temperature Average Narrative
The average low temperature for Victoria, B.C. in September is 12°C. The average low temperature for each of eight randomly selected years is 11.0, 12.4, 11.8, 10.9, 11.4, 12.2, 10.8, and 12.2. Assume the September low temperature distribution is normal. Do the sample data suggest the average low temperature is lower than 12°C?
-Refer to Temperature Average Narrative. Compute the test statistic for the hypotheses in the previous question.
(Essay)
4.9/5  (39)
(39)
Vertical Blinds Installation Narrative
A drapery store manager was interested in determining whether a new employee can install vertical blinds faster than an employee who has been with the company for two years. The manager takes independent samples of ten vertical blind installations of each of the two employees and computes the following information.  -Refer to Vertical Blinds Installation Narrative. Calculate the value of the test statistic.
-Refer to Vertical Blinds Installation Narrative. Calculate the value of the test statistic.
(Essay)
4.9/5  (40)
(40)
Studying Time Narrative
A faculty advisor was interested in determining whether there is a difference between male and female students in the amount of time (in hours) spent studying on weeknights (Monday through Thursday). The advisor selected a random sample of 12 female students and a second random, but independent, sample of 10 male students and asked each student to indicate the average amount of time spent studying on a weeknight. The following summary statistics are obtained. 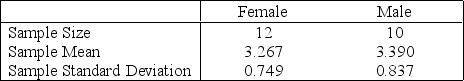 -Refer to Studying Time Narrative. State the null and alternative hypotheses for the advisor.
-Refer to Studying Time Narrative. State the null and alternative hypotheses for the advisor.
(Essay)
4.8/5  (43)
(43)
Grocery Receipts Narrative
A simple random sample of 100 grocery receipts was drawn from a normal population. The mean and standard deviation of the sample were $120 and $25, respectively.
-Refer to Grocery Receipts Narrative. Explain how to use the confidence interval to test the hypotheses at 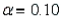 .
.
(Essay)
4.8/5  (34)
(34)
Assume that the population distributions of ages (in years) of students at two different universities in Ontario are normal with equal variances. Two random samples, drawn independently from the populations, showed the following statistics:  = 10,
= 10,  = 25,
= 25, 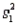 = 4;
= 4; 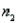 = 9,
= 9, 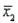 = 24, and
= 24, and  = 9. Construct and interpret a 99% confidence interval for the true difference in average ages of students at each university.
= 9. Construct and interpret a 99% confidence interval for the true difference in average ages of students at each university.
(Essay)
4.8/5  (32)
(32)
Childcare Costs Narrative
The public relations officer for a particular city claims the average monthly cost for childcare outside the home for a single child is $600. A potential resident is interested in whether the claim is correct. She obtains a random sample of 14 records and computes the average monthly cost of this type of childcare to be $589 with a standard deviation of $40.
-Refer to Childcare Costs Narrative. Perform the appropriate test of hypothesis for the potential resident using 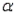 = 0.01.
= 0.01.
(Essay)
5.0/5  (33)
(33)
Bottling Productivity Narrative
Five soft drink bottling companies have agreed to implement a time management program in hopes of increasing productivity (measured in cases of soft drinks bottled per hour). The number of cases of soft drinks bottled per hour before and after the implementation of the program are listed below:
Company  -Refer to Bottling Productivity Narrative. Set up the appropriate rejection region for the hypotheses above, assuming = 0.05.
-Refer to Bottling Productivity Narrative. Set up the appropriate rejection region for the hypotheses above, assuming = 0.05.
(Essay)
4.7/5  (31)
(31)
Running Shoes Narrative
A new runner has decided to purchase a new pair of running shoes. He has narrowed his choices to two brands, each of which would be appropriate for his use. His concern is whether there is a significant difference in the average wear between the two brands of shoes. He enlists a random sample of six veteran runners to test the shoes. Each runner wore each brand of shoe until it wore out. The following data were recorded, representing the number of weeks each runner used each pair of shoes:
Runner  -Refer to Running Shoes Narrative. The new runner used a paired-difference t test for the analysis. Is this method appropriate? Justify your answer.
-Refer to Running Shoes Narrative. The new runner used a paired-difference t test for the analysis. Is this method appropriate? Justify your answer.
(Essay)
4.8/5  (33)
(33)
Studying Time Narrative
A faculty advisor was interested in determining whether there is a difference between male and female students in the amount of time (in hours) spent studying on weeknights (Monday through Thursday). The advisor selected a random sample of 12 female students and a second random, but independent, sample of 10 male students and asked each student to indicate the average amount of time spent studying on a weeknight. The following summary statistics are obtained.  -Refer to Studying Time Narrative. Use
-Refer to Studying Time Narrative. Use 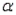 = 0.05 to test the hypothesis that the two population variances are equal.
= 0.05 to test the hypothesis that the two population variances are equal.
(Essay)
4.9/5  (36)
(36)
Preservatives Narrative
A food processing plant wants to compare two preservatives for their effects on retarding spoilage. Suppose 16 cuts of fresh meat are treated with preservative A and 16 are treated with preservative B. The number of hours until spoilage begins is recorded for each of the 32 cuts of meat. The results are summarized in the table below. 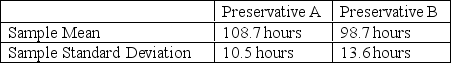 -Refer to Preservatives Narrative. State the null and alternative hypotheses to determine if the average number of hours until spoilage begins differs for the preservatives A and B.
-Refer to Preservatives Narrative. State the null and alternative hypotheses to determine if the average number of hours until spoilage begins differs for the preservatives A and B.
(Essay)
4.9/5  (41)
(41)
A psychologist is trying to determine how many hours the average person sleeps each night. He takes a random sample of 25 individuals and asks each person how many hours he or she slept the previous night. The sum of the observations and the sum of the squared observations are 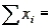 192.5 and
192.5 and 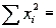 1531.7. Estimate with 99% confidence the mean number of hours of sleep.
1531.7. Estimate with 99% confidence the mean number of hours of sleep.
(Essay)
4.8/5  (30)
(30)
Coffee Breaks Narrative
Do government employees take longer coffee breaks than private sector workers? That is a question that interested a management consultant. To examine the issue, he took a random sample of ten government employees and another random sample of ten private sector workers and measured the amount of time (in minutes) they spent in coffee breaks during the day. The results are listed below. 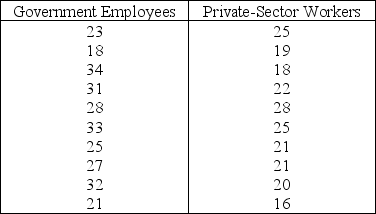 -Refer to Coffee Breaks Narrative. Estimate with 95% confidence the difference between the two groups in coffee break mean time.
-Refer to Coffee Breaks Narrative. Estimate with 95% confidence the difference between the two groups in coffee break mean time.
(Essay)
4.8/5  (32)
(32)
Vertical Blinds Installation Narrative
A drapery store manager was interested in determining whether a new employee can install vertical blinds faster than an employee who has been with the company for two years. The manager takes independent samples of ten vertical blind installations of each of the two employees and computes the following information.  -Refer to Vertical Blinds Installation Narrative. State the appropriate null and alternative hypotheses to test whether the new employee installs vertical blinds faster, on the average, than the veteran employee.
-Refer to Vertical Blinds Installation Narrative. State the appropriate null and alternative hypotheses to test whether the new employee installs vertical blinds faster, on the average, than the veteran employee.
(Essay)
4.8/5  (41)
(41)
Quiz Scores Narrative
Two independent random samples of sizes 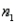 = 4 and
= 4 and 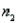 = 5 are selected from two normal populations. The data below represent the scores in a 20-point quiz.
= 5 are selected from two normal populations. The data below represent the scores in a 20-point quiz. 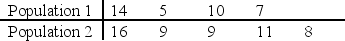 -Refer to Quiz Scores Narrative. Find a 90% confidence interval for (
-Refer to Quiz Scores Narrative. Find a 90% confidence interval for (  ), the difference between the two population means.
), the difference between the two population means.
(Essay)
4.9/5  (27)
(27)
Coffee Breaks Narrative
Do government employees take longer coffee breaks than private sector workers? That is a question that interested a management consultant. To examine the issue, he took a random sample of ten government employees and another random sample of ten private sector workers and measured the amount of time (in minutes) they spent in coffee breaks during the day. The results are listed below.  -Refer to Coffee Breaks Narrative. Explain what the interval estimate tells you.
-Refer to Coffee Breaks Narrative. Explain what the interval estimate tells you.
(Essay)
4.9/5  (41)
(41)
Average Fuel Consumption
The average fuel consumption of a 4-wheel drive truck is 12.9 L/100 km. The average fuel consumption for seven randomly selected trucks is 13.5, 13.0, 12.6, 12.2, 12.8, 12.9, and 13.1. Assume the fuel consumption distribution is normal. The researcher wishes to know if the sample data suggest that the average fuel consumption is different from 12.9 L/100 km.
-Please refer to the Average Fuel Consumption paragraph. State the appropriate hypotheses.
(Essay)
4.8/5  (28)
(28)
Cigarette Tar Content Narrative
Ten measurements of the tar content of a certain brand of cigarette are 13.5, 14.0, 13.9, 14.2, 15.1, 14.6, 13.8, 14.0, 14.1, and 14.7 in milligrams per cigarette. Assume these measurements were taken from a population with a normal distribution.
-One study revealed a child under the age of 10 watches television 4.5 hours per day. A group of families from a certain community would like to believe that their children watch less television than the national average. A random sample of 14 children from the community yielded a mean of 4.1 hours per day with a standard deviation of 1.2. Test the appropriate hypotheses at the 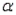 = 0.01 level. Assume the viewing time is normally distributed and interpret your results.
= 0.01 level. Assume the viewing time is normally distributed and interpret your results.
(Essay)
4.7/5  (37)
(37)
Showing 61 - 80 of 124
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)