Question 1

If South Korea's steady-state GDP per worker is higher than that of the Philippines, you might conclude that ________, ceteris paribus.

Accepted Answer

A)  the investment rate in South Korea is higher than in the Philippines 
B)  South Korea is less productive than the Philippines 
C)  the depreciation rate in South Korea is higher than in the Philippines 
D)  South Korea has a larger population 
E)  None of these answers is correct 
A)  the investment rate in South Korea is higher than in the Philippines 
B)  South Korea is less productive than the Philippines 
C)  the depreciation rate in South Korea is higher than in the Philippines 
D)  South Korea has a larger population 
E)  None of these answers is correct A

Question 2

You are asked to make comparisons of two pairs of countries. The first pair is the Latin American countries of Chile and Argentina; the second pair is France and Germany. You are given the following information: the average saving rate in Argentina is 23.3 percent, in Chile it is 28.7 percent, in France it is 21.1 percent, and in Germany it is 20.8 percent. Assuming the countries are identical in every other way, which country would the Solow model predict to have the higher per capita real GDP? However, you find out the steady-state real per capita GDP in each of the countries is $13,300 in Argentina, $12,500 in Chile, $31,300 in France, and $34,000 in Germany. What is the primary factor that the simple Solow model uses to describe these differences? Give an example.

Accepted Answer

It is easiest to do mathematically. Using equation 5.12, p. 115, we can make the comparison, comparing countries 1 and 2.
Because depreciation rates are identical: 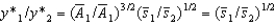  , because  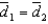 and 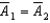  . From this it is clear that the model hypothesizes   .
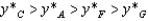However, the data shows that in fact the per capita RGDP is in Germany. The Solow model uses total factor productivity to explain differences in RPCGDP. The combination of a high TFP with the saving rate implies-according to the Solow model-a higher steady-state level of output. On the other end of the scale, the low saving rate in Argentina, possibly coupled with low TFP, means a relatively low steady-state output. Examples of factors that contribute to TFP include human capital, health, institutions, high levels of peace, and so on.

Question 3

Refer to the following figure when answering the following questions.
Figure 5.1: Solow Diagram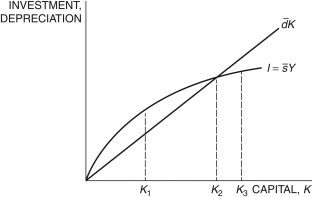  
-In Figure 5.1, if the economy begins with the initial capital stock at K1, the capital stock will ________ and the economy will ________.

Accepted Answer

A)  decrease; grow 
B)  increase; grow 
C)  stay constant; shrink 
D)  decrease; shrink 
E)  stay constant; grow 
A)  decrease; grow 
B)  increase; grow 
C)  stay constant; shrink 
D)  decrease; shrink 
E)  stay constant; grow B

Question 4

The key insight in the Solow model is that:

Accepted Answer

A)  saving rates are determined in a particular manner. 
B)  savings have no impact on economic growth. 
C)  capital depreciation enhances economic growth. 
D)  the relationship between capital and output is static. 
E)  capital accumulation contributes to economic growth. 
A)  saving rates are determined in a particular manner. 
B)  savings have no impact on economic growth. 
C)  capital depreciation enhances economic growth. 
D)  the relationship between capital and output is static. 
E)  capital accumulation contributes to economic growth.

Question 5

A decline in the saving rate causes the steady-state level of output:

Accepted Answer

A)  and capital to rise. 
B)  to rise and capital to fall. 
C)  and capital to fall. 
D)  and capital to remain constant. 
E)  to rise and capital to remain constant. 
A)  and capital to rise. 
B)  to rise and capital to fall. 
C)  and capital to fall. 
D)  and capital to remain constant. 
E)  to rise and capital to remain constant.

Question 6

Refer to the following figure when answering the following questions.
Figure 5.1: Solow Diagram  
-In Figure 5.1, the capital stock at K1 is not the steady state because:

Accepted Answer

A)  the saving rate is too low. 
B)  the saving rate is too high. 
C)  the depreciation rate is too low. 
D)  gross investment is higher than capital depreciation. 
E)  gross investment is lower than capital depreciation. 
A)  the saving rate is too low. 
B)  the saving rate is too high. 
C)  the depreciation rate is too low. 
D)  gross investment is higher than capital depreciation. 
E)  gross investment is lower than capital depreciation.

Question 7

According to the Solow model, in the steady state, countries with high saving rates should have a:

Accepted Answer

A)  low labor-output ratio. 
B)  low capital-output ratio. 
C)  high capital-output ratio. 
D)  high depreciation rate. 
E)  high A. 
A)  low labor-output ratio. 
B)  low capital-output ratio. 
C)  high capital-output ratio. 
D)  high depreciation rate. 
E)  high A.

Question 8

In the Solow model, if capital is in the steady state, output:

Accepted Answer

A)  will continue to grow. 
B)  is also in the steady state. 
C)  will continue to grow, but its rate of growth will slow down. 
D)  will decline, but its rate of growth will be positive. 
E)  will begin to contract. 
A)  will continue to grow. 
B)  is also in the steady state. 
C)  will continue to grow, but its rate of growth will slow down. 
D)  will decline, but its rate of growth will be positive. 
E)  will begin to contract.

Question 9

Refer to the following figure when answering the following questions.
Figure 5.7: Solow Diagram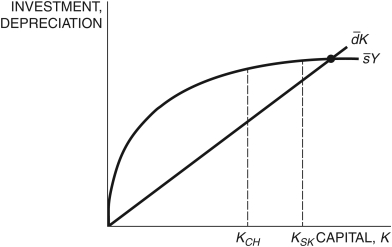  
-Consider Figure 5.7. If KSK is the current capital stock in South Korea and KCH is the current capital stock in China, according to the principle of transition dynamics:

Accepted Answer

A)  South Korea will be richer than China, forever. 
B)  South Korea will initially grow slower, but incomes in China and South Korea will converge. 
C)  China and South Korea have different steady states. 
D)  South Korea will initially grow faster, but incomes in China and South Korea will converge. 
E)  China will initially grow slower, but incomes in China and South Korea will converge. 
A)  South Korea will be richer than China, forever. 
B)  South Korea will initially grow slower, but incomes in China and South Korea will converge. 
C)  China and South Korea have different steady states. 
D)  South Korea will initially grow faster, but incomes in China and South Korea will converge. 
E)  China will initially grow slower, but incomes in China and South Korea will converge.

Question 10

If South Korea's steady-state GDP per worker is higher than that in the Philippines, you might conclude that the saving/depreciation rate in South Korea is higher/lower than in the Philippines.

Accepted Answer

A) True 
 B)False

Question 11

Assume two economies are identical in every way except that one has a higher saving rate. According to the Solow growth model, in the steady state, the country with the higher saving rate will have ________ level of total output and ________ rate of growth of output than/as the country with the lower saving rate.

Accepted Answer

A)  a higher; a higher 
B)  a higher; the same 
C)  a lower; a higher 
D)  a higher; a lower 
E)  the same; the same 
A)  a higher; a higher 
B)  a higher; the same 
C)  a lower; a higher 
D)  a higher; a lower 
E)  the same; the same

Question 12

Refer to the following figure when answering the following questions.
Figure 5.4: Solow Diagram

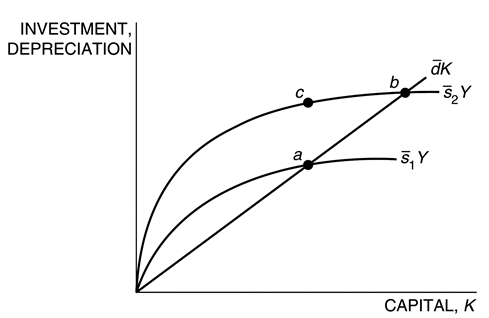-Consider the Solow model exhibited in Figure 5.4. Which of the following is/are true?
i. For any single country, the movement from point a to b is due to an increase in the saving Rate, s1  $\gt$ s2.
ii. For any single country, the movement from point c to b is due to an increase in capital Stock for the saving rate, s2.
iii. If s1 and s2 stand for the saving rates in Countries 1 and 2, respectively, Country 2 has a Lower saving rate.

Accepted Answer

A)  i 
B)  ii 
C)  iii 
D)  i and ii 
E)  i, ii, and iii 
A)  i 
B)  ii 
C)  iii 
D)  i and ii 
E)  i, ii, and iii

Question 13

The steady-state level of output per worker in the Solow model, with the production function   , is given by:

Accepted Answer

A)    . 
B)    . 
C)    . 
D)    . 
E)    . 
A)    . 
B)    . 
C)    . 
D)    . 
E)    .

Question 14

Over the past 30 years, ________ has averaged a saving rate of ________ percent.

Accepted Answer

A)  Japan; 15 
B)  Russia; 5 
C)  the United States; 25 
D)  South Korea; 45 
E)  China; 12.5 
A)  Japan; 15 
B)  Russia; 5 
C)  the United States; 25 
D)  South Korea; 45 
E)  China; 12.5

Question 15

In the Solow model, defining   
as the saving rate and Yt as output, consumption is given by   
.

Accepted Answer

A) True 
 B)False

Question 16

In the Solow model, if net investment is positive:

Accepted Answer

A)  capital accumulation is zero. 
B)  capital accumulation is negative. 
C)  capital accumulation is positive. 
D)  savings are negative. 
E)  Not enough information is given. 
A)  capital accumulation is zero. 
B)  capital accumulation is negative. 
C)  capital accumulation is positive. 
D)  savings are negative. 
E)  Not enough information is given.

Question 17

In the standard production model's production function, the productivity parameter enters the equation with an exponent of one, while in the Solow model's equation for the steady-state stock of capital it is greater than one because:

Accepted Answer

A)  the endogenous level of the capital stock itself depends on productivity. 
B)  there is no productivity parameter in the production function model. 
C)  the productivity measure is zero in the production function model. 
D)  the productivity measure is negative in the Solow model. 
E)  the exogenous level of the capital stock itself depends on productivity. 
A)  the endogenous level of the capital stock itself depends on productivity. 
B)  there is no productivity parameter in the production function model. 
C)  the productivity measure is zero in the production function model. 
D)  the productivity measure is negative in the Solow model. 
E)  the exogenous level of the capital stock itself depends on productivity.

Question 18

Immediately following the increase in the saving rate, output grows rapidly. As the economy approaches its new steady state, the growth rate:

Accepted Answer

A)  gradually increases. 
B)  gradually declines. 
C)  is constant. 
D)  is negative. 
E)  None of these answers is correct. 
A)  gradually increases. 
B)  gradually declines. 
C)  is constant. 
D)  is negative. 
E)  None of these answers is correct.

Question 19

On average, if both rich and poor countries grow at the same rate, this suggests that:

Accepted Answer

A)  most countries are contracting. 
B)  most countries still are growing. 
C)  no countries have reached their steady states. 
D)  most countries have reached their steady states. 
E)  most countries are unproductive. 
A)  most countries are contracting. 
B)  most countries still are growing. 
C)  no countries have reached their steady states. 
D)  most countries have reached their steady states. 
E)  most countries are unproductive.

Question 20

In the Solow model, if a country's saving rate increases, the country:

Accepted Answer

A)  moves from a relatively low steady state to one that is lower. 
B)  moves from a relatively low steady state to one that is higher. 
C)  moves from a relatively high steady state to one that is lower. 
D)  stays at a constant high steady state. 
E)  stays at a constant low steady state. 
A)  moves from a relatively low steady state to one that is lower. 
B)  moves from a relatively low steady state to one that is higher. 
C)  moves from a relatively high steady state to one that is lower. 
D)  stays at a constant high steady state. 
E)  stays at a constant low steady state.

If South Korea's steady-state GDP per worker is higher than that of the Philippines, you might conclude that ________, ceteris paribus.

Refer to the following figure when answering the following questions. Figure 5.1: Solow Diagram -In Figure 5.1, if the economy begins with the initial capital stock at K₁, the capital stock will and the economy will .

The key insight in the Solow model is that:

A decline in the saving rate causes the steady-state level of output:

Refer to the following figure when answering the following questions. Figure 5.1: Solow Diagram -In Figure 5.1, the capital stock at K₁ is not the steady state because:

According to the Solow model, in the steady state, countries with high saving rates should have a:

In the Solow model, if capital is in the steady state, output:

Refer to the following figure when answering the following questions. Figure 5.7: Solow Diagram -Consider Figure 5.7. If K_SK is the current capital stock in South Korea and K_CH is the current capital stock in China, according to the principle of transition dynamics:

If South Korea's steady-state GDP per worker is higher than that in the Philippines, you might conclude that the saving/depreciation rate in South Korea is higher/lower than in the Philippines.

Assume two economies are identical in every way except that one has a higher saving rate. According to the Solow growth model, in the steady state, the country with the higher saving rate will have level of total output and rate of growth of output than/as the country with the lower saving rate.

The steady-state level of output per worker in the Solow model, with the production function , is given by:

Over the past 30 years, has averaged a saving rate of percent.

In the Solow model, defining 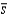as the saving rate and Y_t as output, consumption is given by .

In the Solow model, if net investment is positive:

In the standard production model's production function, the productivity parameter enters the equation with an exponent of one, while in the Solow model's equation for the steady-state stock of capital it is greater than one because:

Immediately following the increase in the saving rate, output grows rapidly. As the economy approaches its new steady state, the growth rate:

On average, if both rich and poor countries grow at the same rate, this suggests that:

In the Solow model, if a country's saving rate increases, the country:

Introduction to Macroeconomics

Measuring the Macroeconomy

An Overview of Long-Run Economic Growth

A Model of Production

Growth and Ideas

The Labor Market, Wages, and Unemployment

Inflation

An Introduction to the Short Run

The Great Recession: a First Look

The Is Curve

Monetary Policy and the Phillips Curve

Stabilization Policy and the Asad Framework

The Great Recession and the Short-Run Model

Dsge Models: the Frontier of Business Cycle Research

Consumption

Investment

The Government and the Macroeconomy

International Trade

Exchange Rates and International Finance

Parting Thoughts

Filters

Exam 5: The Solow Growth Model

If South Korea's steady-state GDP per worker is higher than that of the Philippines, you might conclude that ________, ceteris paribus.

Refer to the following figure when answering the following questions. Figure 5.1: Solow Diagram -In Figure 5.1, if the economy begins with the initial capital stock at K1, the capital stock will ________ and the economy will ________.

The key insight in the Solow model is that:

A decline in the saving rate causes the steady-state level of output:

Refer to the following figure when answering the following questions. Figure 5.1: Solow Diagram -In Figure 5.1, the capital stock at K1 is not the steady state because:

According to the Solow model, in the steady state, countries with high saving rates should have a:

In the Solow model, if capital is in the steady state, output:

Refer to the following figure when answering the following questions. Figure 5.7: Solow Diagram -Consider Figure 5.7. If KSK is the current capital stock in South Korea and KCH is the current capital stock in China, according to the principle of transition dynamics:

If South Korea's steady-state GDP per worker is higher than that in the Philippines, you might conclude that the saving/depreciation rate in South Korea is higher/lower than in the Philippines.

The steady-state level of output per worker in the Solow model, with the production function , is given by:

Over the past 30 years, ________ has averaged a saving rate of ________ percent.

In the Solow model, defining as the saving rate and Yt as output, consumption is given by .

In the Solow model, if net investment is positive:

In the standard production model's production function, the productivity parameter enters the equation with an exponent of one, while in the Solow model's equation for the steady-state stock of capital it is greater than one because:

Immediately following the increase in the saving rate, output grows rapidly. As the economy approaches its new steady state, the growth rate:

On average, if both rich and poor countries grow at the same rate, this suggests that:

In the Solow model, if a country's saving rate increases, the country:

Introduction to Macroeconomics

Measuring the Macroeconomy

An Overview of Long-Run Economic Growth

A Model of Production

Growth and Ideas

The Labor Market, Wages, and Unemployment

Inflation

An Introduction to the Short Run

The Great Recession: a First Look

The Is Curve

Monetary Policy and the Phillips Curve

Stabilization Policy and the Asad Framework

The Great Recession and the Short-Run Model

Dsge Models: the Frontier of Business Cycle Research

Consumption

Investment

The Government and the Macroeconomy

International Trade

Exchange Rates and International Finance

Parting Thoughts

Filters

Refer to the following figure when answering the following questions. Figure 5.1: Solow Diagram -In Figure 5.1, if the economy begins with the initial capital stock at K₁, the capital stock will and the economy will .

Refer to the following figure when answering the following questions. Figure 5.1: Solow Diagram -In Figure 5.1, the capital stock at K₁ is not the steady state because:

Refer to the following figure when answering the following questions. Figure 5.7: Solow Diagram -Consider Figure 5.7. If K_SK is the current capital stock in South Korea and K_CH is the current capital stock in China, according to the principle of transition dynamics:

Over the past 30 years, has averaged a saving rate of percent.

In the Solow model, defining as the saving rate and Y_t as output, consumption is given by .