Exam 13: Statistical Process Control
Exam 1: Introduction126 Questions
Exam 2: Total Quality In Organizations105 Questions
Exam 3: Philosophies And Frameworks130 Questions
Exam 4: Strategic Focus For Performance Excellence104 Questions
Exam 5: Focusing On Customers97 Questions
Exam 6: High Performance Workforce Management109 Questions
Exam 7: Process Management98 Questions
Exam 8: Performance Measurement And Information Management129 Questions
Exam 9: Leading, building, and Sustaining Performance Excellence107 Questions
Exam 10: Statistical Thinking And Applications97 Questions
Exam 11: Six Sigma And Process Improvement78 Questions
Exam 12: Design For Quality And Product Excellence104 Questions
Exam 13: Statistical Process Control100 Questions
Select questions type
In the application of control charts,as the sample size is increased:
(Multiple Choice)
4.8/5  (36)
(36)
If the lower limit for a p-chart computes to a number less than zero,this indicates:
(Multiple Choice)
4.8/5  (35)
(35)
Assignable causes are more difficult to identify in unstable processes.
(True/False)
4.9/5  (36)
(36)
Construct a flow chart that illustrates the decision process for selecting the appropriate control chart(s)for monitoring a given process.
(Essay)
4.8/5  (32)
(32)
Collecting _____ data is usually easier than collecting _____ data because the assessment can usually be done more quickly by a simple inspection or count.
(Multiple Choice)
4.9/5  (29)
(29)
Which of the following is NOT an indication of an out-of-control process?
(Multiple Choice)
4.9/5  (31)
(31)
For variables data,the necessary sample size can be estimated by specifying the desired minimum shift in the process mean to be detected.
(True/False)
4.9/5  (32)
(32)
Control limits for variables and attribute data utilize the same mathematical formulas.
(True/False)
4.7/5  (32)
(32)
If all the points on a control chart are within the control limits,then the process is in statistical control.
(True/False)
4.8/5  (35)
(35)
Process capability indices cannot be computed from data in an 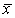 -chart.
-chart.
(True/False)
4.8/5  (32)
(32)
In the manufacture of vitamin capsules, the proportion of calcium in each capsule has a specification of 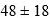 parts per million (ppm). A random sample of 100 capsules revealed a sample average calcium content of 44.5 ppm and a sample standard deviation of 3.2 ppm. Management uses
parts per million (ppm). A random sample of 100 capsules revealed a sample average calcium content of 44.5 ppm and a sample standard deviation of 3.2 ppm. Management uses  and R-charts to monitor the calcium amount using samples of size 4. The charts depict an in-control process.
The centerline of the R chart is 6.7 and the centerline of the
and R-charts to monitor the calcium amount using samples of size 4. The charts depict an in-control process.
The centerline of the R chart is 6.7 and the centerline of the 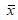 chart is 44.8.
a Compute
chart is 44.8.
a Compute 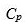 for this process using estimates of the process mean and standard
deviation derived from the random sample.
b. Compute
for this process using estimates of the process mean and standard
deviation derived from the random sample.
b. Compute 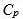 for this process using estimates of the process mean and standard
deviation derived from the control chart parameters.
c. Compute
for this process using estimates of the process mean and standard
deviation derived from the control chart parameters.
c. Compute  for this process using estimates of the process mean and standard
deviation derived from the random sample.
. Compute
for this process using estimates of the process mean and standard
deviation derived from the random sample.
. Compute 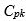 for this process using estimates of the process mean and standard
deviation derived from the control chart parameters
d. What do the differences between the values of
for this process using estimates of the process mean and standard
deviation derived from the control chart parameters
d. What do the differences between the values of  and
and 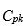 tell you?
tell you?
(Essay)
4.8/5  (36)
(36)
In service applications,we generally use the term _____ to describe a nonconformance.
(Multiple Choice)
4.8/5  (32)
(32)
The control chart most appropriate for data that represent the average number of packing slip errors per package shipped each day is the:
(Multiple Choice)
4.8/5  (40)
(40)
Histograms allow you to distinguish between common and special causes of variation.
(True/False)
4.9/5  (32)
(32)
Showing 41 - 60 of 100
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)