Exam 10: Topics In Analytic Geometry
Exam 1: Functions and Their Graphs513 Questions
Exam 2: Polynomial and Rational Functions456 Questions
Exam 3: Exponential and Logarithmic Functions266 Questions
Exam 4: Trigonometry384 Questions
Exam 5: Analytic Trigonometry265 Questions
Exam 6: Additional Topics In Trigonometery304 Questions
Exam 7: Systems Of Equations and Inequalities305 Questions
Exam 8: Matrices and Determinants283 Questions
Exam 9: Sequences Series and Probability405 Questions
Exam 10: Topics In Analytic Geometry556 Questions
Exam 11: Analytic Geometry In Three Dimensions256 Questions
Exam 12: Limits and An Introduction To Calculus259 Questions
Select questions type
Find the vertex and focus of the parabola from the given equation and select its graph.
(Multiple Choice)
4.9/5  (29)
(29)
Find the equation of the hyperbola with vertices (2, 0), (-2, 0) and focus (4, 0).
(Multiple Choice)
4.9/5  (36)
(36)
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
(Multiple Choice)
4.8/5  (38)
(38)
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
(Multiple Choice)
4.9/5  (31)
(31)
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
(Multiple Choice)
5.0/5  (40)
(40)
Using following result find a set of parametric equation of conic.
Hyperbola:
Hyperbola: vertices: ; foci:
(Multiple Choice)
4.9/5  (42)
(42)
Find the standard form of the equation of the parabola and determine the coordinates of the focus. 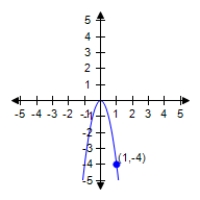
(Multiple Choice)
5.0/5  (31)
(31)
The revenue R (in dollars) generated by the sale of x units of a patio furniture set is given by .Approximate the number of sales that will maximize revenue.
(Multiple Choice)
4.9/5  (37)
(37)
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
(Multiple Choice)
4.7/5  (33)
(33)
The revenue (in dollars) generated by the sale of units of a patio furniture set is given by .Select the correct graph of the function.
(Multiple Choice)
4.8/5  (39)
(39)
Convert the rectangular equation to polar form.Assume a > 0, r > 0.
(Multiple Choice)
4.8/5  (33)
(33)
A point (a,b) = (r, ) shown in below graph in polar coordinates is given.Convert the point to rectangular coordinates. 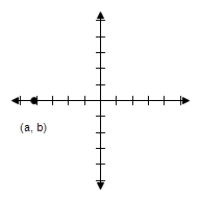
(Multiple Choice)
4.9/5  (40)
(40)
Showing 261 - 280 of 556
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)