Exam 10: Hypothesis Testing Using a Single Sample
Exam 1: The Role of Statistics and the Data Analysis Process17 Questions
Exam 2: Collecting Data Sensibly39 Questions
Exam 3: Graphical Methods for Describing Data25 Questions
Exam 4: Numerical Methods for Describing Data26 Questions
Exam 5: Summarizing Bivariate Data37 Questions
Exam 6: Probability34 Questions
Exam 7: Random Variables and Probability Distributions45 Questions
Exam 8: Sampling Variability and Sampling Distributions27 Questions
Exam 9: Estimation Using a Single Sample46 Questions
Exam 10: Hypothesis Testing Using a Single Sample37 Questions
Exam 11: Comparing Two Populations or Treatments42 Questions
Exam 12: The Analysis of Categorical Data and Goodness-Of-Fit Tests21 Questions
Exam 13: Simple Linear Regression and Correlation: Inferential Methods56 Questions
Exam 14: Multiple Regression Analysis38 Questions
Exam 15: Analysis of Variance40 Questions
Exam 16: Nonparametric Distribution-Free Statistical Methods15 Questions
Select questions type
Small P-values indicate that the observed sample is inconsistent with the null hypothesis.
Free
(True/False)
4.9/5  (32)
(32)
Correct Answer:
True
The university conducts a study designed to find out the proportion of students who have conversational fluency in two or more foreign languages. The previous study provided the result of 7%. The test is carried out using data from a random sample of n = 72. In this sample, the number of students who speak two or more foreign languages is 10. Complete an appropriate hypothesis test with α = 0.05 and make a conclusion using the output from the Shiny app "Exact Binomial Test for One Proportion". 
Free
(Multiple Choice)
4.7/5  (28)
(28)
Correct Answer:
D
For tests of hypotheses about μ, β decreases as the sample size increases if the level of significance stays the same.
Free
(True/False)
4.7/5  (35)
(35)
Correct Answer:
True
The level of significance of a test is the probability of making a type I error, given that the null hypothesis is true.
(True/False)
4.8/5  (32)
(32)
One of the early decisions that must be made when performing a hypothesis test is the choice of significance level. Briefly describe the considerations that go into making this decision.
(Essay)
4.8/5  (40)
(40)
Explain in your own words the distinction between a null and alternative hypothesis.
(Essay)
4.8/5  (33)
(33)
Psychologists have noted that people tend make classifications based on their "typicality." For example, a dog is more readily classified as a mammal than is a whale, because dogs are "more typical" of mammals than are whales. Previous large-scale studies have established the following for songbirds such as robins: when young children are shown a picture of a songbird and asked if it is a bird they respond "yes" in an average of 750 milliseconds. Do children regard chickens as representative of birds? If so, are they more representative or less representative of birds than are songbirds? Let μ represent the mean time it takes children to respond to a question about whether a chicken is a bird. The investigators wish to determine whether the classification time differs from the time needed to classify songbirds.
a) What is the appropriate null hypothesis?
b) What is the appropriate alternative hypothesis?
c) In your own words, distinguish between a Type I and a Type II error in this context.
(Essay)
4.8/5  (34)
(34)
Children as young as 2 years of age, upon seeing an object placed under a pillow in a familiar setting at home, will understand to look for it after an interval of time and be able to find it. Investigators believe this capability will be less pronounced in a laboratory situation, where the child is away from the familiar setting of home. Let π denote the proportion of 2-year-olds than have this understanding in the home situation, and suppose that π = .35. The investigators wish to determine whether the proportion that remembers is less when the child is away from home.
a) What is the appropriate null hypothesis?
b) What is the appropriate alternative hypothesis?
c) In your own words, distinguish between a Type I and a Type II error in this context.
(Essay)
4.9/5  (42)
(42)
City officials will conduct a study of the proportion of vehicle owners among the neighborhood residents. The study, which was held 5 years ago, provided the result of 50%. Suppose that a random sample of size n = 20 with the obtained proportion 0.45 is used to decide if the proportion has increased for the whole neighborhood. Calculate the P-value using the appropriate test to analyze these data.
(Multiple Choice)
4.9/5  (48)
(48)
A type II error is made by failing to reject a false null hypothesis.
(True/False)
4.9/5  (26)
(26)
Suppose that a study has been published linking arsenic, which is commonly found in tap water, to increased cancer rates. The study also stated that arsenic levels below 10 ppm could be considered harmless. Suppose that residents of Hacienda Heights, CA, are worried about the arsenic level in the local tap water.
a) What hypothesis should they test?
b) Describe a Type I and Type II error in this context.
(Essay)
4.8/5  (40)
(40)
The average wingspan of a crowned eagle is supposed to be 40 in. A random sample of 31 crowned eagles raised in captivity gave the following results:  = 39.5, s = 1.8. Do the data indicate that the wingspan of captive eagles is less than that of those that live in the wild? Use the significance level α = 0.05.
= 39.5, s = 1.8. Do the data indicate that the wingspan of captive eagles is less than that of those that live in the wild? Use the significance level α = 0.05.
(Multiple Choice)
4.7/5  (24)
(24)
All other things being equal, choosing a smaller value of α will increase the probability of making a type II error.
(True/False)
4.8/5  (39)
(39)
When performing tests of hypotheses, there are assumptions that must be met in order for the test to be appropriate. For the test of a hypothesis about a population proportion, describe how you would check the assumptions.
(Essay)
5.0/5  (22)
(22)
The average sleeping time required for teenagers who experience major physical exertion is about 8 hours. Do the members of the school sport teams sleep enough? Sleeping time for a random sample of 16 sport team members was obtained. 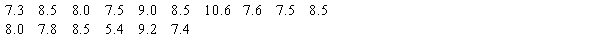 Which test is appropriate for this sample?
Which test is appropriate for this sample?
(Multiple Choice)
4.8/5  (34)
(34)
A cooler bag producing company claims that their coolers keep products cold for 12 hours. A test of hypotheses H0: µ = 12 versus Ha: µ < 12 is based on a sample of size n = 33. Suppose that σ is known to be 0.6 and a significance level of 0.05 will be used. Determine the power of the test and interpret the result when µ = 11.75.
(Multiple Choice)
4.8/5  (35)
(35)
Do the students in the campus spend less on food than an average American resident does? To answer this question, expenses of 25 students were calculated.  Food expenses of an average American person are $220. Interpret the output of the Shiny app "Randomization test for a population mean" using a significance level of 0.05.
Food expenses of an average American person are $220. Interpret the output of the Shiny app "Randomization test for a population mean" using a significance level of 0.05. 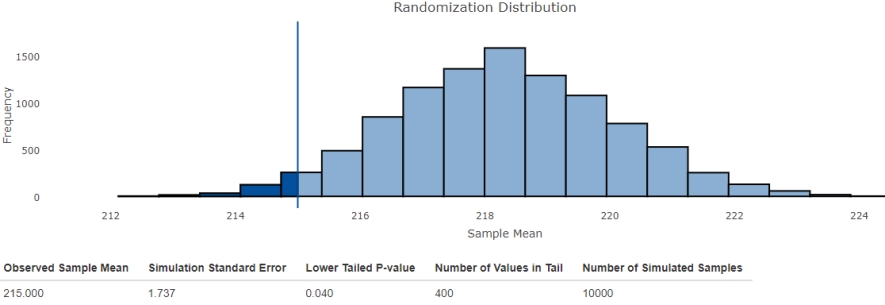
(Multiple Choice)
4.9/5  (28)
(28)
Showing 1 - 20 of 37
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)