Exam 8: Sampling Variability and Sampling Distributions
Exam 1: The Role of Statistics and the Data Analysis Process17 Questions
Exam 2: Collecting Data Sensibly39 Questions
Exam 3: Graphical Methods for Describing Data25 Questions
Exam 4: Numerical Methods for Describing Data26 Questions
Exam 5: Summarizing Bivariate Data37 Questions
Exam 6: Probability34 Questions
Exam 7: Random Variables and Probability Distributions45 Questions
Exam 8: Sampling Variability and Sampling Distributions27 Questions
Exam 9: Estimation Using a Single Sample46 Questions
Exam 10: Hypothesis Testing Using a Single Sample37 Questions
Exam 11: Comparing Two Populations or Treatments42 Questions
Exam 12: The Analysis of Categorical Data and Goodness-Of-Fit Tests21 Questions
Exam 13: Simple Linear Regression and Correlation: Inferential Methods56 Questions
Exam 14: Multiple Regression Analysis38 Questions
Exam 15: Analysis of Variance40 Questions
Exam 16: Nonparametric Distribution-Free Statistical Methods15 Questions
Select questions type
According to internal testing done by the HardCore Apple Company, the mean firmness of their apples, as measured on the Mohs hardness scale is 7.0, with a standard deviation of 0.3.
a) If the claim by Hardcore is true, what is the mean of the sampling distribution of  for samples of size n = 16?
b) If the claim by HardCore is true, what is the standard deviation of the sampling distribution of
for samples of size n = 16?
b) If the claim by HardCore is true, what is the standard deviation of the sampling distribution of  for samples of size n = 16?
c) If the distribution of HardCore firmness is approximately normal, and their claim about the mean firmness is true, what is the probability that the mean firmness of a random sample of size n = 16 will be less than 6.9?
for samples of size n = 16?
c) If the distribution of HardCore firmness is approximately normal, and their claim about the mean firmness is true, what is the probability that the mean firmness of a random sample of size n = 16 will be less than 6.9?
Free
(Essay)
4.9/5  (38)
(38)
Correct Answer:
a) 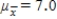
b) 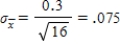
c) 
The closer π is to 0 or 1, the larger n must be in order for the distribution of p to be approximately normal.
Free
(True/False)
4.9/5  (28)
(28)
Correct Answer:
True
The first large-scale study of the human sex ratio involved over 6,000 families each having 12 children. (This was done in 19th Century Germany--large families were more common then and there.) 52% of the children they observed were boys. Suppose that 21st Century researchers wish to replicate this observational study. In order to see if this proportion of boys might have changed in the intervening years, suppose the researchers track down 50 families with 12 children. From these 600 children, a random sample of 50 children is taken. 30 of the 50 children were boys (p = 0.6.)
a) Show that it is reasonable to approximate the sampling distribution of p using a normal distribution.
b) If the modern true population proportion of newborn boys is π = 0.52, what is the probability of observing a sample proportion of at least p = 0.6?
Free
(Essay)
4.9/5  (29)
(29)
Correct Answer:
a) np = 50(.52) = 26 ≥ 10 and n(1 − p) = 50(1 − .52) = 24 ≥ 10
b) 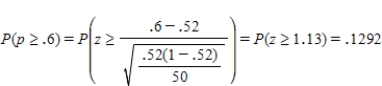 Note: Students may use the binomial distribution to get an exact answer. P(x ≥ 30) = 1 − P(x ≤ 29) = 1 − binomcdf(50, .52, 29) = 1 − .8391 = .1609
Note: Students may use the binomial distribution to get an exact answer. P(x ≥ 30) = 1 − P(x ≤ 29) = 1 − binomcdf(50, .52, 29) = 1 − .8391 = .1609
Consider the following "population": {1, 1, 3, 4}. Suppose that a random sample of size n = 2 is to be selected without replacement from this population. There are 6 possible samples (since the order of selection does not matter). Compute the sample mean for each of these samples and use that information to construct the sampling distribution of  . (Display it in table form.)
. (Display it in table form.)
(Essay)
4.8/5  (45)
(45)
As n grows larger, the mean of the sampling distribution of  gets closer to μ.
gets closer to μ.
(True/False)
4.8/5  (36)
(36)
You will be asked to sketch curves representing the distributions of a set of data, as well as the sampling distributions of the mean under different conditions. You need not get these graphs perfectly correct, but should clearly indicate different aspects of the curves, such as location, variability, and shape. The State Fisheries Department wishes to stock the Styx River with fish, and would like the species to not only survive but thrive. (The "substrate" of a river is an important determinant of the quality of spawning habitat.) The pebble diameters in the river are approximately normally distributed with a mean of 24 mm, and a standard deviation of 8 mm. The Fisheries people will select a random sample of pebbles from the Styx River in an attempt to judge the average pebble size.
a) On the scale below, sketch curves representing the distribution of the original population and the sampling distribution of  for a sample of size n = 16.
for a sample of size n = 16.  b) What is the approximate probability that a sample of n = 16 from this population would result in a sample mean greater than 30 mm?
c) Another issue that must be considered in stocking a river with fish is that not only natural predators of the fish, but fishermen and fisherwomen (fisherpersons?) will be taking fish from the river, and thus driving the population down. The graph below is a relative frequency histogram of the lengths of the gentle Hecate fish in the Styx River, as recorded during a massive fish tagging survey. The mean length for these fish was 0.8 feet.
b) What is the approximate probability that a sample of n = 16 from this population would result in a sample mean greater than 30 mm?
c) Another issue that must be considered in stocking a river with fish is that not only natural predators of the fish, but fishermen and fisherwomen (fisherpersons?) will be taking fish from the river, and thus driving the population down. The graph below is a relative frequency histogram of the lengths of the gentle Hecate fish in the Styx River, as recorded during a massive fish tagging survey. The mean length for these fish was 0.8 feet.  Suppose we were to take a random sample, n = 4, from this population of fish and measure their lengths. Describe the shape, center, and spread of the sampling distribution of
Suppose we were to take a random sample, n = 4, from this population of fish and measure their lengths. Describe the shape, center, and spread of the sampling distribution of  .
.
(Essay)
4.8/5  (31)
(31)
One method for estimating abundance of animals is known as line-intercept sampling. The theory of this method, when applied to Alaskan wolverines, predicts that π = 0.453 of attempts to locate wolverine tracks should be successful. Suppose that biologists will make 100 attempts to locate wolverine tracks in random locations in Alaska.
a) Show that this sample size is large enough to justify using the normal approximation to the sampling distribution of p.
b) What is the mean of the sampling distribution of p if the theory of line-intercept sampling is correct?
c) What is the standard deviation of the sampling distribution of p if the theory of line-intercept sampling is correct?
d) If the theory of line-intercept sampling is correct, what is the probability that a sample proportion, p, would differ from π = 0.453 by as much as 0.05?
(Essay)
4.8/5  (40)
(40)
The mean of the sampling distribution of p is π no matter how large n is.
(True/False)
4.8/5  (35)
(35)
The sampling distribution of p tends to be more spread out for larger sample sizes than for smaller sample sizes.
(True/False)
4.7/5  (35)
(35)
The principal at Thomas Jefferson High School has been asked to estimate the proportion of students at KHS who drive to school and use the school parking lot. He takes a random sample of size n = 32 students and calculates a sample proportion, p = 0.8. "Now," he exclaims, "since my sample size is greater than 30, the sampling distribution of the sample proportion is approximately normal." Write a short paragraph that explains why his statement is not correct.
(Essay)
4.8/5  (33)
(33)
Some biologists believe the evolution of handedness is linked to complex behaviors such as tool-use. Under this theory, handedness would be genetically passed on from parents to children. That is, left-handed parents would be more likely to have left-handed children than right-handed parents. An alternate theory asserts that handedness should be random, with left- and right-handedness equally likely. In a recent study using a simple random sample of n = 76 right-handed parents, 50 of the children born were right-handed. (p = 0.658.) Suppose handedness is a random occurrence with either hand equally likely to be dominant, implying that the probability of a right-handed offspring is π = 0.50.
a) Show that it is reasonable to approximate the sampling distribution of p using a normal distribution.
b) Assuming left- and right-handed children are equally likely from right-handed parents, what is the probability of observing a sample proportion of at least p = 0.658?
(Essay)
4.8/5  (40)
(40)
If π = 0.90, a sample size of n = 10 is large enough for the sampling distribution to be well approximated by a normal distribution.
(True/False)
4.8/5  (41)
(41)
One method for estimating the availability of office space in large cities is to conduct a random sample of offices, and calculate the proportion of offices currently being used. Suppose that real estate agents believe that π = 0.70 of all offices are currently occupied, and decide to take a sample to assess their belief. They are considering a sample size of n = 40.
a) Show that this sample size is large enough to justify using the normal approximation to the sampling distribution of p.
b) What is the mean of the sampling distribution of p if the real estate agents are correct? c) What is the standard deviation of the sampling distribution of p if the real estate agents are correct?
d) If the real estate agents are correct, what is the probability that a sample proportion, p, would differ from π = 0.70 by as much as 0.05?
(Essay)
4.9/5  (31)
(31)
Consider a sample from a skewed population. What is the minimum sample size to apply the Central Limit Theorem?
(Multiple Choice)
4.9/5  (41)
(41)
Suppose we artificially categorize populations as approximately normal or not, and samples as large or small. This categorization results in 4 categories:
a) Small samples from an approximately normal population
b) Large samples from an approximately normal population
c) Small samples from a population that is not approximately normal
d) Large samples from a population that is not approximately normal What, if anything, can be said about the shape of the sampling distribution of  for each of these 4 situations?
for each of these 4 situations?
(Essay)
4.7/5  (30)
(30)
Showing 1 - 20 of 27
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)