Exam 9: Project Scheduling: Pertcpm
Exam 1: Introduction63 Questions
Exam 2: An Introduction to Linear Programming66 Questions
Exam 3: Linear Programming: Sensitivity Analysis and Interpretation of Solution56 Questions
Exam 4: Linear Programming Applications in Marketing, Finance, and Operations Management63 Questions
Exam 5: Advanced Linear Programming Applications46 Questions
Exam 6: Distribution and Network Models70 Questions
Exam 7: Integer Linear Programming61 Questions
Exam 8: Nonlinear Optimization Models51 Questions
Exam 9: Project Scheduling: Pertcpm59 Questions
Exam 10: Inventory Models65 Questions
Exam 11: Waiting Line Models68 Questions
Exam 12: Simulation62 Questions
Exam 13: Decision Analysis97 Questions
Exam 14: Multicriteria Decisions50 Questions
Exam 15: Time Series Analysis and Forecasting63 Questions
Exam 16: Markov Processes49 Questions
Exam 17: Linear Programming: Simplex Method51 Questions
Exam 18: Simplex-Based Sensitivity Analysis and Duality35 Questions
Exam 19: Solution Procedures for Transportation and Assignment Problems44 Questions
Exam 20: Minimal Spanning Tree19 Questions
Exam 21: Dynamic Programming38 Questions
Select questions type
Consider a project that has been modeled as follows: 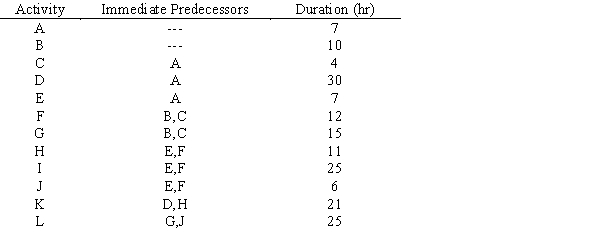
a.
Draw the PERT/CPM network for this project and determine project's expected completion time and its critical path.
b.
Can activities E and G be performed simultaneously without delaying the minimum project completion time?
c.
Can one person perform A, G, and I without delaying the project?
d.
By how much can activities G and L be delayed without delaying the entire project?
e.
How much would the project be delayed if activity G was delayed by 7 hours and activity L was delayed by 4 hours? Explain.
a.
Draw the PERT/CPM network for this project and determine project's expected completion time and its critical path.
b.
Can activities E and G be performed simultaneously without delaying the minimum project completion time?
c.
Can one person perform A, G, and I without delaying the project?
d.
By how much can activities G and L be delayed without delaying the entire project?
e.
How much would the project be delayed if activity G was delayed by 7 hours and activity L was delayed by 4 hours? Explain.
(Essay)
4.9/5  (36)
(36)
The earliest finish time for the final activity is the project duration.
(True/False)
4.9/5  (43)
(43)
Activities G, P, and R are the immediate predecessors for activity W. If the earliest finish times for the three are 12, 15, and 10, then the earliest start time for W
(Multiple Choice)
4.9/5  (47)
(47)
The linear programming model for crashing presented in the textbook assumes that any portion of the activity crash time can be achieved for a corresponding portion of the activity crashing cost.
(True/False)
4.7/5  (36)
(36)
The project manager should monitor the progress of any activity with a large time variance even if the expected time does not identify the activity as a critical activity.
(True/False)
4.8/5  (33)
(33)
The earliest start time for an activity is equal to the smallest of the earliest finish times for all its immediate predecessors.
(True/False)
4.8/5  (37)
(37)
Use the following network of related activities with their duration times (weeks) to complete a row for each activity under the column headings below. 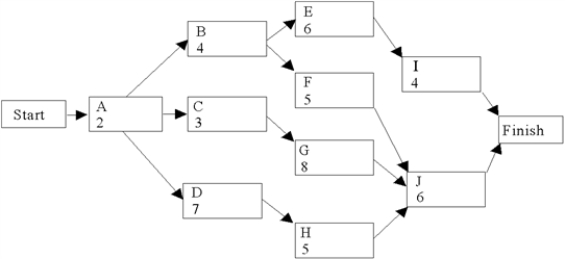
(Essay)
4.7/5  (32)
(32)
Crashing refers to an unanticipated delay in a critical path activity that causes the total time to exceed its limit.
(True/False)
4.8/5  (28)
(28)
A senior MIS design class project team has developed the following schedule of activities for their project, using their best estimate of completion times. Both written and oral reports are required. Draw the project network. Can they complete the project in the 38 class days remaining until the end of the semester? 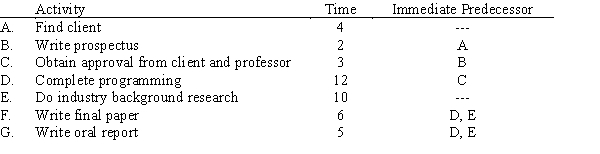
(Essay)
4.8/5  (34)
(34)
The normal distribution tends to be a better approximation of the distribution of total time for shorter projects where the critical path has relatively few activities.
(True/False)
4.9/5  (32)
(32)
Constraints in the LP models for crashing decisions are required to compare the activity's earliest finish time with the earliest finish time of each predecessor.
(True/False)
4.9/5  (33)
(33)
Critical activities are those that can be delayed without delaying the entire project.
(True/False)
4.9/5  (39)
(39)
The difference between an activity's earliest finish time and latest finish time equals the difference between its earliest start time and latest start time.
(True/False)
4.9/5  (40)
(40)
PERT and CPM are applicable only when there is no dependence among activities.
(True/False)
4.7/5  (38)
(38)
Joseph King has ambitions to be mayor of Williston, North Dakota. Joe has determined the breakdown of the steps to the nomination and has estimated normal and crash costs and times for the campaign as follows (times are in weeks). 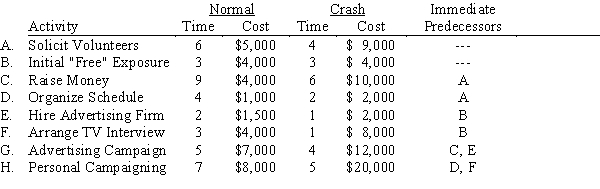
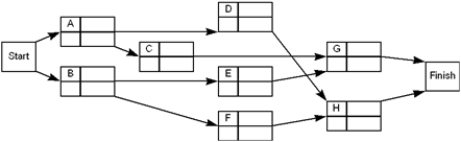
Joe King is not a wealthy man and would like to organize a 16-week campaign at minimum cost. Write and solve a linear program to accomplish this task.
Joe King is not a wealthy man and would like to organize a 16-week campaign at minimum cost. Write and solve a linear program to accomplish this task.
(Essay)
4.9/5  (29)
(29)
Showing 21 - 40 of 59
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)