Exam 18: Simplex-Based Sensitivity Analysis and Duality
Exam 1: Introduction63 Questions
Exam 2: An Introduction to Linear Programming66 Questions
Exam 3: Linear Programming: Sensitivity Analysis and Interpretation of Solution56 Questions
Exam 4: Linear Programming Applications in Marketing, Finance, and Operations Management63 Questions
Exam 5: Advanced Linear Programming Applications46 Questions
Exam 6: Distribution and Network Models70 Questions
Exam 7: Integer Linear Programming61 Questions
Exam 8: Nonlinear Optimization Models51 Questions
Exam 9: Project Scheduling: Pertcpm59 Questions
Exam 10: Inventory Models65 Questions
Exam 11: Waiting Line Models68 Questions
Exam 12: Simulation62 Questions
Exam 13: Decision Analysis97 Questions
Exam 14: Multicriteria Decisions50 Questions
Exam 15: Time Series Analysis and Forecasting63 Questions
Exam 16: Markov Processes49 Questions
Exam 17: Linear Programming: Simplex Method51 Questions
Exam 18: Simplex-Based Sensitivity Analysis and Duality35 Questions
Exam 19: Solution Procedures for Transportation and Assignment Problems44 Questions
Exam 20: Minimal Spanning Tree19 Questions
Exam 21: Dynamic Programming38 Questions
Select questions type
Explain why the zj value for a slack variable is the dual price.
(Short Answer)
4.9/5  (39)
(39)
The range of feasibility indicates right-hand side values for which
(Multiple Choice)
4.9/5  (33)
(33)
When sensitivity calculations yield several potential upper bounds and several lower bounds, how is the range
determined?
(Short Answer)
4.9/5  (35)
(35)
For an objective function coefficient change outside the range of optimality, explain how to calculate the new optimal
solution. Must you return to the (revised) initial tableau?
(Short Answer)
4.8/5  (34)
(34)
The entries in the associated slack column of the final tableau indicate the changes in the values of the current basic variables corresponding to a one-unit increase in the right-hand side.
(True/False)
4.8/5  (25)
(25)
The dual price for an equality constraint is the zj value for its artificial variable.
(True/False)
4.8/5  (31)
(31)
For the following linear programming problem
Max Z
−2x1 + x2 − x3
s.t.
2x1 + x2 ≤ 7
1x1 + x2 + x3 ≥ 4
the final tableau is 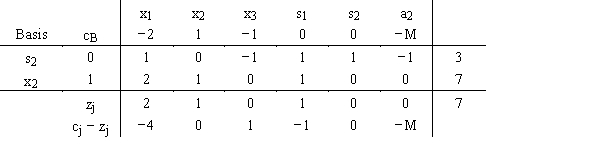
a.Find the range of optimality for c1, c2 , c3. c4, c5 , and c6.
b.Find the range of feasibility for b1, and b2.
a.Find the range of optimality for c1, c2 , c3. c4, c5 , and c6.
b.Find the range of feasibility for b1, and b2.
(Essay)
4.9/5  (23)
(23)
The dual price is the improvement in value of the optimal solution per unit increase in the value of the right-hand side associated with a linear programming problem.
(True/False)
4.9/5  (35)
(35)
For this optimal simplex tableau, the right-hand sides for the two original ≥ constraints were 300 and 250. The problem was a minimization. 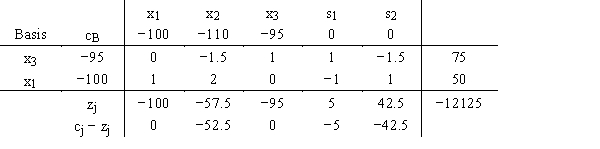
a.
What would the new solution be if the right-hand side value in the first constraint had been 325?
b.
What would the new solution be if the right-hand side value for the second constraint had been 220?
a.
What would the new solution be if the right-hand side value in the first constraint had been 325?
b.
What would the new solution be if the right-hand side value for the second constraint had been 220?
(Essay)
4.7/5  (31)
(31)
The ranges for which the right-hand side values are valid are the same as the ranges over which the dual prices are valid.
(True/False)
4.7/5  (36)
(36)
Explain the simplex tableau location of the dual constraint for each type of constraint.
(Essay)
4.9/5  (29)
(29)
Given the following linear programming problem
Max Z
0.5x1 + 6x2 + 5x3
s.t.
4x1 + 6x2 + 3x3 ≤ 24
1x1 + 1.5x2 + 3x3 ≤ 12
3x1 + x2 ≤ 12
and the final tableau is 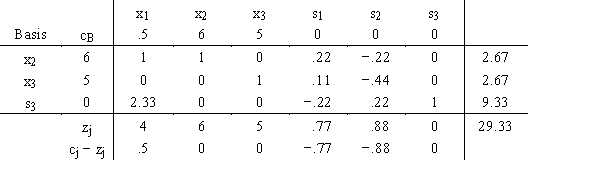
a.Find the range of optimality for c1, c2, c3, c4, c5, and c6.
b.Find the range of feasibility for b1, b2, and b3.
a.Find the range of optimality for c1, c2, c3, c4, c5, and c6.
b.Find the range of feasibility for b1, b2, and b3.
(Essay)
4.9/5  (32)
(32)
If the simplex tableau is from a maximization converted from a minimization, the signs and directions of the inequalities that give the objective function ranges will need to be adjusted to apply to the original coefficients.
(True/False)
4.8/5  (33)
(33)
As long as the objective function coefficient remains within the range of optimality, the variable values will not change although the value of the objective function could.
(True/False)
4.7/5  (30)
(30)
Showing 21 - 35 of 35
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)