Exam 8: Sampling Variability and Sampling Distributions
Exam 1: Collecting Data in Reasonable Ways56 Questions
Exam 2: Graphical Methods for Describing Data Distributions62 Questions
Exam 3: Numerical Methods for Describing Data Distributions37 Questions
Exam 4: Describing Bivariate Numerical Data70 Questions
Exam 5: Probability55 Questions
Exam 6: Random Variables and Probability Distributions72 Questions
Exam 7: An Overview of Statistical Inference - Learning From Data19 Questions
Exam 8: Sampling Variability and Sampling Distributions35 Questions
Exam 9: Estimating a Population Proportion36 Questions
Exam 10: Asking and Answering Questions About a Population Proportion31 Questions
Exam 11: Asking and Answering Questions About the Difference Between Two Proportions42 Questions
Exam 12: Asking and Answering Questions About a Population Mean51 Questions
Exam 13: Asking and Answering Questions About the Difference Between Two Means46 Questions
Exam 14: Learning From Categorical Data36 Questions
Exam 15: Understanding Relationships - Numerical Data Part 243 Questions
Exam 16: Asking and Answering Questions About More Than Two Means25 Questions
Select questions type
For which of the following combinations of sample size and population proportion would the standard deviation of  be smallest?
be smallest?
(Multiple Choice)
4.9/5  (32)
(32)
Which of the following statements is a population proportion?
(Multiple Choice)
4.9/5  (36)
(36)
For n sufficiently large, the distribution of 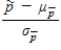 is approximately a standard normal distribution.
is approximately a standard normal distribution.
(True/False)
4.9/5  (40)
(40)
As n grows larger, the mean of the sampling distribution of  gets closer to μ.
gets closer to μ.
(True/False)
4.9/5  (32)
(32)
The distribution of 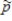 will always have the same shape as the distribution of the population being sampled.
will always have the same shape as the distribution of the population being sampled.
(True/False)
4.9/5  (25)
(25)
Consider the following "population": {2, 2, 4, 5}. Suppose that a random sample of size n = 2 is to be selected without replacement from this population. There are 6 possible samples (since the order of selection does not matter). Compute the sample mean for each of these samples and use that information to construct the sampling distribution of  . (Display it in table form.)
. (Display it in table form.)
(Essay)
4.9/5  (32)
(32)
The sampling distribution of  tends to be more spread out for larger sample sizes than for smaller sample sizes.
tends to be more spread out for larger sample sizes than for smaller sample sizes.
(True/False)
4.8/5  (32)
(32)
A random sample is to be selected from a population that has a proportion of successes p = 0.50. Determine the mean and standard deviation of the sampling distribution of 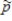 for the sample size n = 100.
for the sample size n = 100.
(Multiple Choice)
4.9/5  (44)
(44)
One method for estimating abundance of animals is known as line-intercept sampling. The theory of this method, when applied to Alaskan wolverines, predicts that p = 0.453 of attempts to locate wolverine tracks should be successful. Suppose that biologists will make 100 attempts to locate wolverine tracks in random locations in Alaska.
a)Show that this sample size is large enough to justify using the normal approximation to the sampling distribution of  .
b)What is the mean of the sampling distribution of
.
b)What is the mean of the sampling distribution of 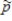 ?
c)What is the standard deviation of the sampling distribution of
?
c)What is the standard deviation of the sampling distribution of 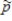 ?
d)What is the probability that a sample proportion,
?
d)What is the probability that a sample proportion, 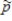 , would differ from p = 0.453 by as much as 0.05?
, would differ from p = 0.453 by as much as 0.05?
(Essay)
4.8/5  (39)
(39)
Some colleges now allow students to rent textbooks for a semester. Suppose that 73% of all students enrolled at a particular college would rent textbooks if that option were available to them. If the campus bookstore uses a random sample of size 40 to estimate the proportion of students at the college who would rent textbooks, then what is the standard deviation of the sampling distribution of 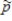 ?
?
(Multiple Choice)
4.8/5  (33)
(33)
Consider sampling from a skewed population. As the sample size, n, increases, some characteristics of the sampling distribution of 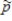 change. Does an increasing sample size cause changes in the characteristics of the sampling distribution shown below? If so, specifically how does the sampling distribution change?
a)The mean of the sampling distribution of
change. Does an increasing sample size cause changes in the characteristics of the sampling distribution shown below? If so, specifically how does the sampling distribution change?
a)The mean of the sampling distribution of 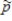 b)The standard deviation of the sampling distribution of
b)The standard deviation of the sampling distribution of 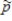 c)The shape of the sampling distribution of
c)The shape of the sampling distribution of 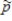
(Essay)
4.7/5  (33)
(33)
The closer p is to 0 or 1, the larger n must be in order for the distribution of 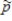 to be approximately normal.
to be approximately normal.
(True/False)
5.0/5  (46)
(46)
In a random sample of 79 students at a university, 7 had the flu.
Suppose you are interested in learning about the value of p, the proportion of all students at this university who have the flu. This proportion can be estimated using the sample proportion, 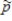 . What is the value of
. What is the value of 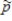 for this sample?
for this sample?
(Multiple Choice)
4.8/5  (36)
(36)
The principal at Thomas Jefferson High School has been asked to estimate the proportion of students at KHS who drive to school and use the school parking lot. He takes a random sample of size n = 32 students and calculates a sample proportion, 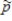 = 0.8. "Now," he exclaims, "since my sample size is greater than 30, the sampling distribution of the sample proportion is approximately normal." Write a short paragraph that explains why his statement is not correct.
= 0.8. "Now," he exclaims, "since my sample size is greater than 30, the sampling distribution of the sample proportion is approximately normal." Write a short paragraph that explains why his statement is not correct.
(Essay)
4.8/5  (31)
(31)
Showing 21 - 35 of 35
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)