Question 1

Find the definite integral by computing an area.
-$$\int _ { 0 } ^ { 5 } 7 x d x$$

Accepted Answer

A)  175 
B)  35 
C)  $\frac { 175 } { 2 }$ 
D)  $\frac { 25 } { 2 }$ 
A)  175 
B)  35 
C)  $\frac { 175 } { 2 }$ 
D)  $\frac { 25 } { 2 }$

Question 2

Use NDER on a calculator to find the numerical derivative of the function at the specified point.
-$f ( x ) = - 4 x ^ { 2 } + 7 x$ at $x = 5$

Accepted Answer

A)  3 
B)  $- 33$ 
C)  $- 13$ 
D)  33 
A)  3 
B)  $- 33$ 
C)  $- 13$ 
D)  33

Question 3

Find the derivative of the function using the definition of derivative.
-$$f ( x ) = - \frac { 2 } { x }$$

Accepted Answer

A)  $f ^ { \prime } ( x ) = - 2 x ^ { 2 }$ 
B)  $f ^ { \prime } ( x ) = - \frac { 2 } { x ^ { 2 } }$ 
C)  $f ^ { \prime } ( x ) = - 2$ 
D)  $f ^ { \prime } ( x ) = \frac { 2 } { x ^ { 2 } }$ 
A)  $f ^ { \prime } ( x ) = - 2 x ^ { 2 }$ 
B)  $f ^ { \prime } ( x ) = - \frac { 2 } { x ^ { 2 } }$ 
C)  $f ^ { \prime } ( x ) = - 2$ 
D)  $f ^ { \prime } ( x ) = \frac { 2 } { x ^ { 2 } }$

Question 4

Use NINT on a calculator to find the numerical integral of the function over the specified interval.
-$$f ( x ) = \frac { x } { 36 + x ^ { 2 } } ; [ 0,10 ]$$
Round to three decimal places.

Accepted Answer

A)  $1.899$ 
B)  $0.332$ 
C)  $0.665$ 
D)  $- 0.665$ 
A)  $1.899$ 
B)  $0.332$ 
C)  $0.665$ 
D)  $- 0.665$

Question 5

Find the limit of the function by using direct substitution.
-$$\lim _ { x \rightarrow \pi / 2 } \left( 4 e ^ { x } \cos x \right)$$

Accepted Answer

A)  0 
B)  1 
C)  $\frac { \pi } { 2 }$ 
D)  $4 \mathrm { e } ^ { \pi / 2 }$ 
A)  0 
B)  1 
C)  $\frac { \pi } { 2 }$ 
D)  $4 \mathrm { e } ^ { \pi / 2 }$

Question 6

Find the limit.
-Let $\lim _ { x \rightarrow 7 } f ( x ) = - 5$ and $\lim _ { x \rightarrow 7 } g ( x ) = - 10$. Find $\lim _ { x \rightarrow 7 } \frac { f ( x ) } { g ( x ) }$.

Accepted Answer

A)  5 
B)  2 
C)  $\frac { 1 } { 2 }$ 
D)  $- 7$ 
A)  5 
B)  2 
C)  $\frac { 1 } { 2 }$ 
D)  $- 7$

Question 7

Compute the average of the RRAM and LRAM approximations to estimate the area between the graph of the function
and the x-axis over the given interval using the indicated number of subintervals. (The function is non-negative on the
given interval).
-$f ( x ) = 16 + 6 x - x ^ { 2 } ; [ 0,4 ] ; 4$ subintervals

Accepted Answer

A)  $\frac { 225 } { 4 }$ 
B)  115 
C)  230 
D)  $\frac { 225 } { 2 }$ 
A)  $\frac { 225 } { 4 }$ 
B)  115 
C)  230 
D)  $\frac { 225 } { 2 }$

Question 8

Match the function with the correct table values.
-$$f ( x ) = \frac { x ^ { 2 } + 5 x + 6 } { x ^ { 2 } + 7 x + 12 }$$

Accepted Answer

A)  $0.7101 ; 0.7139 ; 0.7142 ; 0.7143 ; 0.7147 ; 0.7183$ 
B)  $- 1.1222 ; - 0.9202 ; - 0.9020 ; - 0.8980 ; - 0.8802 ; - 0.7182$ 
C)  $- 1.2222 ; - 1.0202 ; - 1.0020 ; - 0.9980 ; - 0.9802 ; - 0.8182$ 
D)  $- 1.3222 ; - 1.1202 ; - 1.1020 ; - 1.0980 ; - 1.0802 ; - 0.9182$ 
A)  $0.7101 ; 0.7139 ; 0.7142 ; 0.7143 ; 0.7147 ; 0.7183$ 
B)  $- 1.1222 ; - 0.9202 ; - 0.9020 ; - 0.8980 ; - 0.8802 ; - 0.7182$ 
C)  $- 1.2222 ; - 1.0202 ; - 1.0020 ; - 0.9980 ; - 0.9802 ; - 0.8182$ 
D)  $- 1.3222 ; - 1.1202 ; - 1.1020 ; - 1.0980 ; - 1.0802 ; - 0.9182$

Question 9

Find the equation of the tangent line to the curve when x has the given value.
-$$f ( x ) = \sqrt { x } ; x = 25$$

Accepted Answer

A)  $y = \frac { 1 } { 10 } x + 4$ 
B)  $y = - \frac { 1 } { 10 } x + \frac { 5 } { 2 }$ 
C)  $y = \frac { 1 } { 10 } x + \frac { 5 } { 2 }$ 
D)  $y = \frac { 1 } { 10 } x - \frac { 5 } { 2 }$ 
A)  $y = \frac { 1 } { 10 } x + 4$ 
B)  $y = - \frac { 1 } { 10 } x + \frac { 5 } { 2 }$ 
C)  $y = \frac { 1 } { 10 } x + \frac { 5 } { 2 }$ 
D)  $y = \frac { 1 } { 10 } x - \frac { 5 } { 2 }$

Question 10

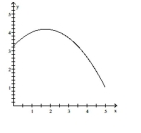
Solve the problem.
-Estimate the "RRAM" area under the graph of the function above the $x$-axis and under the graph of the function from $x = 0$ to $x = 5$. Use 5 subintervals.

Accepted Answer

A)  15.5 
B)  18.75 
C)  17.5 
D)  14.5 
A)  15.5 
B)  18.75 
C)  17.5 
D)  14.5

Question 11

Use graphs and tables to find the limit and identify any vertical asymptotes.
-$$\lim _ { x \rightarrow 9 ^ { - } } \frac { x } { x - 9 }$$

Accepted Answer

A)  9 ; no vertical asymptotes 
B)  $x ; x = 9$ 
C)  $- \infty ; x = - 9$ 
D)  $- \infty ; x = 9$ 
A)  9 ; no vertical asymptotes 
B)  $x ; x = 9$ 
C)  $- \infty ; x = - 9$ 
D)  $- \infty ; x = 9$

Question 12

Find the derivative of the function at the specified point.
-$f ( x ) = \frac { 8 } { x + 2 }$ at $x = 0$

Accepted Answer

A)  $- 2$ 
B)  $- 32$ 
C)  8 
D)  2 
A)  $- 2$ 
B)  $- 32$ 
C)  8 
D)  2

Question 13

Match the function with the correct table values.
-$$f ( x ) = \frac { x ^ { 4 } - 1 } { x - 1 }$$

Accepted Answer

A)  $4.595 ; 5.046 ; 5.095 ; 5.105 ; 5.154 ; 5.677$ 
B)  $1.032 ; 1.182 ; 1.198 ; 1.201 ; 1.218 ; 1.392$ 
C)  $3.439 ; 3.940 ; 3.994 ; 4.006 ; 4.060 ; 4.641$ 
A)  $4.595 ; 5.046 ; 5.095 ; 5.105 ; 5.154 ; 5.677$ 
B)  $1.032 ; 1.182 ; 1.198 ; 1.201 ; 1.218 ; 1.392$ 
C)  $3.439 ; 3.940 ; 3.994 ; 4.006 ; 4.060 ; 4.641$

Question 14

Solve the problem.
-A toy rocket is launched straight up from level ground. Its velocity function is $\mathrm { f } ( \mathrm { t } ) = 189 - 32 \mathrm { t } \mathrm { ft } / \mathrm { sec }$, where $\mathrm { t }$ is the number of seconds after launch. At what time does the rocket reach its maximum height?

Accepted Answer

A)  $6.23 \mathrm { sec }$ 
B)  $5.72 \mathrm { sec }$ 
C)  $5.91 \mathrm { sec }$ 
D)  $11.81 \mathrm { sec }$ 
A)  $6.23 \mathrm { sec }$ 
B)  $5.72 \mathrm { sec }$ 
C)  $5.91 \mathrm { sec }$ 
D)  $11.81 \mathrm { sec }$

Question 15

Find the limit of the function algebraically.
-$$\lim _ { x \rightarrow 3 } \frac { x ^ { 2 } - 2 x - 15 } { x + 3 }$$

Accepted Answer

A)  Does not exist 
B)  $- 8$ 
C)  0 
D)  5 
A)  Does not exist 
B)  $- 8$ 
C)  0 
D)  5

Question 16

Find the derivative of the function at the specified point.
-$g ( x ) = x ^ { 3 } + 5 x$ at $x = 1$

Accepted Answer

A)  8 
B)  3 
C)  7 
D)  6 
A)  8 
B)  3 
C)  7 
D)  6

Question 17

Find the indicated limit.
-$$\lim _ { x \rightarrow } x \cos \frac { 1 } { x }$$

Accepted Answer

A)  $\propto$ 
B)  0 
C)  $- \infty$ 
D)  1 
A)  $\propto$ 
B)  0 
C)  $- \infty$ 
D)  1

Question 18

Solve the problem.
-The position of an object at time $t$ is given by $s ( t )$. Find the instantaneous velocity at the indicated value of $t$. $s ( t ) = - 7 - 5$ t at $t = 4$

Accepted Answer

A)  5 
B)  $- 27$ 
C)  $- 5$ 
D)  $- 7$ 
A)  5 
B)  $- 27$ 
C)  $- 5$ 
D)  $- 7$

Question 19

Find the derivative of the function at the specified point.
-$f ( x ) = 5 x + 9$ at $x = 2$

Accepted Answer

A)  0 
B)  5 
C)  10 
D)  9 
A)  0 
B)  5 
C)  10 
D)  9

Question 20

Solve the problem.
-A snail travels at $0.4$ feet/min for 5 minutes. How far does it travel?

Accepted Answer

A)  $0.4 \mathrm { ft }$ 
B)  $5 \mathrm { ft }$ 
C)  $2 \mathrm { ft }$ 
D)  $\frac { 0.4 } { 5 } \mathrm { ft }$ 
A)  $0.4 \mathrm { ft }$ 
B)  $5 \mathrm { ft }$ 
C)  $2 \mathrm { ft }$ 
D)  $\frac { 0.4 } { 5 } \mathrm { ft }$

Find the definite integral by computing an area. - $\int _ { 0 } ^ { 5 } 7 x d x$

Use NDER on a calculator to find the numerical derivative of the function at the specified point. - $f ( x ) = - 4 x ^ { 2 } + 7 x$ at $x = 5$

Find the derivative of the function using the definition of derivative. - $f ( x ) = - \frac { 2 } { x }$

Use NINT on a calculator to find the numerical integral of the function over the specified interval. - $f ( x ) = \frac { x } { 36 + x ^ { 2 } } ; [ 0,10 ]$ Round to three decimal places.

Find the limit of the function by using direct substitution. - $\lim _ { x \rightarrow \pi / 2 } \left( 4 e ^ { x } \cos x \right)$

Find the limit. -Let $\lim _ { x \rightarrow 7 } f ( x ) = - 5$ and $\lim _ { x \rightarrow 7 } g ( x ) = - 10$ . Find $\lim _ { x \rightarrow 7 } \frac { f ( x ) } { g ( x ) }$ .

Match the function with the correct table values. - $f ( x ) = \frac { x ^ { 2 } + 5 x + 6 } { x ^ { 2 } + 7 x + 12 }$ $Match the function with the correct table values. - f ( x ) = \frac { x ^ { 2 } + 5 x + 6 } { x ^ { 2 } + 7 x + 12 }$

Find the equation of the tangent line to the curve when x has the given value. - $f ( x ) = \sqrt { x } ; x = 25$

Solve the problem. -Estimate the "RRAM" area under the graph of the function above the $x$ -axis and under the graph of the function from $x = 0$ to $x = 5$ . Use 5 subintervals.

Use graphs and tables to find the limit and identify any vertical asymptotes. - $\lim _ { x \rightarrow 9 ^ { - } } \frac { x } { x - 9 }$

Find the derivative of the function at the specified point. - $f ( x ) = \frac { 8 } { x + 2 }$ at $x = 0$

Match the function with the correct table values. - $f ( x ) = \frac { x ^ { 4 } - 1 } { x - 1 }$ $Match the function with the correct table values. - f ( x ) = \frac { x ^ { 4 } - 1 } { x - 1 }$

Find the limit of the function algebraically. - $\lim _ { x \rightarrow 3 } \frac { x ^ { 2 } - 2 x - 15 } { x + 3 }$

Find the derivative of the function at the specified point. - $g ( x ) = x ^ { 3 } + 5 x$ at $x = 1$

Find the indicated limit. - $\lim _ { x \rightarrow } x \cos \frac { 1 } { x }$

Solve the problem. -The position of an object at time $t$ is given by $s ( t )$ . Find the instantaneous velocity at the indicated value of $t$ . $s ( t ) = - 7 - 5$ t at $t = 4$

Find the derivative of the function at the specified point. - $f ( x ) = 5 x + 9$ at $x = 2$

Solve the problem. -A snail travels at $0.4$ feet/min for 5 minutes. How far does it travel?

Functions and Graphs

Polynomial, Power, and Rational Functions

Exponential, Logistic, and Logarithmic Functions

Trigonometric Functions

Analytic Trigonometry

Applications of Trigonometry

Systems and Matrices

Analytic Geometry in Two and Three Dimensions

Discrete Mathematics

Statistics and Probability

Prerequisites

Filters

Exam 11: An Introduction to Calculus: Limits, Derivatives, and Integrals

Find the definite integral by computing an area. - ∫057xdx\int _ { 0 } ^ { 5 } 7 x d x∫05​7xdx

Use NDER on a calculator to find the numerical derivative of the function at the specified point. - f(x)=−4x2+7xf ( x ) = - 4 x ^ { 2 } + 7 xf(x)=−4x2+7x at x=5x = 5x=5

Find the derivative of the function using the definition of derivative. - f(x)=−2xf ( x ) = - \frac { 2 } { x }f(x)=−x2​

Use NINT on a calculator to find the numerical integral of the function over the specified interval. - f(x)=x36+x2;[0,10]f ( x ) = \frac { x } { 36 + x ^ { 2 } } ; [ 0,10 ]f(x)=36+x2x​;[0,10] Round to three decimal places.

Find the limit of the function by using direct substitution. - lim⁡x→π/2(4excos⁡x)\lim _ { x \rightarrow \pi / 2 } \left( 4 e ^ { x } \cos x \right)limx→π/2​(4excosx)

Find the limit. -Let lim⁡x→7f(x)=−5\lim _ { x \rightarrow 7 } f ( x ) = - 5limx→7​f(x)=−5 and lim⁡x→7g(x)=−10\lim _ { x \rightarrow 7 } g ( x ) = - 10limx→7​g(x)=−10 . Find lim⁡x→7f(x)g(x)\lim _ { x \rightarrow 7 } \frac { f ( x ) } { g ( x ) }limx→7​g(x)f(x)​ .

Match the function with the correct table values. - f(x)=x2+5x+6x2+7x+12f ( x ) = \frac { x ^ { 2 } + 5 x + 6 } { x ^ { 2 } + 7 x + 12 }f(x)=x2+7x+12x2+5x+6​

Find the equation of the tangent line to the curve when x has the given value. - f(x)=x;x=25f ( x ) = \sqrt { x } ; x = 25f(x)=x​;x=25

Solve the problem. -Estimate the "RRAM" area under the graph of the function above the xxx -axis and under the graph of the function from x=0x = 0x=0 to x=5x = 5x=5 . Use 5 subintervals.

Use graphs and tables to find the limit and identify any vertical asymptotes. - lim⁡x→9−xx−9\lim _ { x \rightarrow 9 ^ { - } } \frac { x } { x - 9 }limx→9−​x−9x​

Find the derivative of the function at the specified point. - f(x)=8x+2f ( x ) = \frac { 8 } { x + 2 }f(x)=x+28​ at x=0x = 0x=0

Match the function with the correct table values. - f(x)=x4−1x−1f ( x ) = \frac { x ^ { 4 } - 1 } { x - 1 }f(x)=x−1x4−1​

Find the limit of the function algebraically. - lim⁡x→3x2−2x−15x+3\lim _ { x \rightarrow 3 } \frac { x ^ { 2 } - 2 x - 15 } { x + 3 }limx→3​x+3x2−2x−15​

Find the derivative of the function at the specified point. - g(x)=x3+5xg ( x ) = x ^ { 3 } + 5 xg(x)=x3+5x at x=1x = 1x=1

Find the indicated limit. - lim⁡x→xcos⁡1x\lim _ { x \rightarrow } x \cos \frac { 1 } { x }limx→​xcosx1​

Solve the problem. -The position of an object at time ttt is given by s(t)s ( t )s(t) . Find the instantaneous velocity at the indicated value of ttt . s(t)=−7−5s ( t ) = - 7 - 5s(t)=−7−5 t at t=4t = 4t=4

Find the derivative of the function at the specified point. - f(x)=5x+9f ( x ) = 5 x + 9f(x)=5x+9 at x=2x = 2x=2

Solve the problem. -A snail travels at 0.40.40.4 feet/min for 5 minutes. How far does it travel?