Exam 2: Functions and Graphs
Exam 1: Equations, Inequalities, and Modeling531 Questions
Exam 2: Functions and Graphs365 Questions
Exam 3: Polynomial and Rational Functions396 Questions
Exam 4: Exponential and Logarithmic Functions203 Questions
Exam 5: The Trigonometric Functions398 Questions
Exam 6: Trigonometric Identities and Conditional Equations674 Questions
Exam 7: Applications of Trigonometry332 Questions
Exam 8: Systems of Equations and Inequalities293 Questions
Exam 9: Matrices and Determinants218 Questions
Exam 10: The Conic Sections218 Questions
Exam 11: Sequences, Series, and Probability338 Questions
Exam 12: Basic Algebra Review226 Questions
Select questions type
Find the difference quotient, 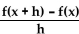 , for the function and simplify it.
-g(x) =
, for the function and simplify it.
-g(x) = 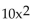 + 10x - 5
+ 10x - 5
(Multiple Choice)
4.9/5  (39)
(39)
Find the difference quotient, 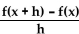 , for the function and simplify it.
-p(x) = 2 -
, for the function and simplify it.
-p(x) = 2 - 
(Multiple Choice)
5.0/5  (22)
(22)
Write a formula to express the relationship. Use k as the constant of variation.
-John kept track of the time it took him to drive to college from his home and the speed at which he drove. He found that the time t varies inversely as the speed r.
(Multiple Choice)
4.8/5  (36)
(36)
Find the constant of variation and construct the function that is expressed in each statement.
-y varies inversely as x: y = 7, when x = 16
(Multiple Choice)
4.9/5  (33)
(33)
Determine whether or not the function is one-to-one.
-f(x) = 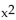 - 3
- 3
(Multiple Choice)
4.7/5  (34)
(34)
Find the constant of variation and construct the function that is expressed in each statement.
-y varies directly as x: y = 39, when x = 12
(Multiple Choice)
4.8/5  (37)
(37)
Solve.
-Find  for the function f = {(9, -6), (2, -8), (-5, 8)}.
for the function f = {(9, -6), (2, -8), (-5, 8)}.
(Multiple Choice)
4.8/5  (44)
(44)
Find the specified domain.
-For f(x) = 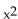 - 36 and g(x) = 2x + 3, what is the domain of f - g?
- 36 and g(x) = 2x + 3, what is the domain of f - g?
(Multiple Choice)
5.0/5  (48)
(48)
The graph of the given function is drawn with a solid line. The graph of a function, g(x), transformed from this one is
drawn with a dashed line. Find a formula for g(x).
-
(Multiple Choice)
4.8/5  (33)
(33)
The graph of a function f is given. On the same axes, sketch the graph of 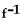 .
-
.
-
(Multiple Choice)
4.7/5  (28)
(28)
Solve the problem.
-The weight of a liquid varies directly as its volume V. If the weight of the liquid in a cubical container 5 cm on a side is 250 g, find the weight of the liquid in a cubical container 4 cm on a side.
(Multiple Choice)
4.8/5  (29)
(29)
Determine whether or not the function is one-to-one.
-f(x) = 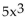 - 6
- 6
(Multiple Choice)
4.8/5  (32)
(32)
Find the constant of variation and construct the function that is expressed in each statement.
-y varies inversely as x: y = 5.25, when x = 0.52
(Multiple Choice)
4.8/5  (25)
(25)
Use transformations to graph the function and state the domain and range.
-
(Multiple Choice)
4.8/5  (38)
(38)
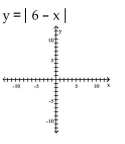
Use transformations to graph the function and state the domain and range.
-
(Multiple Choice)
4.8/5  (43)
(43)
Showing 21 - 40 of 365
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)