Exam 2: Functions and Graphs
Exam 1: Equations, Inequalities, and Modeling531 Questions
Exam 2: Functions and Graphs365 Questions
Exam 3: Polynomial and Rational Functions396 Questions
Exam 4: Exponential and Logarithmic Functions203 Questions
Exam 5: The Trigonometric Functions398 Questions
Exam 6: Trigonometric Identities and Conditional Equations674 Questions
Exam 7: Applications of Trigonometry332 Questions
Exam 8: Systems of Equations and Inequalities293 Questions
Exam 9: Matrices and Determinants218 Questions
Exam 10: The Conic Sections218 Questions
Exam 11: Sequences, Series, and Probability338 Questions
Exam 12: Basic Algebra Review226 Questions
Select questions type
Use the vertical line test to determine whether y is a function of x.
-
(Multiple Choice)
4.8/5  (21)
(21)
Find the difference quotient, 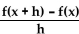 , for the function and simplify it.
-q(x) =
, for the function and simplify it.
-q(x) = 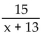
(Multiple Choice)
4.8/5  (28)
(28)
Find the specified domain.
-For f(x) = 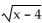 and g(x) =
and g(x) = 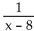 , what is the domain of f · g ?
, what is the domain of f · g ?
(Multiple Choice)
4.9/5  (29)
(29)
Graph the pair of functions on the same plane. Use a dashed line for g(x).
-
(Multiple Choice)
5.0/5  (27)
(27)
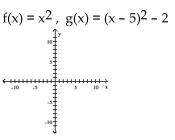
Graph the pair of functions on the same plane. Use a dashed line for g(x).
-
(Multiple Choice)
4.9/5  (36)
(36)
Graph the function as a solid curve and its inverse as a dashed curve.
-f(x) = 2x + 2 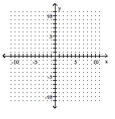
(Multiple Choice)
4.8/5  (31)
(31)
Find the requested composition of functions.
-Given f(x) = 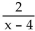 and g(x) =
and g(x) = 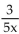 , find
, find 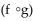 (x).
(x).
(Multiple Choice)
5.0/5  (36)
(36)
Decide whether or not the functions are inverses of each other.
-f(x) = 9x - 9, g(x) = 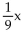 + 1
+ 1
(Multiple Choice)
4.7/5  (35)
(35)
Solve the problem.
-If f varies jointly as  and h, and f = -16 when q = 2 and h = 2, find f when q = 4 and h = 5.
and h, and f = -16 when q = 2 and h = 2, find f when q = 4 and h = 5.
(Multiple Choice)
4.8/5  (44)
(44)
Write the equation of the graph after the indicated transformation(s).
-The graph of  is translated 3 units to the right.
is translated 3 units to the right.
(Multiple Choice)
4.9/5  (38)
(38)
Solve the problem.
-Assume that the sales of a certain appliance dealer are approximated by a linear function. Suppose that sales were $4500 in 1982 and $63,000 in 1987. Let x = 0 represent 1982. Find the equation giving yearly sales S(x).
(Multiple Choice)
4.8/5  (35)
(35)
Write the equation of the graph after the indicated transformation(s).
-The graph of y = x is vertically stretched by a factor of 5.1. This graph is then reflected across the x-axis. Finally, the graph is shifted 0.42 units downward.
(Multiple Choice)
4.8/5  (41)
(41)
Provide an appropriate response.
-True or false? If f is a one-to-one function and the graph of f lies completely within the first quadrant, then the
graph of 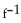 lies completely within the first quadrant.
lies completely within the first quadrant.
(True/False)
4.7/5  (28)
(28)
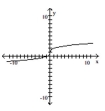
Determine the intervals on which the function is increasing, decreasing, and constant.
-
(Multiple Choice)
4.8/5  (34)
(34)
Solve the problem.
-If f varies jointly as 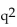 and h, and f = 54 when q = 3 and h = 3, find k.
and h, and f = 54 when q = 3 and h = 3, find k.
(Multiple Choice)
4.7/5  (40)
(40)
The graph of a function f is given. On the same axes, sketch the graph of 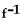 .
-
.
-
(Multiple Choice)
4.9/5  (37)
(37)
Showing 81 - 100 of 365
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)