Exam 8: Random Variables

Use the following information for questions: The Southside Bowling Alley has collected data on the number of children that come to birthday parties held at the bowling alley. Let the random variable X = the number of children per party. The distribution for the random variable X is given below. -What is the standard deviation for the number of children per party? Include the appropriate symbol and units in your answer.

Use the following information for questions: John and Mary both leave for class at the same time. The time it takes them to get to class, (J = time for John, M = time for Mary) are independent normal random variables. For John, the mean time E(J) = 10 minutes with variance V(J) = 4 minutes. For Mary, the mean time E(M) = 8 minutes with variance V(M) = 1 minute. -What is the probability that Mary will get to class before John does?

The expected value for a random variable is

Use the following information for questions: The average time taken to complete an exam, X, follows a normal probability distribution with mean = 60 minutes and standard deviation 30 minutes. -By what time should 99% of the students have finished the exam? (i.e. What is the 99^th percentile for X?)

Use the following information for questions: The probability distribution for X = number of heads in 4 tosses of a fair coin is partially given in the table below. -What is the probability of getting at least one head?

The expected value of a random variable is the

Use the following information for questions: Some managers of companies use employee rankings to laud the best and let go of the worst. Suppose the distribution of rankings of employees at a large company is normal with a mean of 65 points and a standard deviation of 6 points. -Using the provided model for rankings, what is the cut-off for an employee to be "weeded out?"

A random variable cannot be both continuous and

Which one of these 'X' variables is a discrete random variable?

Use the following information for questions: Find the requested probability for the standard normal random variable Z. -What is the probability that Z is greater than 2, P(Z > 2)?

Keeping in mind that a normal distribution is a model for a continuous variable, which one of the following variables cannot possibly have a normal distribution?

Use the following information for questions: Ellen is taking 4 courses for the semester. She believes that the probability distribution function for X = the number of courses for which she will get an A grade is given below. -What is the value of the cumulative probability distribution at 3, i.e. P(X ≤ 3)?

Use the following information for questions: For each situation, decide if the random variable described is a discrete random variable or a continuous random variable. -Random variable X = the time (in seconds) it takes one email to travel between a sender and receiver.

Use the following information for questions: The time it takes a student to finish buying his/her text books, W, is a normal random variable. This variable W is the sum of two other normal variables, X and Y (W = X + Y), where X = the time to wait in line at the ATM machine to get cash, and Y = the time to wait in line at the cashier to buy the books. Assume that X and Y are independent normal random variables, with the following means and standard deviations: -What is the standard deviation for the time it takes a student to finish buying his/her text books?

Use the following information for questions: In an experiment, a person guesses which one of three different cards a researcher has randomly picked (and hidden from the person who guesses). This is repeated four times, replacing the cards each time. Let X = number of correct guesses in the four tries. The probability distribution for X, assuming the person is just guessing, is partially provided below. 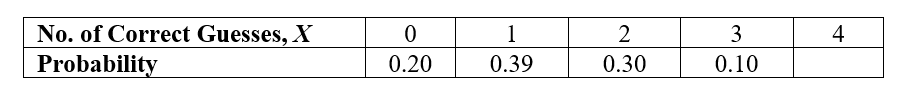-What is the value of P(X $\le$ 2) = probability that number of correct guesses is less than or equal to 2?

A landscaping company claims that 90% of the trees they plant survive (defined as being still alive one year from planting). If a tree does not survive, the company will replace the tree with a new one. A homeowner will have 5 trees planted in his yard by this landscaping company. Consider these 5 trees to be a random sample of all trees planted by this company. If the company's claim is correct, what is the probability that all 5 of the trees will survive?

Use the following information for questions: Find the requested probability for the standard normal random variable Z. -What is the probability that Z is between -1.2 and 1.45, P(-1.2 $\leq$ Z $\leq$ 1.45)?

Use the following information for questions: A child is observing squirrels in the park and notices that some are black and some are gray. For the next five squirrels she sees, she counts X = the number of black squirrels. Suppose X is a binomial random variable with n = 5 and p = 0.50. -What is the probability that she will see exactly two black squirrels out of the five?

Use the following information for questions: Ellen is taking 4 courses for the semester. She believes that the probability distribution function for X = the number of courses for which she will get an A grade is given below. -What is the probability that Ellen will get at least 2 A's?

The formula for the standard deviation for any discrete random variables with values x_i and corresponding probabilities p_i is: