Exam 4: Exponential and Logarithmic Functions
Exam 1: Algebra and Equations409 Questions
Exam 2: Graphs, Lines, and Inequalities255 Questions
Exam 3: Functions and Graphs323 Questions
Exam 4: Exponential and Logarithmic Functions192 Questions
Exam 5: Mathematics of Finance183 Questions
Exam 6: Systems of Linear Equations and Matrices215 Questions
Exam 7: Linear Programming203 Questions
Exam 8: Sets and Probability240 Questions
Exam 9: Counting, Probability Distributions, and Further Topics in Probability210 Questions
Exam 10: Introduction to Statistics169 Questions
Exam 11: Differential Calculus342 Questions
Exam 12: Applications of the Derivative220 Questions
Exam 13: Integral Calculus227 Questions
Exam 14: Multivariate Calculus152 Questions
Select questions type
Solve the problem.
-In the formula is the amount of radioactive material remaining from an initial amount I at a given time and is a negative constant determined by the nature of the material. An artifact is discovered at a certain site. If it has of the carbon-14 it originally contained, what is the approximate age of the artifact? (carbon-14 decays at the rate of annually.) (Round to the nearest year.)
(Multiple Choice)
4.9/5  (33)
(33)
Solve the problem.
-The decay of of an isotope is given by , where is time in years. Find the amount left after 13 years.
(Multiple Choice)
4.9/5  (37)
(37)
Write the logarithmic and exponential equations associated with the display.
-
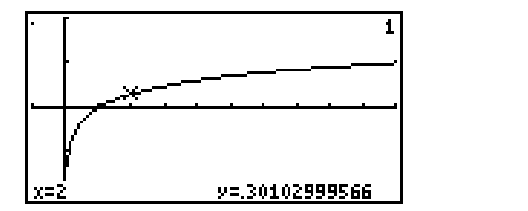
(Multiple Choice)
5.0/5  (26)
(26)
Write the word or phrase that best completes each statement or answers the question.
-
 The graph of an exponential function with base a is given. Sketch the graph of . Give the domain and range of .
The graph of an exponential function with base a is given. Sketch the graph of . Give the domain and range of .
(Essay)
4.9/5  (34)
(34)
Solve the problem.
-How long must be in a bank at compounded annually to become ? (Use the function , where is the amount initially placed in savings, is the time in years, and is the balance after time . Round to the nearest year.)
(Multiple Choice)
4.9/5  (28)
(28)
Showing 181 - 192 of 192
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)