Exam 11: Experimental Design and Analysis of Variance
Exam 1: An Introduction to Business Statistics54 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Methods90 Questions
Exam 3: Descriptive Statistics: Numerical Methods149 Questions
Exam 4: Probability135 Questions
Exam 5: Discrete Random Variables128 Questions
Exam 6: Continuous Random Variables150 Questions
Exam 7: Sampling and Sampling Distributions116 Questions
Exam 8: Confidence Intervals144 Questions
Exam 9: Hypothesis Testing148 Questions
Exam 10: Statistical Inferences Based on Two Samples132 Questions
Exam 11: Experimental Design and Analysis of Variance115 Questions
Exam 12: Chi-Square Tests96 Questions
Exam 13: Simple Linear Regression Analysis148 Questions
Exam 14: Multiple Regression122 Questions
Exam 15: Model Building and Model Diagnostics102 Questions
Exam 16: Time Series Forecasting150 Questions
Exam 17: Process Improvement Using Control Charts122 Questions
Exam 18: Nonparametric Methods97 Questions
Exam 19: Decision Theory90 Questions
Select questions type
Consider the one-way ANOVA table.  What is the mean square error?
What is the mean square error?
(Multiple Choice)
4.9/5  (33)
(33)
Consider the following partial analysis of variance table from a randomized block design with 6 blocks and 4 treatments.  Calculate the degrees of freedom for blocks.
Calculate the degrees of freedom for blocks.
(Multiple Choice)
4.8/5  (42)
(42)
A sum of squares that measures the total amount of variability in the observed values of the response variable is referred to as:
(Multiple Choice)
4.8/5  (29)
(29)
Consider the following partial analysis of variance table from a randomized block design with 10 blocks and 6 treatments. 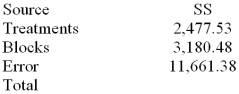 What is the mean square error?
What is the mean square error?
(Multiple Choice)
4.9/5  (30)
(30)
The ___________________ units are the entities (objects,people,etc. )to which the treatments are assigned.
(Multiple Choice)
4.9/5  (32)
(32)
When using a completely randomized design (one - way analysis of variance),the calculated F statistic will decrease
(Multiple Choice)
4.8/5  (38)
(38)
Find Tukey's simultaneous 95 percent confidence interval for 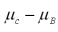 where
where 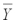 C = 51.5,
C = 51.5, 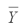 B = 55.8,and MSE = 6.125.There were 4 treatments and 24 observations total and the number of observations were equal in each group.
B = 55.8,and MSE = 6.125.There were 4 treatments and 24 observations total and the number of observations were equal in each group.
(Multiple Choice)
4.9/5  (40)
(40)
Consider the following calculations for a one-way analysis of variance from a completely randomized design with 20 total observations.The response variable is sales in millions of dollars and four treatment levels represent the four regions that the company serves. MSE = 101.25  overall = 39
overall = 39 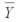 1 = 33
1 = 33 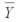 2 = 43
2 = 43 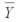 3 = 49
3 = 49 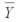 4 = 31
Perform a pairwise comparison between treatment mean 1 and treatment mean 4 by computing a Tukey 95% simultaneous confidence interval.
4 = 31
Perform a pairwise comparison between treatment mean 1 and treatment mean 4 by computing a Tukey 95% simultaneous confidence interval.
(Multiple Choice)
4.7/5  (27)
(27)
In general,a Tukey simultaneous 100 (1 -  )percent confidence interval is _____ than the corresponding individual 100 (1 -
)percent confidence interval is _____ than the corresponding individual 100 (1 - 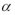 )percent confidence interval.
)percent confidence interval.
(Multiple Choice)
4.8/5  (41)
(41)
 Consider the randomized block design with 4 blocks and 3 treatments given above. What is the error sum of squares?
Consider the randomized block design with 4 blocks and 3 treatments given above. What is the error sum of squares?
(Multiple Choice)
4.9/5  (31)
(31)
In one-way ANOVA,a large value of F results when the within-treatment variability is large compared to the between-treatment variability.
(True/False)
4.8/5  (30)
(30)
The degrees of freedom error (within group variation)of a completely randomized design (one-way)ANOVA test with 4 groups and 15 observations per each group is:
(Multiple Choice)
4.8/5  (30)
(30)
In a completely randomized ANOVA,other things equal as the sample means get closer to each other,the probability of rejecting the null hypothesis:
(Multiple Choice)
4.8/5  (38)
(38)
When we compute 100 (1 - )confidence intervals,the value of is called the
(Multiple Choice)
4.8/5  (44)
(44)
In a completely randomized (one-way)ANOVA,other things equal as the sample means get closer to each other the probability of rejecting the null hypothesis decreases.
(True/False)
4.9/5  (31)
(31)
Consider the one-way ANOVA table.  If there are an equal number of observations in each group,then each group (treatment level)consists of ______ observations.
If there are an equal number of observations in each group,then each group (treatment level)consists of ______ observations.
(Multiple Choice)
4.9/5  (37)
(37)
Consider the following partial analysis of variance table from a randomized block design with 6 blocks and 4 treatments.  Test H0: There is no difference between blocks at
Test H0: There is no difference between blocks at  = .05.
= .05.
(Multiple Choice)
4.7/5  (31)
(31)
We have just performed a one-way ANOVA on a given set of data and did not reject the null hypothesis for the ANOVA F test.Assume that we are able to perform a randomized block design ANOVA on the same data.For the randomized block design ANOVA,the null hypothesis for equal treatments will ______ be rejected.
(Multiple Choice)
4.8/5  (42)
(42)
Consider the following partial analysis of variance table from a randomized block design with 10 blocks and 6 treatments.  Test Ho: there is no difference between blocks at
Test Ho: there is no difference between blocks at  = .05.
= .05.
(Multiple Choice)
4.8/5  (27)
(27)
 Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for error?
Consider the randomized block design with 4 blocks and 3 treatments given above. What are the degrees of freedom for error?
(Multiple Choice)
4.7/5  (33)
(33)
Showing 41 - 60 of 115
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)