Exam 15: Multiple Integrals
Exam 1: Functions and Limits117 Questions
Exam 2: Derivatives151 Questions
Exam 3: Applications of Differentiation153 Questions
Exam 4: Integrals95 Questions
Exam 5: Applications of Integration120 Questions
Exam 6: Inverse Functions127 Questions
Exam 7: Techniques of Integration124 Questions
Exam 8: Further Applications of Integration86 Questions
Exam 9: Differential Equations67 Questions
Exam 10: Parametric Equations and Polar Coordinates72 Questions
Exam 11: Infinite Sequences and Series158 Questions
Exam 12: Vectors and the Geometry of Space60 Questions
Exam 13: Vector Functions93 Questions
Exam 14: Partial Derivatives132 Questions
Exam 15: Multiple Integrals124 Questions
Exam 16: Vector Calculus137 Questions
Exam 17: Second-Order Differential Equations63 Questions
Exam 18: Final Exam44 Questions
Select questions type
Find the mass and the center of mass of the lamina occupying the region R, where R is the region bounded by the graphs of the equations 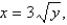
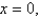 and
and  and having the mass density
and having the mass density 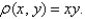
(Essay)
4.7/5  (32)
(32)
Find the area of the part of the sphere  that lies inside the paraboloid
that lies inside the paraboloid 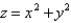 .
.
(Multiple Choice)
4.8/5  (33)
(33)
Find the volume of the solid bounded by the surface 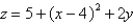 and the planes
and the planes 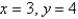 and coordinate planes.
and coordinate planes.
(Multiple Choice)
4.8/5  (29)
(29)
Find the area of the surface. The part of the sphere 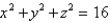 that lies above the plane
that lies above the plane 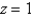 .
.
(Multiple Choice)
4.9/5  (31)
(31)
Find the mass and the center of mass of the lamina occupying the region R, where R is the triangular region with vertices 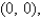
 and
and 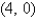 , and having the mass density
, and having the mass density 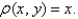
(Multiple Choice)
4.8/5  (41)
(41)
Use the given transformation to evaluate the integral. 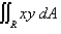 , where R is the region in the first quadrant bounded by the lines
, where R is the region in the first quadrant bounded by the lines 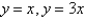 and the hyperbolas
and the hyperbolas  .
.
(Multiple Choice)
4.8/5  (27)
(27)
Evaluate the integral by making an appropriate change of variables. Round your answer to two decimal places. 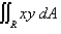 R is the parallelogram bounded by the lines
R is the parallelogram bounded by the lines 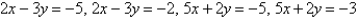 .
.
(Essay)
4.9/5  (29)
(29)
Evaluate the double integral. 
 is bounded by the circle with center the origin and radius
is bounded by the circle with center the origin and radius 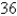 .
.
(Essay)
4.9/5  (40)
(40)
Calculate the iterated integral. Round your answer to two decimal places. 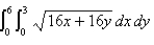
(Multiple Choice)
4.8/5  (32)
(32)
Find the volume of the solid bounded in the first octanat bounded by the cylinder  and the planes
and the planes 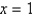 .
.
(Short Answer)
4.7/5  (40)
(40)
Use cylindrical coordinates to evaluate the triple integral 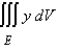 where E is the solid that lies between the cylinders
where E is the solid that lies between the cylinders  and
and 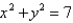 above the xy-plane and below the plane
above the xy-plane and below the plane 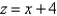 .
.
(Multiple Choice)
4.8/5  (25)
(25)
Find the area of the surface. The part of the surface  that lies above the xy-plane.
that lies above the xy-plane.
(Multiple Choice)
5.0/5  (37)
(37)
Find the area of the part of the plane 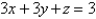 that lies in the first octant.
that lies in the first octant.
(Essay)
4.7/5  (34)
(34)
Use spherical coordinate to find the volume above the cone  and inside sphere
and inside sphere 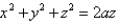 .
.
(Essay)
4.8/5  (27)
(27)
Evaluate the integral 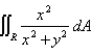 , where R is the annular region bounded by the circles
, where R is the annular region bounded by the circles  and
and 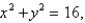 by changing to polar coordinates.
by changing to polar coordinates.
(Essay)
4.9/5  (36)
(36)
Showing 21 - 40 of 124
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)