Exam 13: Queuing Theory

Customers arrive at a store randomly, following a Poisson distribution at an average rate of 90 per hour. How many customers would you expect to arrive in a 20 minute period?

Exhibit 13.4 The following questions refer to the information and output below. A grocery store can serve an average of 360 customers per hour. The service times are exponentially distributed. The store has 4 checkout lines each of which serves 90 customers per hour. Customers arrive at the store at a Poisson rate of 240 customers per hour. The following queuing analysis spreadsheet was developed from this information. 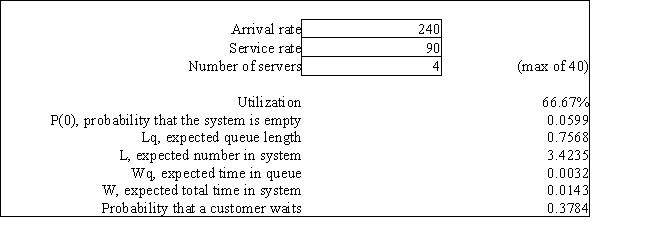-Refer to Exhibit 13.4. Based on this report what is the average number of customers waiting for a checker?

Exhibit 13.2 The following questions refer to the information and output below. A barber shop has one barber who can give 12 haircuts per hour. Customers arrive at a rate of 8 customers per hour. Customer inter-arrival times and service times are exponentially distributed. The following queuing analysis spreadsheet was developed from this information. 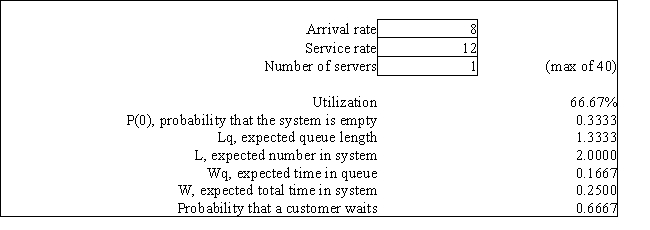-Refer to Exhibit 13.2. Based on this report what is the average waiting time before the barber begins a customer's haircut?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -The M/M/s model with finite capacity queue can be used to model

Exhibit 13.3 The following questions refer to the information below. A company has recorded the following customer inter-arrival times and service times for 10 customers at one of its single teller service lines. Assume the data are exponentially distributed and the 10 data points represent a reasonable sample. -Refer to Exhibit 13.3. What is the mean service rate per hour?

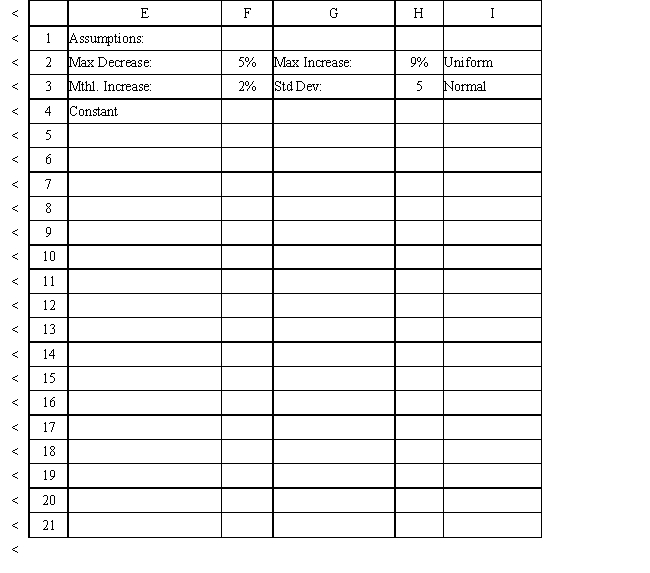
Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. 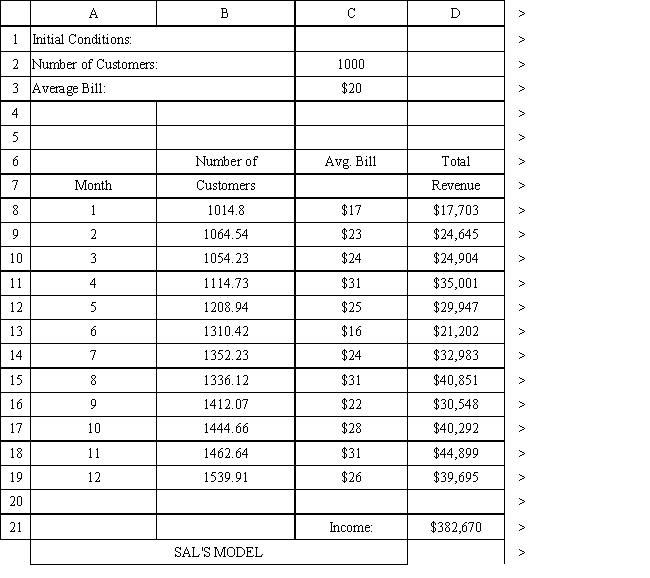-One way to improve performance of a queuing system from the customer perspective is to reduce the number of servers.

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. -A barber shop has one barber, a Poisson arrival rate and exponentially distributed service times. What is the Kendall notation for this system?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -Refer to Exhibit 13.1. What is the probability that a customer must wait in queue before being served?

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. -An arrival process is memoryless if the time until the next arrival occurs is inversely proportional to the time elapsed since the last arrival.

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. -The number of arrivals to a store follows a Poisson distribution with mean λ = 10/hour. What is the mean inter-arrival time?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -The service times for a grocery store with one checkout line have a mean of 3 minutes and a standard deviation of 20 seconds. Customer arrivals at the checkout stand follow a Poisson distribution. What type of system is it?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -Refer to Exhibit 13.1. What is the probability that a customer can go directly into service without waiting in line?

Exhibit 13.6 The following questions refer to the information and output below. The university computer lab has 10 computers which are constantly being used by students. Users need help from the one lab assistant fairly often. Students ask for help at a Poisson rate of with an average of 4 requests per hour for any one computer. The assistant answers questions as quickly as possible and the service time follows an exponential distribution with mean of 1 minute per help session. The following queuing analysis spreadsheet was developed from this information. -Refer to Exhibit 13.6. Based on this report what is the probability that a student will not get instantaneous help?

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. -The M in M/G/1 stands for

Exhibit 13.6 The following questions refer to the information and output below. The university computer lab has 10 computers which are constantly being used by students. Users need help from the one lab assistant fairly often. Students ask for help at a Poisson rate of with an average of 4 requests per hour for any one computer. The assistant answers questions as quickly as possible and the service time follows an exponential distribution with mean of 1 minute per help session. The following queuing analysis spreadsheet was developed from this information. 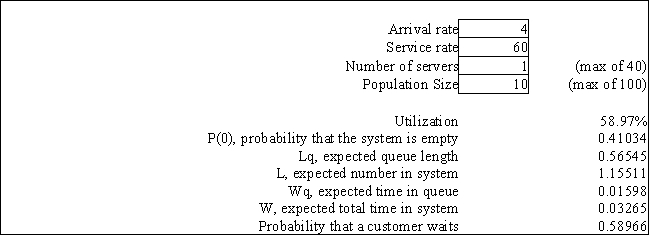-Refer to Exhibit 13.6. Based on this report how much time do students spend getting help before they can resume work on their computers?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -If cell B2 contains the value for μ and cell A5 contains the value for T, what formula should go in cell B5 to compute the P(Service time) ≤ T for this exponential distribution?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -A jockey refers to

Exhibit 12.5 The following questions use the information below. The owner of Sal's Italian Restaurant wants to study the growth of his business using simulation. He is interested in simulating the number of customers and the amount ordered by customers each month. He currently serves 1000 customers per month and feels this can vary uniformly between a decrease of as much as 5% and an increase of up to 9%. The bill for each customer is a normally distributed random variable with a mean of $20 and a standard deviation of $5. The average order has been increasing steadily over the years and the owner expects the mean order will increase by 2% per month. You have created the following spreadsheet to simulate the problem. -A balk refers to

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -Which of the following is the typical operating characteristic for the probability an arriving unit has to wait for service?

Exhibit 13.1 The following questions are based on the output below. A store currently operates its service system with 1 operator. Arrivals follow a Poisson distribution and service times are exponentially distributed. The following spreadsheet has been developed for the system. -A common queue discipline used in practice is