Exam 9: A: large-Sample Tests of Hypotheses
Exam 1: Describing Data With Graphs134 Questions
Exam 2: Describing Data With Numerical Measures235 Questions
Exam 3: Describing Bivariate Data57 Questions
Exam 4: A: probability and Probability Distributions107 Questions
Exam 4: B: probability and Probability Distributions157 Questions
Exam 5: Several Useful Discrete Distributions166 Questions
Exam 6: The Normal Probability Distribution235 Questions
Exam 7: Sampling Distributions231 Questions
Exam 8: Large-Sample Estimation187 Questions
Exam 9: A: large-Sample Tests of Hypotheses154 Questions
Exam 9: B: large-Sample Tests of Hypotheses106 Questions
Exam 10: A: Inference From Small Samples192 Questions
Exam 10: B: Inference From Small Samples124 Questions
Exam 11: A: The Analysis of Variance136 Questions
Exam 11: B: The Analysis of Variance137 Questions
Exam 12: A: linear Regression and Correlation131 Questions
Exam 12: B: linear Regression and Correlation171 Questions
Exam 13: Multiple Regression Analysis232 Questions
Exam 14: Analysis of Categorical Data158 Questions
Exam 15: A:nonparametric Statistics139 Questions
Exam 15: B:nonparametric Statistics95 Questions
Select questions type
In estimating the difference between two population means, the estimate for the standard deviation of the sampling distribution of 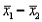 is found by taking the square root of the sum of the two sample variances.
is found by taking the square root of the sum of the two sample variances.
(True/False)
4.9/5  (35)
(35)
If you wish to construct a confidence interval estimate for the difference between two population means, what would an increase in the sample sizes used result in?
(Multiple Choice)
4.8/5  (29)
(29)
When testing 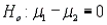 vs.
vs. 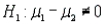 , the observed value of the z-score was found to be -2.15. What is the p-value for this test?
, the observed value of the z-score was found to be -2.15. What is the p-value for this test?
(Multiple Choice)
4.8/5  (31)
(31)
What is the rejection region for testing  at the 0.05 level of significance?
at the 0.05 level of significance?
(Multiple Choice)
4.7/5  (35)
(35)
A two-tailed test of hypothesis for a population mean 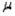 with a significance level
with a significance level 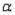 equal to 0.05 will have a critical value z equal to 0.475.
equal to 0.05 will have a critical value z equal to 0.475.
(True/False)
4.9/5  (28)
(28)
Which of the following would be an appropriate null hypothesis to test a proportion?
(Multiple Choice)
4.7/5  (32)
(32)
If the power of a statistical test is 0.9207, then the probability of accepting a false null hypothesis is 0.0793.
(True/False)
4.9/5  (33)
(33)
A one-tailed hypothesis test of the population proportion is used when the alternative hypothesis takes the form 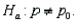
(True/False)
4.9/5  (30)
(30)
Which of the following conditions must hold before one can make use of the standard normal distribution for constructing a confidence interval estimate for the population proportion p?
(Multiple Choice)
4.9/5  (38)
(38)
The z-test can be used to determine whether two population means are equal.
(True/False)
4.7/5  (25)
(25)
The necessary conditions having been met, a two-tailed test is being conducted to test the difference between two population proportions. The two sample proportions are 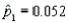 and
and 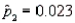 , and the standard error of the sampling distribution of
, and the standard error of the sampling distribution of 
 is 0.0085. Under these circumstances, the calculated value of the test statistic will be z = 3.41.
is 0.0085. Under these circumstances, the calculated value of the test statistic will be z = 3.41.
(True/False)
4.9/5  (42)
(42)
The sampling distribution of 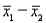 is normal if the sampled populations are normal, and approximately normal if the populations are non-normal and the sample sizes
is normal if the sampled populations are normal, and approximately normal if the populations are non-normal and the sample sizes 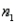 and
and 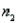 are large.
are large.
(True/False)
4.7/5  (44)
(44)
The probabilities of committing Type I and Type II errors are related such that when one is increased, the other will increase as well.
(True/False)
4.8/5  (34)
(34)
If you wish to test whether two populations means are the same, the appropriate null and alternative hypotheses would be 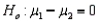 vs.
vs. 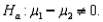
(True/False)
4.8/5  (31)
(31)
The p-value or observed significance level measures the strength of the evidence against the alternative hypothesis.
(True/False)
4.8/5  (39)
(39)
The sampling distribution of 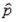 is approximately normal, provided that the sample size is large enough (n > 30).
is approximately normal, provided that the sample size is large enough (n > 30).
(True/False)
4.7/5  (32)
(32)
Suppose in testing a hypothesis about a proportion, the z test statistic is computed to be 1.92. The null hypothesis should be rejected if the chosen level of significance is 0.01 and a two-tailed test is used.
(True/False)
4.8/5  (43)
(43)
When formulating a hypothesis test about a population mean, the alternative hypothesis should avoid using an equality.
(True/False)
4.7/5  (36)
(36)
The necessary conditions having been met, a lower-tailed test is being conducted for the difference between two population proportions. If the value of the test statistic is -2.43, then the null hypothesis cannot be rejected at 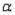 = 0.025.
= 0.025.
(True/False)
4.8/5  (33)
(33)
Suppose in testing a hypothesis about a proportion, the p-value is computed to be 0.038. The null hypothesis should be rejected if the chosen level of significance is 0.05.
(True/False)
4.9/5  (26)
(26)
Showing 61 - 80 of 154
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)