Exam 9: A: large-Sample Tests of Hypotheses
Exam 1: Describing Data With Graphs134 Questions
Exam 2: Describing Data With Numerical Measures235 Questions
Exam 3: Describing Bivariate Data57 Questions
Exam 4: A: probability and Probability Distributions107 Questions
Exam 4: B: probability and Probability Distributions157 Questions
Exam 5: Several Useful Discrete Distributions166 Questions
Exam 6: The Normal Probability Distribution235 Questions
Exam 7: Sampling Distributions231 Questions
Exam 8: Large-Sample Estimation187 Questions
Exam 9: A: large-Sample Tests of Hypotheses154 Questions
Exam 9: B: large-Sample Tests of Hypotheses106 Questions
Exam 10: A: Inference From Small Samples192 Questions
Exam 10: B: Inference From Small Samples124 Questions
Exam 11: A: The Analysis of Variance136 Questions
Exam 11: B: The Analysis of Variance137 Questions
Exam 12: A: linear Regression and Correlation131 Questions
Exam 12: B: linear Regression and Correlation171 Questions
Exam 13: Multiple Regression Analysis232 Questions
Exam 14: Analysis of Categorical Data158 Questions
Exam 15: A:nonparametric Statistics139 Questions
Exam 15: B:nonparametric Statistics95 Questions
Select questions type
If a hypothesis test leads to incorrectly accepting the null hypothesis, a Type I error has been committed.
(True/False)
4.9/5  (30)
(30)
The lower limit of the 90% confidence interval for the population proportion p, given that n = 400 and 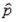 = 0.10, is 0.1247.
= 0.10, is 0.1247.
(True/False)
4.8/5  (48)
(48)
In testing 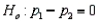 vs.
vs. 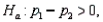 the test statistic value was found to be z = 1.28. In this case, the p-value of the test would be approximately 0.1003.
the test statistic value was found to be z = 1.28. In this case, the p-value of the test would be approximately 0.1003.
(True/False)
4.9/5  (33)
(33)
Hypothesis testing is a systematic approach to assessing tentative beliefs about reality, which involves confronting those beliefs with evidence and deciding, in light of this evidence, whether the beliefs can be maintained as reasonable or must be discarded as untenable.
(True/False)
4.8/5  (28)
(28)
If the probability of committing a Type I error is set at 0.10, then the probability of committing a Type II error will be 0.90, since the sum of probabilities must be 1.0.
(True/False)
4.9/5  (33)
(33)
In order to calculate the p-value associated with a test, it is necessary to know the level of significance 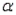 .
.
(True/False)
4.8/5  (30)
(30)
If a decision maker is concerned that the chance of committing a Type II error is large, one way to reduce the risk is to increase the significance level.
(True/False)
4.9/5  (31)
(31)
In testing a hypothesis about a population proportion p, the z-test statistic measures how close the computed sample proportion 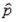 has come to the hypothesized population parameter.
has come to the hypothesized population parameter.
(True/False)
4.9/5  (28)
(28)
The probability of making the Type II error of incorrectly rejecting a true null hypothesis equals 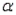 and is called the test's significance level, or risk.
and is called the test's significance level, or risk.
(True/False)
4.8/5  (33)
(33)
A two-tailed test of the population proportion produces a test statistic z = 1.77. The p-value of the test is 0.4616.
(True/False)
4.8/5  (34)
(34)
The p-value in hypothesis testing equals the probability, 1 - 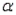 , of avoiding the Type I error of erroneously rejecting a null hypothesis that is in fact true.
, of avoiding the Type I error of erroneously rejecting a null hypothesis that is in fact true.
(True/False)
4.7/5  (27)
(27)
The necessary conditions having been met, a two-tailed test is being conducted for the difference between two population proportions. If the value of the test statistic is 1.96, then the null hypothesis is rejected at 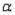 = 0.10.
= 0.10.
(True/False)
4.8/5  (24)
(24)
The p-value of a statistical test measures the actual risk of committing a Type I error.
(True/False)
4.9/5  (25)
(25)
Type II error is typically greater for two-tailed hypothesis tests than for one-tailed tests.
(True/False)
4.9/5  (37)
(37)
A Type I error is represented by  , and is the probability of not rejecting a false null hypothesis.
, and is the probability of not rejecting a false null hypothesis.
(True/False)
4.9/5  (27)
(27)
If a hypothesis is rejected at the 0.05 level of significance, what can be deduced from that?
(Multiple Choice)
4.8/5  (38)
(38)
A government testing agency studies aspirin capsules to see if they contain less medication than advertised. Suppose the testing agent concludes the capsules contain a mean amount below the advertised level when in fact the advertised level is the true mean. Which type of error, if any, did the testing agency commit?
(Multiple Choice)
4.8/5  (41)
(41)
In estimating the difference between two population means, the following summary statistics were found: 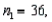
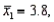
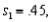
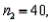
 and
and  Based on these results, the point estimate of
Based on these results, the point estimate of 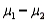 is 0.70.
is 0.70.
(True/False)
4.8/5  (32)
(32)
In testing 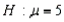 vs.
vs. 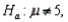 which of the following would happen if the sample size were increased?
which of the following would happen if the sample size were increased?
(Multiple Choice)
4.9/5  (39)
(39)
If a null hypothesis about the population proportion p is rejected at the 0.10 level of significance, it must be rejected at the 0.05 level.
(True/False)
4.8/5  (29)
(29)
Showing 81 - 100 of 154
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)