Exam 12: Experimental Design and Analysis of Variance

Consider the following partial analysis of variance table from a randomized block design with 6 blocks and 4 treatments. 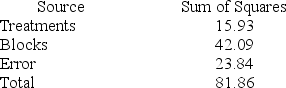What is the calculated F statistic for blocks?

If sample mean plots look essentially parallel, we can intuitively conclude that there is an interaction between the two factors.

________ simultaneous confidence intervals test all of the pairwise differences between means, respectively, while controlling the overall Type I error.

In a one-way analysis of variance with three treatments, each with five measurements, in which a completely randomized design is used, compute the F statistic where the sum of squares treatment is 17.0493 and the sum of squares error is 8.028.

Different levels of a factor are called ________.

Consider the randomized block design with 4 blocks and 3 treatments given above. What is the block sum of squares?

In one-way ANOVA, as the between-treatment variation decreases, the probability of rejecting the null hypothesis increases.

Consider the randomized block design with 4 blocks and 3 treatments given above. What is the treatment sum of squares?

Consider the following partial analysis of variance table from a randomized block design with 6 blocks and 4 treatments. 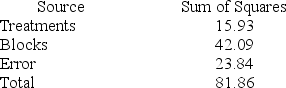Determine the degrees of freedom for treatments.

Consider the following partial analysis of variance table from a randomized block design with 6 blocks and 4 treatments. Test H₀: There is no difference between treatment effects at α = .05.

In a one-way analysis of variance with three treatments, each with five measurements, in which a completely randomized design is used, what are the degrees of freedom for treatments?

The F test for testing the difference between means is equal to the ratio of Mean Square ________ over Mean Square ________.

Consider the following partial analysis of variance table from a randomized block design with 10 blocks and 6 treatments. What is the calculated F statistic for blocks?

Suppose you are a researcher investigating the annual sales differences among five categories of businesses. The study looks at 55 companies equally divided among categories A, B, C, D, and E. 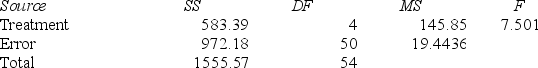Is there a significant difference in the annual sales of the five business categories at α = .05? Do you reject H₀?

ANOVA table 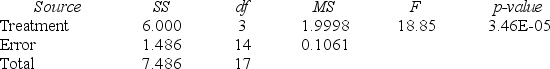Post hoc analysis Tukey simultaneous comparison t-values The Excel/MegaStat output given above summarizes the results of a one-way analysis of variance in an attempt to compare the performance characteristics of four brands of vacuum cleaners. The response variable is the amount of time it takes to clean a specific size room with a specific amount of dirt. How many total observations were there in this experiment?

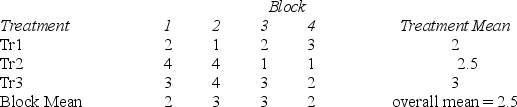Consider the randomized block design with 4 blocks and 3 treatments given above. What is the mean square error?

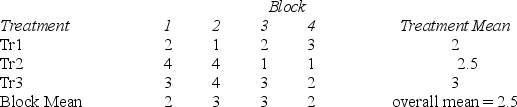Consider the randomized block design with 4 blocks and 3 treatments given above. Find the Tukey simultaneous 95 percent confidence interval for the difference between the means of block 2 and block 4.

A company that fills one-gallon containers of water has four machines. The quality control manager needs to determine whether the average fill for these machines is the same. For a sample of 19 one-gallon containers, we have the following data of fill measures (x) in quarts. Machine 1 Machine 2 Machine 3 Machine 4 N 4 6 5 4 (3 - 2) ± 4.53 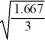= (-2.377, 4.377) 4.03 4.0017 3.974 4.005 S 0.0183 0.0117 0.0182 0.0129 And the following partial ANOVA table. 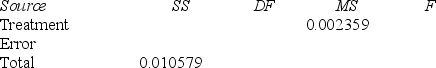Is there a significant difference in the fill amounts of the four machines at α = .05 to reject the null hypothesis?

Consider the following partial analysis of variance table from a randomized block design with 6 blocks and 4 treatments. 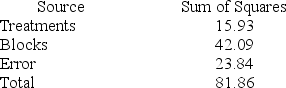Calculate the degrees of freedom for blocks.

In the randomized block ANOVA, the sum of squares for factor 1 equals