Exam 7: Random Variables and Discrete Probability Distributions

Mobile Phones Sales After analyzing sales data, the owner of a Mobile Phone store produced the following joint probability distribution of the number of iPhones (X) and Blackberries (Y) sold daily. 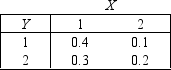-{Mobile Phones Sales Narrative} Compute the variance of the number of Blackberries sold daily.

Online Bankers An official from the securities commission estimates that 75% of all online bankers have profited from the use of insider information.Assume that 15 online bankers are selected at random from the commission's registry. -{Online Bankers Narrative} Find the probability that at least 6 have profited from insider information.

Number of Hamsters The joint probability distribution of X and Y is shown in the accompanying table, where X denotes the number of hamsters that Quinn may have next year, and Y denotes the number of hamsters that her boyfriend, Jason, may have when she moves in with him next year. 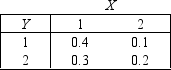-{Number of Hamsters Narrative} Are X and Y independent? Explain.

The following information regarding a portfolio of two stocks are given: w₁ = .25, w₂ = .75, E(R₁) = .08, and E(R₂) = .15.Which of the following regarding the portfolio expected return, E(R_p), is correct?

Sports Fans Suppose that past history shows that 5% of college students are sports fans.A sample of 10 students is to be selected. -{Sports Fans Narrative} Find the probability that more than 1 student is a sports fan.

Number of Birds Alana and Eva are sisters.Let X denote the number of birds that Alana may have in the next two years, and let Y denote the number of birds Eva may have during the same period.The marginal probability distributions of X and Y are shown below. -{Number of Birds Narrative} Calculate V(X + Y) directly by using the probability distribution of X + Y.

Let X be a binomial random variable with n = 25 and p = 0.01. a. Use the binomial table to find P(X = 0), P(X = 1), and P(X = 2). b. Find the variance and standard deviation of X.

Which of the following cannot have a Poisson distribution?

Post office The number of arrivals at a local post office between 3:00 and 5:00 P.M.has a Poisson distribution with a mean of 12. -{Post Office Narrative} Find the probability that the number of arrivals between 3:30 and 4:00 P.M.is at least 10.

The expected value of the sum of two random variables X and Y is equal to the ____________________ of the expected value of X and the expected value of Y.

If X is a binomial random variable with n = 25, and p = 0.25, then P(X = 25) = 1.0.

If X has a binomial distribution with n = 4 and p = 0.3, find P(X > 1).

Suppose that the number of buses arriving at a Depot per minute is a Poisson process.If the average number of buses arriving per minute is 3, what is the probability that exactly 6 buses arrive in the next minute?

Post office The number of arrivals at a local post office between 3:00 and 5:00 P.M.has a Poisson distribution with a mean of 12. -{{Post Office Narrative} Find the probability that the number of arrivals between 4:00 and 5:00 P.M.is exactly two.