Exam 3: Linear Programming: Sensitivity Analysis and Interpretation of Solution
Exam 1: Introduction63 Questions
Exam 2: An Introduction to Linear Programming66 Questions
Exam 3: Linear Programming: Sensitivity Analysis and Interpretation of Solution56 Questions
Exam 4: Linear Programming Applications in Marketing, Finance, and Operations Management63 Questions
Exam 5: Advanced Linear Programming Applications46 Questions
Exam 6: Distribution and Network Models70 Questions
Exam 7: Integer Linear Programming61 Questions
Exam 8: Nonlinear Optimization Models51 Questions
Exam 9: Project Scheduling: Pertcpm59 Questions
Exam 10: Inventory Models65 Questions
Exam 11: Waiting Line Models68 Questions
Exam 12: Simulation62 Questions
Exam 13: Decision Analysis97 Questions
Exam 14: Multicriteria Decisions50 Questions
Exam 15: Time Series Analysis and Forecasting63 Questions
Exam 16: Markov Processes49 Questions
Exam 17: Linear Programming: Simplex Method51 Questions
Exam 18: Simplex-Based Sensitivity Analysis and Duality35 Questions
Exam 19: Solution Procedures for Transportation and Assignment Problems44 Questions
Exam 20: Minimal Spanning Tree19 Questions
Exam 21: Dynamic Programming38 Questions
Select questions type
A section of output from The Management Scientist is shown here. 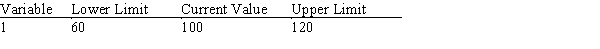 What will happen to the solution if the objective function coefficient for variable 1 decreases by 20?
What will happen to the solution if the objective function coefficient for variable 1 decreases by 20?
(Multiple Choice)
4.7/5  (38)
(38)
How would sensitivity analysis of a linear program be undertaken if one wishes to consider simultaneous changes for
both the right-hand-side values and objective function.
(Essay)
4.8/5  (28)
(28)
Portions of a Management Scientist output are shown below. Use what you know about the solution of linear programs to fill in the ten blanks.
LINEAR PROGRAMMING PROBLEM
MAX 12X1+9X2+7X3
S.T.
1) 3X1+5X2+4X3<150
2) 2X1+1X2+1X3<64
3) 1X1+2X2+1X3<80
4) 2X1+4X2+3X3>116
OPTIMAL SOLUTION
Objective Function Value = 336.000 
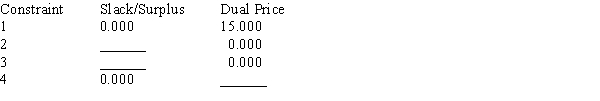 OBJECTIVE COEFFICIENT RANGES
OBJECTIVE COEFFICIENT RANGES  RIGHT HAND SIDE RANGES
RIGHT HAND SIDE RANGES 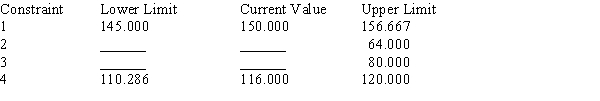
(Essay)
4.8/5  (37)
(37)
The dual price measures, per unit increase in the right hand side of the constraint,
(Multiple Choice)
5.0/5  (43)
(43)
The 100 percent rule can be applied to changes in both objective function coefficients and right-hand sides at the same time.
(True/False)
4.9/5  (38)
(38)
The dual price for a percentage constraint provides a direct answer to questions about the effect of increases or decreases in that percentage.
(True/False)
4.8/5  (37)
(37)
A negative dual price indicates that increasing the right-hand side of the associated constraint would be detrimental to the objective.
(True/False)
4.9/5  (38)
(38)
Eight of the entries have been deleted from the LINDO output that follows. Use what you know about linear programming to find values for the blanks.
MIN 6 X1 + 7.5 X2 + 10 X3
SUBJECT TO
2) 25 X1 + 35 X2 + 30 X3 >= 2400
3) 2 X1 + 4 X2 + 8 X3 >= 400
END
LP OPTIMUM FOUND AT STEP 2
OBJECTIVE FUNCTION VALUE
1) 612.50000 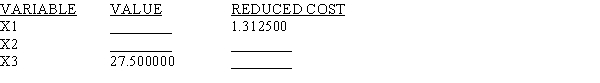
 NO. ITERATIONS= 2
RANGES IN WHICH THE BASIS IS UNCHANGED:
NO. ITERATIONS= 2
RANGES IN WHICH THE BASIS IS UNCHANGED: 

(Essay)
4.9/5  (35)
(35)
The cost that varies depending on the values of the decision variables is a
(Multiple Choice)
4.9/5  (32)
(32)
How can the interpretation of dual prices help provide an economic justification for new technology?
(Short Answer)
4.8/5  (32)
(32)
The optimal solution of the linear programming problem is at the intersection of constraints 1 and 2.
Max
2x1 + x2
s.t.
4x1 + 1x2 ≤ 400
4x1 + 3x2 ≤ 600
1x1 + 2x2 ≤ 300
x1 , x2 ≥ 0
a.Over what range can the coefficient of x1 vary before the current solution is no longer optimal?
b.Over what range can the coefficient of x2 vary before the current solution is no longer optimal?
c.Compute the dual prices for the three constraints.
(Essay)
4.8/5  (29)
(29)
Showing 41 - 56 of 56
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)