Exam 10: Conic Sections and Analytic Geometry
Exam 1: Equations and Inequalities425 Questions
Exam 2: Functions and Graphs359 Questions
Exam 3: Polynomial and Rational Functions532 Questions
Exam 4: Exponential and Logarithmic Functions270 Questions
Exam 5: Trigonometric Functions386 Questions
Exam 6: Analytic Trigonometry226 Questions
Exam 7: Additional Topics in Trigonometry264 Questions
Exam 8: Systems of Equations and Inequalities288 Questions
Exam 9: Matrices and Determinants152 Questions
Exam 10: Conic Sections and Analytic Geometry228 Questions
Exam 11: Sequences, Induction, and Probability304 Questions
Exam 12: Prerequisites: Fundamental Concepts of Algebra409 Questions
Select questions type
Use point plotting to graph the plane curve described by the given parametric equations.
-
(Multiple Choice)
4.7/5  (44)
(44)
Write the appropriate rotation formulas so that in a rotated system the equation has no x'y'-term.
-
(Multiple Choice)
4.9/5  (30)
(30)
Conic Sections in Polar Coordinates
1 Define Conics in Terms of a Focus and a Directrix
-
(Multiple Choice)
4.8/5  (35)
(35)
Convert the equation to the standard form for a hyperbola by completing the square on x and y.
-
(Multiple Choice)
4.8/5  (34)
(34)
Convert the equation to the standard form for a parabola by completing the square on x or y as appropriate.
-
(Multiple Choice)
4.8/5  (33)
(33)
Find the standard form of the equation of the ellipse satisfying the given conditions.
-Major axis horizontal with length 20 ; length of minor axis center
(Multiple Choice)
4.8/5  (32)
(32)
Find Parametric Equations for Functions
-The line segment starting at with and ending at with
(Multiple Choice)
4.8/5  (30)
(30)
Graph the Polar Equations of Conics
- Identify the directrix and vertices.
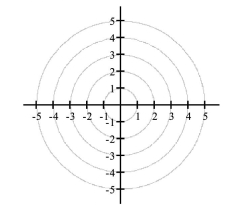
(Multiple Choice)
4.8/5  (30)
(30)
Graph the Polar Equations of Conics
- Identify the directrix and vertices.

(Multiple Choice)
4.9/5  (26)
(26)
Showing 101 - 120 of 228
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)