Exam 3: Graphs and Functions
Exam 1: Review of Basic Concepts637 Questions
Exam 2: Equations and Inequalities498 Questions
Exam 3: Graphs and Functions531 Questions
Exam 4: Polynomials and Rational Functions517 Questions
Exam 5: Inverse, Exponential, and Logarithmic Functions472 Questions
Exam 6: Systems and Matrices505 Questions
Exam 7: Arithmetic Sequence: Common Difference and First n Terms570 Questions
Select questions type
Determine whether the equation has a graph that is symmetric with respect to the y-axis, the x-axis, the origin, or none of
these.
-
(Multiple Choice)
4.8/5  (33)
(33)
Suppose the point (2, 4) is on the graph of y . Find a point on the graph of the given function.
-
(Multiple Choice)
4.8/5  (40)
(40)
Determine the largest open intervals of the domain over which the function is increasing, decreasing, and constant.
-
(Multiple Choice)
4.7/5  (36)
(36)
Write all linear equations in slope-intercept form.
-A company can make 9 satellite dishes for $92,300, while 16 satellite dishes cost $97,130. Find a linear equation that models the cost to produce x satellite dishes.
(Multiple Choice)
4.9/5  (28)
(28)
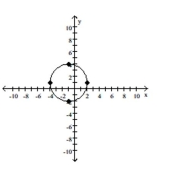
Use the graph to determine the equation of the circle in center-radius form.
-
(Multiple Choice)
4.8/5  (30)
(30)
For the pair of functions, find the indicated sum, difference, product, or quotient.
-
Find .
(Multiple Choice)
4.9/5  (27)
(27)
Describe how the graph of the equation relates to the graph of y .
-
![Describe how the graph of the equation relates to the graph of y y = \sqrt [ 3 ] { x } . - f(x)=(x-3)^{2}](https://storage.examlex.com/TB7516/11ecc545_8ae9_8e51_8347_1f15bfaca5a0_TB7516_11.jpg)
(Multiple Choice)
4.8/5  (36)
(36)
Describe how the graph of the equation relates to the graph of y .
-
![Describe how the graph of the equation relates to the graph of y y = \sqrt [ 3 ] { x } . - y=(x+3)^{2}+5](https://storage.examlex.com/TB7516/11ecc545_a7df_f446_8347_8d566b1a1f8e_TB7516_11.jpg)
(Multiple Choice)
4.8/5  (38)
(38)
Determine whether the three points are the vertices of a right triangle.
-
(True/False)
4.7/5  (33)
(33)
Showing 201 - 220 of 531
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)