Exam 6: Normal Probability Distributions
Exam 1: Introduction to Statistics85 Questions
Exam 2: Summarizing and Graphing Data82 Questions
Exam 3: Statistics for Describing, Exploring, and Comparing Data149 Questions
Exam 4: Probability170 Questions
Exam 5: Probability Distributions158 Questions
Exam 6: Normal Probability Distributions173 Questions
Exam 7: Estimates and Sample Sizes139 Questions
Exam 8: Hypothesis Testing130 Questions
Exam 9: Inferences From Two Samples105 Questions
Exam 10: Correlation and Regression129 Questions
Exam 11: Multinomial Experiments and Contingency Tables31 Questions
Exam 12: Analysis of Variance60 Questions
Exam 13: Nonparametric Statistics64 Questions
Exam 14: Statistical Process Control38 Questions
Select questions type
A population consists of these values: . Find the mean, , and standard deviation, , of the population. List all samples of size that are obtained without replacement. Find the population of all values of by finding the mean of each sample that you listed. Find the mean and standard deviation for the population of sample means by using the formulas:
Free
(Essay)
4.8/5  (36)
(36)
Correct Answer:
possible samples are: , population of values of and
Construct a normal probability plot of the given data. Use your plot to determine whether the data come from a normally distributed population
-The weekly incomes (in dollars)of a sample of 12 nurses working at a Los Angeles hospital are given below. 500 750 630 480 550 650 720 780 820 960 1200 770
Free
(Essay)
4.7/5  (41)
(41)
Correct Answer:
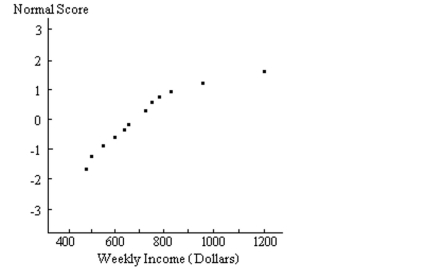 Because the pattern of the points is curved, it appears that the data do not come from a normally distributed
Because the pattern of the points is curved, it appears that the data do not come from a normally distributed
population.
The Precision Scientific Instrument Company manufactures thermometers that are supposed to give readings of 0°C at the freezing point of water. Tests on a large sample of these thermometers reveal that at the freezing point of water, some give readings below 0°C (denoted by negative numbers) and some give readings above 0°C (denoted by positive numbers). Assume that the mean reading is 0°C and the standard deviation of the readings is 1.00°C. Also assume that the frequency distribution of errors closely resembles the normal distribution. A thermometer is randomly selected and tested. Find the temperature reading corresponding to the given information.
-If 9% of the thermometers are rejected because they have readings that are too high, but all other thermometers are acceptable, find the temperature that separates the rejected thermometers from the others.
Free
(Multiple Choice)
4.8/5  (38)
(38)
Correct Answer:
A
Solve the problem.
-In a continuous uniform distribution,
Find the mean and standard deviation for a uniform distribution having a minimum of and a maximum of 6
(Multiple Choice)
4.9/5  (35)
(35)
Solve the problem.
-A study of the amount of time it takes a mechanic to rebuild the transmission for a 1992 Chevrolet Cavalier shows that the mean is 8.4 hours and the standard deviation is 1.8 hours. If 40 mechanics are randomly selected, find the probability that their mean rebuild time is less than 8.9 hours.
(Multiple Choice)
4.8/5  (23)
(23)
In a game of roulette, Jorge places 170 bets of $1 each on the number 3. A win pays off with odds 35:1 and on any one spin there is a 1/38 probability that 3 will be the winning number. Among the 170 bets, what is the minimum number of wins needed for Jorge to make a profit? Estimate the probability that Jorge will make a profit.
(Multiple Choice)
4.7/5  (39)
(39)
When sampling without replacement from a finite population of size N, the following formula is used to find the standard deviation of the population of sample means:
However, when the sample size , is smaller than of the population size, , the finite population correction , , can be omitted. Explain in your own words why this is reasonable. For , find the values of the finite population correction factor when the sample size is of the population, respectively. What do you notice?
(Essay)
4.7/5  (38)
(38)
Provide an appropriate response.
-Under what conditions are you allowed to use the normal distribution to approximate the binomial distribution? Under what conditions might you want to use the normal distribution to approximate the binomial as opposed to using the binomial probability formula, a table of binomial probabilities, or a computer?
(Essay)
4.8/5  (45)
(45)
Solve the problem.
-If a continuous uniform distribution has parameters of and , then the minimum is and the maximum is . For this distribution, find . Round your answer to three decimal places.
(Multiple Choice)
4.8/5  (30)
(30)
Assume that X has a normal distribution, and find the indicated probability.
-The mean is and the standard deviation is . Find the probability that is greater than .
(Multiple Choice)
4.8/5  (30)
(30)
The Precision Scientific Instrument Company manufactures thermometers that are supposed to give readings of 0°C at the freezing point of water. Tests on a large sample of these thermometers reveal that at the freezing point of water, some give readings below 0°C (denoted by negative numbers) and some give readings above 0°C (denoted by positive numbers). Assume that the mean reading is 0°C and the standard deviation of the readings is 1.00°C. Also assume that the frequency distribution of errors closely resembles the normal distribution. A thermometer is randomly selected and tested. Find the temperature reading corresponding to the given information.
-If 6.3% of the thermometers are rejected because they have readings that are too high and another 6.3% are rejected because they have readings that are too low, find the two readings that are cutoff values separating the rejected thermometers from the others.
(Multiple Choice)
4.7/5  (46)
(46)
Solve the problem.
-A study of the amount of time it takes a mechanic to rebuild the transmission for a 1992 Chevrolet Cavalier shows that the mean is 8.4 hours and the standard deviation is 1.8 hours. If 40 mechanics are randomly selected, find the probability that their mean rebuild time exceeds 8.7 hours.
(Multiple Choice)
4.9/5  (38)
(38)
A coin is tossed 20 times. A person, who claims to have extrasensory perception, is asked to predict the outcome of each flip in advance. She predicts correctly on 11 tosses. What is the probability of being correct 11 or more times by guessing? Does this probability seem to verify her claim?
(Multiple Choice)
4.8/5  (40)
(40)
Solve the problem.
-Scores on a test are normally distributed with a mean of and a standard deviation of . Find P81, which separates the bottom from the top .
(Multiple Choice)
4.8/5  (41)
(41)
A bank's loan officer rates applicants for credit. The ratings are normally distributed with a mean of 200 and a standard deviation of 50. If an applicant is randomly selected, find the probability of a rating that is between 200 and 275.
(Multiple Choice)
4.9/5  (30)
(30)
Use the continuity correction and describe the region of the normal curve that corresponds to the indicated binomial probability.
-The probability of more than 56 correct answers
(Multiple Choice)
4.8/5  (33)
(33)
Solve the problem.
-For women aged 18-24, systolic blood pressures (in mm Hg)are normally distributed with a mean of 114.8 and a standard deviation of 13.1. If 23 women aged 18-24 are randomly selected, find the probability that their mean systolic blood pressure is between 119 and 122.
(Multiple Choice)
4.9/5  (36)
(36)
Assume that the weight loss for the first month of a diet program varies between 6 pounds and 12 pounds, and is spread evenly over the range of possibilities, so that there is a uniform distribution. Find the probability of the given range of pounds lost
-Less than 9 pounds
(Multiple Choice)
4.8/5  (39)
(39)
A poll of 1700 randomly selected students in grades 6 through 8 was conducted and found that 37% enjoy playing sports. Would confidence in the results increase if the sample size were 3800 instead of 1700? Why or why not?
(Essay)
4.9/5  (41)
(41)
A normal probability plot is given below for a sample of scores on an aptitude test. Use the plot to assess the normality of scores on this test. Explain your reasoning. 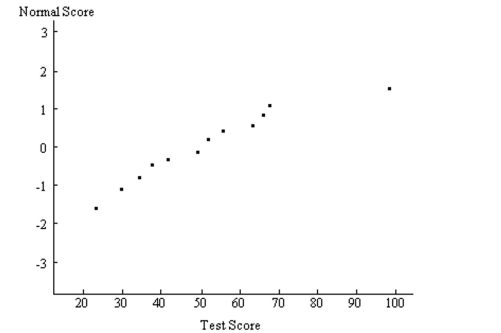
(Essay)
4.8/5  (30)
(30)
Showing 1 - 20 of 173
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)