Question 1

Which of the following is NOT a requirement for one-way ANOVA?

Accepted Answer

A)  The populations have distributions that are approximately normal. 
B)  The samples are simple random samples. 
C)  The samples are independent of each other. 
D)  The data is categorical data. 
A)  The populations have distributions that are approximately normal. 
B)  The samples are simple random samples. 
C)  The samples are independent of each other. 
D)  The data is categorical data.

Question 2

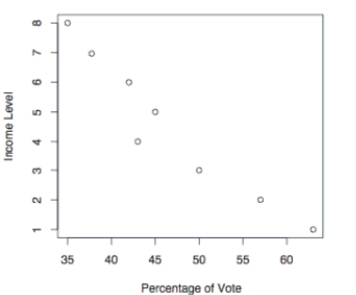
The following scatterplot shows the percentage of the vote a candidate received in the 2004 senatorial elections according to the voter's income level based on an exit poll of voters
Conducted by CNN. The income levels 1 -8 correspond to the following income classes: Use the election scatterplot to the find the critical values corresponding to a $0.01$ significance level used to test the null hypothesis of $\rho _ { z } = 0$.

Accepted Answer

A)  $- 0.881$ and $0.881$ 
B)  $- 0.881$ 
C)  $- 0.738$ and $0.738$ 
D)  $0.881$ 
A)  $- 0.881$ and $0.881$ 
B)  $- 0.881$ 
C)  $- 0.738$ and $0.738$ 
D)  $0.881$

Question 3

A control chart for attributes is to be constructed.
limits, a process which has been having a 5% rate of nonconforming items, or a process which
has been having a 10% of nonconforming items? Assume that both processes have the same
sample sizes. For a given sample size, would it be easier to detect a shift from 5% to 10% or a
shift from 10% to 15%? Explain your reasoning.

Accepted Answer

The process which has been having a 10%

Question 4

Are control charts based on actual behavior or on desired behavior? Give an example to
illustrate the difference between the two types of behavior.

Accepted Answer

Control charts are based on actual behav

Question 5

List the assumptions for testing hypotheses that three or more means are equivalent.

Accepted Answer

1) The populations have normal distribut

Question 6

Use the data in the given table and the corresponding Minitab display to test the hypothesis.
The following table shows the mileage for four different cars and three different brands of
gas. Assuming no effect from the interaction between car and brand of gas, test the claim that
the four cars have the same mean mileage. Use a 0.05 significance level. $\begin{array}{l|lll} 
& \text { Brand 1 } & \text { Brand 2 } & \text { Brand 3 } \
\hline \text { Car 1 } & 22.4 & 25.2 & 24.3 \
\text { Car 2 } & 19 & 18.6 & 19.8 \
\text { Car 3 } & 24.6 & 25 & 25.4 \
\text { Car 4 } & 23.5 & 23.6 & 24.1
\end{array}$

$\begin{array}{lrcrrc}
\text { Source } & D F & S S & M S & F & p \
\text { Car } & 3 & 61.249 & 20.416 & 39.033 & 0.000249 \
\text { Gas } & 2 & 2.222 & 1.111 & 2.124 & 0.200726 \
\text { Error } & 6 & 3.138 & 0.523 & & \
\text { Total } & 11 & 66.609 & & &
\end{array}$

Accepted Answer

@#LAT-DLM& : The cars have the same mean mileage.

Question 7

Use the Minitab display to test the indicated claim. A manager records the production output
of three employees who each work on three different machines for three different days. The
sample results are given below and the Minitab results follow. $\begin{array}{cccc}
&&&\text { Employee }\
&&\mathbf{A}&\mathbf{B}&\mathbf{C}\
&\text { I } & 31,34,32 & 29,23,22 & 21,20,24 \
\text { Machine } &\text { II } & 19,26,22 & 35,33,30 & 25,19,23 \
&\text { III } & 21,18,26 & 20,23,24 & 36,37,31
\end{array}$

$  \quad $$  \quad $$\text { ANALYSIS OF VARIANCE ITEMS }$

$\begin{array}{lrrr}
\text { SOURCE } & \text { DF } &{\text { SS }} & \text { MS } \
\text { MACHINE } & 2 & 1.19 & .59 \
\text { EMPLOYEE } & 2 & 5.85 & 2.93 \
\text { INTERACTION } & 4 & 710.81 & 177.70 \
\text { ERROR } & 18 & 160.00 & 8.89 \
\text { TOTAL } & 26 & 877.85 &
\end{array}$ Assume that the number of items produced is not affected by an interaction between
employee and machine. Using a 0.05 significance level, test the claim that the machine has no
effect on the number of items produced.

Accepted Answer

@#LAT-DLM& : There is no machine effect.
@#LAT-DLM& : There

Question 8

Describe a p chart and give an example. What does it attempt to monitor?

Accepted Answer

A p chart is a sequential plot of propor

Question 9

Construct an R chart and determine whether the process variation is within statistical control. $\text { Control Chart Constants }$
$  \quad $$  \quad $$  \quad $$  \quad $$  \quad $$ \bar{x} $ $  \quad $$  \quad $$  \quad $$  \quad $$  \quad $$  \quad $    $ \boldsymbol{s} $  $  \quad $$  \quad $$  \quad $$  \quad $$  \quad $$  \quad $ $ \boldsymbol{R} $
$\begin{array}{ccccccc}
\hline n & A_{2} & A_{3} & B_{3} & B_{4} & D_{3} & D_{4} \
\hline 2 & 1.880 & 2.659 & 0.000 & 3.267 & 0.000 & 3.267 \
3 & 1.023 & 1.954 & 0.000 & 2.568 & 0.000 & 2.574 \
4 & 0.729 & 1.628 & 0.000 & 2.266 & 0.000 & 2.282 \
5 & 0.577 & 1.427 & 0.000 & 2.089 & 0.000 & 2.114 \
6 & 0.483 & 1.287 & 0.030 & 1.970 & 0.000 & 2.004 \
7 & 0.419 & 1.182 & 0.118 & 1.882 & 0.076 & 1.924 \
8 & 0.373 & 1.099 & 0.185 & 1.815 & 0.136 & 1.864 \
9 & 0.337 & 1.032 & 0.239 & 1.761 & 0.184 & 1.816 \
10 & 0.308 & 0.975 & 0.284 & 1.716 & 0.223 & 1.777 \
\hline
\end{array}$
A machine that is supposed to produce ball bearings with a diameter of 7 millimeters yields the following data from a test of 5 ball bearings every 20 minutes.
 $  \quad $ $  \quad $ $  \quad $ $  \quad $ $  \quad $$\text { Ball Bearing Diameter }(\mathrm{mm} \text { ) }$
$\begin{array}{c|ccccc|c|c|}
\text { Sample } &&&&& & \bar{x} & \text { Range } \
\hline 1 & 6.3 & 6.8 & 6.9 & 6.8 & 6.9 & 6.74 & 0.6 \
2 & 6.3 & 6.6 & 6.6 & 6.3 & 7.0 & 6.56 & 0.7 \
3 & 6.8 & 6.7 & 7.0 & 6.5 & 7.0 & 6.80 & 0.5 \
4 & 7.0 & 6.7 & 6.7 & 6.8 & 6.8 & 6.80 & 0.3 \
5 & 6.8 & 6.8 & 6.6 & 6.5 & 6.4 & 6.62 & 0.4 \
6 & 6.8 & 6.7 & 6.6 & 6.3 & 6.9 & 6.66 & 0.6 \
7 & 7.3 & 7.3 & 7.4 & 7.4 & 7.0 & 7.28 & 0.4 \
8 & 7.2 & 7.0 & 7.2 & 6.9 & 7.1 & 7.08 & 0.3 \
9 & 7.3 & 7.6 & 7.1 & 7.4 & 7.6 & 7.40 & 0.5 \
10 & 7.2 & 7.6 & 7.5 & 7.6 & 7.1 & 7.40 & 0.5 \
11 & 7.2 & 7.2 & 7.4 & 7.0 & 7.0 & 7.16 & 0.4 \
12 &  \quad7.5  \quad& 7.4 & 7.4 & 7.6 & \quad 7.1  \quad& 7.40 & 0.5
\end{array}$

Accepted Answer

The process appears

Question 10

Test the claim that the samples come from populations with the same mean. Assume that the
populations are normally distributed with the same variance. At the 0.025 significance level,
test the claim that the four brands have the same mean if the following sample results have
been obtained. $$\begin{array} { c c c c } 
\text { Brand A } & \text { Brand B } & \text { Brand C } & \text { Brand D } \
\hline 17 & 18 & 21 & 22 \
20 & 18 & 24 & 25 \
21 & 23 & 25 & 27 \
22 & 25 & 26 & 29 \
21 & 26 & 29 & 35 \
& & 29 & 36 \
& & & 37
\end{array}$$

Accepted Answer

@#LAT-DLM& The means are not all equal. @#LAT-DLM&-value: @#LAT-DLM&.

Question 11

Use the data in the given table and the corresponding Minitab display to test the hypothesis?
The following table entries are test scores for males and females at different times of day.
Assuming no effect from the interaction between gender and test time, test the claim that time
of day does not affect test scores. Use a 0.05 significance level. $\begin{array}{l|cccc} 
& \text { 6 a.m. - 9 a.m. } & \text { 9 a.m. - 12 p.m. } & \text { 12 p.m. }-\mathbf{3} \text { p.m. } & \mathbf{3} \text { p.m. }-\mathbf{6} \text { p.m. } \
\hline \text { Male } & 87 & 89 & 92 & 85 \
\text { Female } & 72 & 84 & 94 & 89
\end{array}$

$\begin{array}{lcllcc}
\text { Source } & \text { DF } & \text { SS } & \text { MS } & \text { F } & \text { p } \
\text { Gender } & 1 & 24.5 & 24.5 & 0.6652 & 0.4745 \
\text { Time } & 3 & 183 & 61 & 1.6561 & 0.3444 \
\text { Error } & 3 & 110.5 & 36.83 & & \
\text { Total } & 7 & 318 & & &
\end{array}$

Accepted Answer

@#LAT-DLM& : There is no effect due to the time of

Question 12

Describe the runs test for randomness. What types of hypotheses is it used to test? Does the
runs test measure frequency? What is the underlying concept?

Accepted Answer

The runs test for randomness is a proced

Question 13

Provide the appropriate response. Describe the Wilcoxon signed-ranks test. What types of
hypotheses is it used to test? What assumptions are made for this test?

Accepted Answer

The Wilcoxon signed-ranks test is simila

Question 14

Suppose you are to test for equality of four different population means, with $H _ { 0 } : \mu _ { d } = \mu _ { B } = \mu _ { C } = \mu _ { D }$. Write the hypotheses for the paired tests. Use methods of probability to explain why the process of ANOVA has a higher degree of confidence than testing each of the pairs separately.

Accepted Answer

The six paired hypotheses are @#LAT-DLM&. Suppose

Question 15

Control charts are used to monitor changing characteristics of data over ____________.

Accepted Answer

A)  time 
B)  space 
C)  distance 
D)  speed 
A)  time 
B)  space 
C)  distance 
D)  speed

Question 16

Match the chart with its characteristic. $\begin{array}{ll}
  \text { A) Run chart  } & \text { 1) Each sample belongs to one of two  } \
 & \text { categories (such as defective or not defective). } \
  \text { B)  R chart  } & \text {2) It has no upper or lower control limits.  } \
  \text { C) }\bar{x}\text {  chart } & \text { 3) The centerline is }\overline{\bar{x}} \
  \text { D) p  chart  } & \text { 4) Monitors variation in a process. } \
\end{array}$

Accepted Answer

The answer of Match the chart with its characteristic. \(\begin{array}{ll}
...

Question 17

Use the runs test to determine whether the given sequence is random. Use a significance level
of 0.05. A sample of 30 clock radios is selected in sequence from an assembly line. Each
radio is examined and judged to be acceptable (A)or defective (D). The results are shown
below. Test for randomness. A A D A A A D A A D
A D A A A D A A A A
A A D D A A A A D A

Accepted Answer

@#LAT-DLM&. Test statistic: @#LAT-DLM&.

Question 18

Use the rank correlation coefficient to test for a correlation between the two variables. A
placement test is required for students desiring to take a finite mathematics course at a
university. The instructor of the course studies the relationship between students' placement
test score and final course score. A random sample of eight students yields the following data. $\begin{array}{cc}
\underline {\text { Placement Score }  } & \underline { \text { Final Course Score }} \
38 & 63 \
90 & 41 \
95 & 54 \
51 & 32 \
86 & 93 \
74 & 60 \
60 & 61 \
57 & 89
\end{array}$ Compute the rank correlation coefficient, rs, of the data and test the claim of correlation
between placement score and final course score. Use a significance level of 0.05.

Accepted Answer

@#LAT-DLM&. Critical values: @#LAT-DLM&.
Fail to r

Question 19

The test statistic for one-way ANOVA is equal to _________________.

Accepted Answer

A)  $\frac { \text { Variance within samples } } { \text { Variance between samples } }$ 
B)  $\frac { \text { Variance between samples } } { \text { Variance within samples } }$ 
C)  $\frac { \text { Mean within samples } } { \text { Mean between samples } }$ 
D)  $\frac { \text { Variance within populations } } { \text { Variance between populations } }$ 
A)  $\frac { \text { Variance within samples } } { \text { Variance between samples } }$ 
B)  $\frac { \text { Variance between samples } } { \text { Variance within samples } }$ 
C)  $\frac { \text { Mean within samples } } { \text { Mean between samples } }$ 
D)  $\frac { \text { Variance within populations } } { \text { Variance between populations } }$

Question 20

Fill in the missing entries in the following partially completed one -way ANOVA table. $\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & & & 11.16 \
\hline \text { Error } & & 13.72 & 0.686 & \
\hline \text { Total } & & & &
\end{array}$

Accepted Answer

A) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 22.97 & 7.66 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 36.69 & &
\end{array}$ 
B) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 2.55 & 7.66 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 16.27 & &
\end{array}$ 
C) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 48.80 & 16.27 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 62.52 & &
\end{array}$ 
D) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 0.184 & 0.061 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 13.90 & &
\end{array}$ 
A) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 22.97 & 7.66 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 36.69 & &
\end{array}$ 
B) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 2.55 & 7.66 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 16.27 & &
\end{array}$ 
C) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 48.80 & 16.27 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 62.52 & &
\end{array}$ 
D) 
$\begin{array}{l|c|c|c|c}
\text { Source } & d f & S S & M S=S S / d f & F \text {-statistic } \
\hline \text { Treatment } & 3 & 0.184 & 0.061 & 11.16 \
\hline \text { Error } & 20 & 13.72 & 0.686 & \
\hline \text { Total } & 23 & 13.90 & &
\end{array}$

Which of the following is NOT a requirement for one-way ANOVA?

Are control charts based on actual behavior or on desired behavior? Give an example to illustrate the difference between the two types of behavior.

List the assumptions for testing hypotheses that three or more means are equivalent.

Describe a p chart and give an example. What does it attempt to monitor?

Describe the runs test for randomness. What types of hypotheses is it used to test? Does the runs test measure frequency? What is the underlying concept?

Provide the appropriate response. Describe the Wilcoxon signed-ranks test. What types of hypotheses is it used to test? What assumptions are made for this test?

Control charts are used to monitor changing characteristics of data over ____________.

Match the chart with its characteristic. A) Run chart 1) Each sample belongs to one of two categories (such as defective or not defective). B) R chart 2) It has no upper or lower control limits. C) chart 3) The centerline is D) p chart 4) Monitors variation in a process.

The test statistic for one-way ANOVA is equal to _________________.

Fill in the missing entries in the following partially completed one -way ANOVA table. Source df SS MS=SS/df F -statistic Treatment 3 11.16 Error 13.72 0.686 Total

Introduction to Statistics

Exploring Data With Tables and Graphs

Describing, Exploring, and Comparing Data

Probability

Discrete Probability Distributions

Normal Probability Distributions

Estimating Parameters and Determining Sample Sizes

Hypothesis Testing

Inferences From Two Samples

Correlation and Regression

Chi-Square and Analysis of Variance

Filters

Exam 12: Control Charts and Process Monitoring

Which of the following is NOT a requirement for one-way ANOVA?

Are control charts based on actual behavior or on desired behavior? Give an example to illustrate the difference between the two types of behavior.

List the assumptions for testing hypotheses that three or more means are equivalent.

Describe a p chart and give an example. What does it attempt to monitor?

Describe the runs test for randomness. What types of hypotheses is it used to test? Does the runs test measure frequency? What is the underlying concept?

Provide the appropriate response. Describe the Wilcoxon signed-ranks test. What types of hypotheses is it used to test? What assumptions are made for this test?

Control charts are used to monitor changing characteristics of data over ____________.

Match the chart with its characteristic. A) Run chart 1) Each sample belongs to one of two categories (such as defective or not defective). B) R chart 2) It has no upper or lower control limits. C) chart 3) The centerline is D) p chart 4) Monitors variation in a process.

The test statistic for one-way ANOVA is equal to _________________.

Fill in the missing entries in the following partially completed one -way ANOVA table. Source df SS MS=SS/df F -statistic Treatment 3 11.16 Error 13.72 0.686 Total

Introduction to Statistics

Exploring Data With Tables and Graphs

Describing, Exploring, and Comparing Data

Probability

Discrete Probability Distributions

Normal Probability Distributions

Estimating Parameters and Determining Sample Sizes

Hypothesis Testing

Inferences From Two Samples

Correlation and Regression

Chi-Square and Analysis of Variance

Filters