Exam 15: Multiple Integrals
Exam 1: Functions and Models179 Questions
Exam 2: Limits and Derivatives139 Questions
Exam 3: Differentiation Rules160 Questions
Exam 4: Applications of Differentiation160 Questions
Exam 5: Integrals158 Questions
Exam 6: Applications of Integration157 Questions
Exam 7: Techniques of Integration160 Questions
Exam 8: Further Applications of Integration160 Questions
Exam 9: Differential Equations160 Questions
Exam 10: Parametric Equations and Polar Coordinates160 Questions
Exam 11: Infinite Sequences and Series159 Questions
Exam 12: Vectors and the Geometry of Space160 Questions
Exam 13: Vector Functions159 Questions
Exam 14: Partial Derivatives158 Questions
Exam 15: Multiple Integrals159 Questions
Exam 16: Vector Calculus159 Questions
Exam 17: Second-Order Differential Equations159 Questions
Select questions type
Find the mass of the lamina that occupies the region and has the given density function, if is bounded by the parabola and the line .
Select the correct answer.
Free
(Multiple Choice)
4.9/5  (37)
(37)
Correct Answer:
D
Evaluate the integral where and with respect to , and , in that order Select the correct answer.
Free
(Multiple Choice)
4.7/5  (43)
(43)
Correct Answer:
A
Evaluate the iterated integral by converting to polar coordinates. Round the answer to two decimal places.
Free
(Short Answer)
4.9/5  (26)
(26)
Correct Answer:
Use the transformation to evaluate the integral , where is the region bounded by the ellipse .
(Short Answer)
4.8/5  (37)
(37)
Find the mass of the solid bounded by the paraboloid and the plane if has constant density 3 .
(Short Answer)
4.9/5  (28)
(28)
Use polar coordinates to find the volume of the solid under the paraboloid and above the disk . Select the correct answer.
(Multiple Choice)
4.8/5  (27)
(27)
Use polar coordinates to find the volume of the solid bounded by the paraboloid and the plane .
Select the correct answer.
(Multiple Choice)
4.8/5  (35)
(35)
Find the mass and the moments of inertia , and and the radii of gyration and for the lamina occupying the region , where is the region bounded by the graphs of the equations , and , and having the mass density .
(Short Answer)
4.9/5  (34)
(34)
Use cylindrical coordinates to evaluate the triple integral
where is the solid that lies between the cylinders and above the -plane and below the plane .
(Short Answer)
4.8/5  (33)
(33)
Calculate the iterated integral. Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (38)
(38)
Use the given transformation to evaluate the integral.
, where is the region in the first quadrant bounded by the lines and the hyperbolas .
(Multiple Choice)
4.8/5  (31)
(31)
Find the area of the surface where is the part of the sphere that lies inside the cylinder .
(Short Answer)
4.8/5  (27)
(27)
Use cylindrical coordinates to evaluate
where is the region that lies inside the cylinder and between the planes and .
Round the answer to two decimal places.
(Short Answer)
4.9/5  (27)
(27)
Use a double integral to find the area of the region where is bounded by the circle .
Select the correct answer.
(Multiple Choice)
4.7/5  (26)
(26)
Use spherical coordinates.
Evaluate , where is the ball with center the origin and radius 4 .
(Multiple Choice)
4.9/5  (39)
(39)
Calculate the double integral. Round your answer to two decimal places.
(Short Answer)
5.0/5  (33)
(33)
Find the mass of a solid hemisphere of radius 5 if the mass density at any point on the solid is directly proportional to its distance from the base of the solid.
(Short Answer)
4.7/5  (38)
(38)
The sketch of the solid is given below. Given , write the inequalities that describe it.
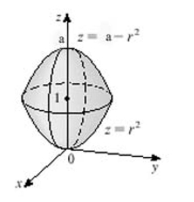
(Multiple Choice)
5.0/5  (36)
(36)
Use cylindrical coordinates to evaluate the triple integral
where is the solid that lies between the cylinders and above the -plane and below the plane .
Select the correct answer.
(Multiple Choice)
4.9/5  (36)
(36)
Showing 1 - 20 of 159
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)