Exam 10: Sequences, Series, and Power Series
Exam 1: Preliminaries127 Questions
Exam 2: Limits and Continuity92 Questions
Exam 3: Differentiation131 Questions
Exam 4: Transcendental Functions129 Questions
Exam 5: More Applications of Differentiation130 Questions
Exam 6: Integration117 Questions
Exam 7: Techniques of Integration118 Questions
Exam 8: Applications of Integration139 Questions
Exam 9: Conics, Parametric Curves, and Polar Curves114 Questions
Exam 10: Sequences, Series, and Power Series125 Questions
Exam 11: Vectors and Coordinate Geometry in 3-Space119 Questions
Exam 12: Vector Functions and Curves87 Questions
Exam 13: Partial Differentiation104 Questions
Exam 14: Applications of Partial Derivatives67 Questions
Exam 15: Multiple Integration105 Questions
Exam 16: Vector Fields90 Questions
Exam 17: Vector Calculus92 Questions
Exam 18: Differential Forms and Exterior Calculus76 Questions
Exam 19: Ordinary Differential Equations135 Questions
Select questions type
Find the Maclaurin series (binomial series) for 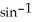 (x).
(x).
Free
(Multiple Choice)
4.9/5  (45)
(45)
Correct Answer:
C
The radius of convergence of the power series 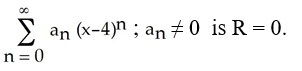 Which of the following statements is true?
Which of the following statements is true?
Free
(Multiple Choice)
4.9/5  (36)
(36)
Correct Answer:
E
Given f(x) = ln(3x + 1)
(a) Find Taylor's polynomial of degree n = 2 for f about c = 0
(b) Use part (a) to estimate the value of ln(1.3).
(c) Estimate the maximum absolute error involved in the approximation obtained in part (b) above.
(Essay)
4.9/5  (35)
(35)
Which of the three series (i)  (ii)
(ii) 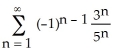 (iii)
(iii) 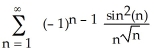 is absolutely convergent?
is absolutely convergent?
(Multiple Choice)
4.8/5  (44)
(44)
Which of the following descriptors apply to the sequence 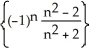 ?
(a) increasing (or ultimately increasing)
(b) decreasing (or ultimately decreasing)
(c) positive (or ultimately positive)
(d) negative (or ultimately negative)
(e) bounded below only
(f) bounded above only
(g) bounded
(h) unbounded above and below
(i) alternating
(j) divergent (but not to or - )
(k) divergent to
(l) divergent to -
(m) convergent
?
(a) increasing (or ultimately increasing)
(b) decreasing (or ultimately decreasing)
(c) positive (or ultimately positive)
(d) negative (or ultimately negative)
(e) bounded below only
(f) bounded above only
(g) bounded
(h) unbounded above and below
(i) alternating
(j) divergent (but not to or - )
(k) divergent to
(l) divergent to -
(m) convergent
(Multiple Choice)
4.9/5  (31)
(31)
For what values of x does the series ln x + 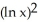 +
+ 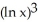 +
+  +... converge?
+... converge?
(Multiple Choice)
4.9/5  (36)
(36)
Use the integral test bounds to estimate the sum of the series 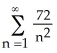 using the first three terms.
using the first three terms.
(Multiple Choice)
4.8/5  (35)
(35)
Find the centre, radius, and interval of convergence of the series 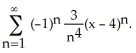
(Multiple Choice)
4.8/5  (38)
(38)
Showing 1 - 20 of 125
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)