Exam 7: Techniques of Integration

Find the Trapezoid Rule approximation for I = based on dividing [0, 1] into 5 equal subintervals. Quote your answer to 4 decimal places. Calculate the exact value of I and so determine the error in the approximation.

Let f(x) = 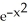and let I = 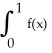dx. Given that 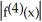$\le$ 12 for 0 $\le$ x $\le$ 1, what is the smallest value of n for which the Simpson's Rule approximation I ? S_2n will have error less than 0.0005 in absolute value? Hence, what is the value of I rounded to 3 decimal places?

Evaluate, if convergent, dx.

Evaluate the integral .

Given that (t) < 0 on the interval [a, b], what can be said about the relationship between the values of the integral I = dx, the Trapezoid Rule approximation for I, and the Midpoint Rule approximation for I?

Apply Simpson's Rule with n = 2 to approximate I = 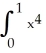dx. What is the actual error in this approximation? What does the Simpson's Rule error estimate give as an upper bound for the size of the error?

Evaluate, if convergent, . dx

Find the area between the curves y = 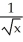and y = 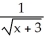to the right of x = 0 if the area is finite.

Evaluate the integral, dx.

Evaluate the integral

Find the area under the curve y = 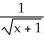and above the x-axis between x = -1 and x = 1.

Evaluate the integral dx.

What technique would you use to evaluate the integral I = 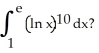Instead, try to evaluate it using Maple or another computer algebra system.

Find a reduction formula for I_n = 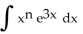and use it to evaluate I₄ = . 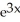dx

Evaluate, if convergent, cos x dx.

Evaluate the integral sin 4x dx.

Integrate dx.

Evaluate, if convergent,

Integrate

Evaluate the integral dx.