Exam 15: Functions of Several Variables
Exam 1: Functions226 Questions
Exam 2: Limits224 Questions
Exam 3: Derivatives367 Questions
Exam 4: Applications of the Derivative228 Questions
Exam 5: Integration166 Questions
Exam 6: Applications of Integration211 Questions
Exam 7: Logarithmic, Exponential, and Hyperbolic Functions85 Questions
Exam 8: Integration Techniques287 Questions
Exam 9: Differential Equations76 Questions
Exam 10: Sequences and Infinite Series173 Questions
Exam 11: Power Series103 Questions
Exam 12: Parametric and Polar Curves169 Questions
Exam 13: Vectors and the Geometry of Space131 Questions
Exam 14: Vector-Valued Functions83 Questions
Exam 15: Functions of Several Variables229 Questions
Exam 16: Multiple Integration299 Questions
Exam 17: Vector Calculus173 Questions
Select questions type
Find all the first order partial derivatives for the following function.
-f(x, y) = 
Free
(Multiple Choice)
4.9/5  (35)
(35)
Correct Answer:
A
Find all local extreme values of the given function and identify each as a local maximum, local minimum, or saddle point.
-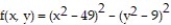
Free
(Multiple Choice)
4.9/5  (33)
(33)
Correct Answer:
D
Find all the second order partial derivatives of the given function.
-f(x, y) = 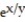
Free
(Multiple Choice)
4.9/5  (36)
(36)
Correct Answer:
B
Find the extreme values of the function subject to the given constraint.
-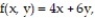

(Multiple Choice)
4.8/5  (42)
(42)
Find the extreme values of the function subject to the given constraint.
-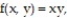
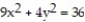
(Multiple Choice)
4.8/5  (28)
(28)
Find all the second order partial derivatives of the given function.
-f(x, y) = xy 
(Multiple Choice)
4.9/5  (40)
(40)
Find the derivative of the function at the given point in the direction of A.
-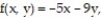
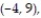 A = 4i- 3j
A = 4i- 3j
(Multiple Choice)
4.7/5  (45)
(45)
Write a chain rule formula for the following derivative.
- for z = f(r, s); r = g(t), s = h(t)
for z = f(r, s); r = g(t), s = h(t)
(Multiple Choice)
4.8/5  (34)
(34)
Use implicit differentiation to find the specified derivative at the given point.
-Find  at the point (2, 1) for ln x + x
at the point (2, 1) for ln x + x 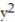 + ln y = 0.
+ ln y = 0.
(Multiple Choice)
4.8/5  (36)
(36)
Find all the first order partial derivatives for the following function.
-f(x, y) = ln 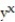
(Multiple Choice)
4.8/5  (32)
(32)
Solve the problem.
-Find the point on the sphere 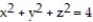 that is farthest from the point
that is farthest from the point 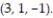
(Multiple Choice)
4.8/5  (29)
(29)
Solve the problem.
-A rectangular box with square base and no top is to have a volume of 32  . What is the least amount of material required?
. What is the least amount of material required?
(Multiple Choice)
4.8/5  (30)
(30)
Find two paths of approach from which one can conclude that the function has no limit as (x, y) approaches (0, 0).
-f(x, y) = 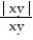
(Short Answer)
4.9/5  (32)
(32)
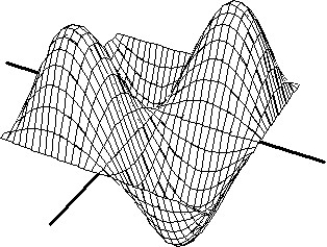
Match the surface show below to the graph of its level curves.
-
(Multiple Choice)
4.8/5  (34)
(34)
Write a chain rule formula for the following derivative.
-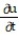 for u = f(v); v = h(s, t)
for u = f(v); v = h(s, t)
(Multiple Choice)
4.8/5  (38)
(38)
Solve the problem.
-Evaluate 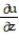 at (x, y, z) = ( 3, 4, 3) for the function u(p, q, r) =
at (x, y, z) = ( 3, 4, 3) for the function u(p, q, r) = 
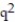 - r; p = y - z,
- r; p = y - z, 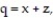
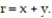
(Multiple Choice)
4.8/5  (40)
(40)
Find all the first order partial derivatives for the following function.
-f(x, y) = 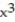 + 10
+ 10 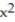 y - 2x
y - 2x 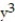
(Multiple Choice)
4.8/5  (34)
(34)
Provide an appropriate response.
-Find the direction in which the function is increasing most rapidly at the point 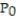 . f(x, y) = x
. f(x, y) = x  - ln(x),
- ln(x), 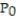 ( 2, 0)
( 2, 0)
(Multiple Choice)
4.8/5  (35)
(35)
Determine whether the function is a solution of the wave equation.
-w(x, t) = cos ( 4ct) sin ( 4x)
(True/False)
4.9/5  (28)
(28)
Find all the second order partial derivatives of the given function.
-f(x, y) = x ln (y - x)
(Multiple Choice)
4.7/5  (34)
(34)
Showing 1 - 20 of 229
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)