Exam 12: Multiple Regression

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: In a study of foreign holdings in Egyptian banks,the following sample regression results were obtained,based on 14 annual observations: = -3.25 + 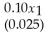- 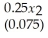+ 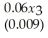,and R² = 0.92, Where the numbers in parentheses under the coefficient estimates are the estimated coefficient standard errors,and y = Year-end share of assets in Egyptian bank subsidiaries held by foreigners,as a percentage of total assets x₁ = Annual change,in billions of Egyptian pounds,in foreign direct investment in Egypt x₂ = Bank price-earnings ratio x₃ = Index of the exchange value of the Egyptian pounds -Find a 95% confidence intervals for β₃.

As you add irrelevant independent variables to a regression model,the coefficient of determination R² will increase.

In a multiple regression model,there are two independent variables and 25 observations.If SSE = 0.0625 and SST = 0.475,what is the value of the adjusted coefficient of determination?

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: An estimated linear model is given by = 12 - 3x₁ - 4x₂ + 7x₃. -When x₃ decreases by 2,what is the change in ?

What is the value of SST?

Multiple regression is a procedure for obtaining an equation that predicts a dependent or endogenous variable as a function of two or more independent or exogenous variables.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: The computer output for the multiple regression model,y = β₀ + β₁X₁ + β₂X₂ + ε is shown below.However,because of a printer malfunction some of the results are not shown.These are identified by asterisks. 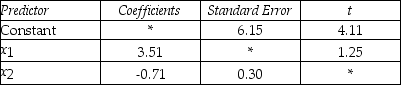S = * R-Sq = * ANALYSIS OF VARIANCE -What is the test statistic for testing H₀ : β₂ = 0 against H₁ : β₂ ≠ 0?

What is the 95% confidence interval for β₁?

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: The model y = β₀ + β₁X₁ + β₂X₂ + ε was fitted to a sample of 25 families in order to explain household milk consumption: where y = Milk consumption,in quarts,per week,x₁ = Weekly income,in hundreds of dollars,and x₂ = Family size.The least squares estimates of the regression parameters were b₀ = -0.03,b₁ = 0.05,and b₂ = 1.1,with coefficient standard errors 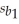= 0.02; 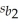= 0.38.The total sum of squares and the error sum of squares were found to be 165.8 and 66.32 respectively. -Test against the appropriate one-sided alternative,the null hypothesis that,for fixed family size,milk consumption does not depend linearly on income.Use α = 0.025.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A production manager is interested in modeling the determinants of the average cost of production.He creates the following model: Y = β₀ + β₁X₁ + β₂X₂ + β₃ + ε,where X₁ is the cost per unit of the primary input,and X₂ is the level of output.He examines the records over the past 45 production runs and obtains the following results: 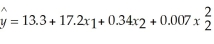. -What would the manager's null and alternative hypotheses for testing the significance of β₂ be?

In a multiple regression problem involving two independent variables X₁ and X₂,what does it mean if b₂ is computed to be -1.5?

Calculate the value of b₁.

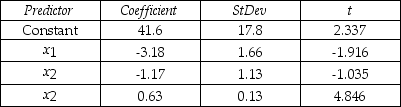
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A professor investigated some of the factors that affect an individual student's final grade in his course.He proposed the multiple regression model Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + ε ,where Y is the final mark (out of 100),X₁ is the number of lectures skipped,X₂ is the number of late assignments,and X₃ is the mid-term test mark (out of 100).The professor recorded the data for 50 randomly selected students.The computer output is shown below. The regression equation is = 41.6 - 3.18x₁ - 1.17x₂ + 0.63x₃. S = 13.74 R-Sq = 30.0% ANALYSIS OF VARIANCE 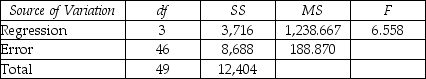-Does the data provide enough evidence to conclude that,at the 1% significance level,the final mark and the mid-term test mark show a positive linear relationship?

Which of the following is the value of ?

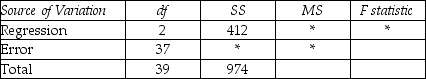
THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A regression analysis has produced the following partial analysis of variance table: Analysis of Variance 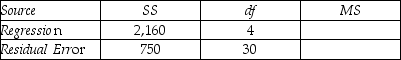-Compute the adjusted coefficient of determination.

In a multiple regression with two independent variables X₁ and X₂,the multiple standard error of the estimate measures the variation of the dependent variable Y about a predicted regression plane.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: In examining the determinants of income,data were collected regarding the characteristics of 45 adults,and the regression Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ +ε was used,where Y is the annual income (in thousands of dollars),X₁ is the person's age,X₂ is his/her years of education,and X₃ is a dummy variable = 1 if the adult is female. -If you get = 26.3 + 1.38x₁ + 2.98x₂ - 0.76x₃ when you run the regression,how would you interpret the coefficient on gender?

In calculating the standard error of the estimate,s_e = ,there are (n - K - 1)degrees of freedom,where n is the sample size and K is the number of independent variables in the model.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A professor investigated some of the factors that affect an individual student's final grade in his course.He proposed the multiple regression model Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + ε ,where Y is the final mark (out of 100),X₁ is the number of lectures skipped,X₂ is the number of late assignments,and X₃ is the mid-term test mark (out of 100).The professor recorded the data for 50 randomly selected students.The computer output is shown below. The regression equation is = 41.6 - 3.18x₁ - 1.17x₂ + 0.63x₃. S = 13.74 R-Sq = 30.0% ANALYSIS OF VARIANCE -Does the data provide enough evidence to conclude that,at the 5% significance level,the final mark and the number of skipped lectures are linearly related?

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A regression analysis has produced the following partial analysis of variance table: Analysis of Variance -Compute 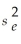and s_e.