Exam 12: Multiple Regression

If we run the regression Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + β₄X₄ + ε using a sample of 30 observations,what is the alternative hypothesis for the F-test of overall significance?

Most statistical softwares provide the p-value for testing each coefficient in the multiple regression model.In the case of the regression coefficient b₃,this p-value represents the probability that:

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A regression analysis has produced the following partial analysis of variance table: Analysis of Variance 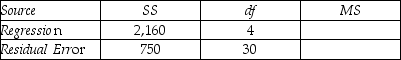-Set up the analysis of variance table for regression.

In regression analysis,the total variation in the dependent variable y,measured by 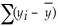²,can be decomposed into two parts: the explained variation,measured by SSR,and the unexplained variation,measured by SSE.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: An actuary wanted to develop a model to predict how long individuals will live.After consulting a number of physicians,she collected the age at death (y),the average number of hours of exercise per week (x₁),the cholesterol level (x₂),and the number of points that the individual's blood pressure exceeded the recommended value (x₃).A random sample of 40 individuals was selected.The computer output of the multiple regression model is shown below. THE REGRESSION EQUATION IS: = 55.8 + 1.79x₁ - 0.021x₂ - 0.016x₃ S = 9.47 R-Sq = 22.5% ANALYSIS OF VARIANCE -What is the coefficient of determination? What does this statistic tell you?

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A loan officer is interested in examining the determinants of the total dollar value of residential loans made during a month.The officer used Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + ε to model the relationship,where Y is the total dollar value of residential loans in a month (in millions of dollars),X₁ is the number of loans,X₂ is the interest rate,and X₃ is the dollar value of expenditures of the bank on advertising (in thousands of dollars).Using data from the past 24 months,she obtained the following results: = 5.7 + 0.189x₁ - 1.3x₂ + 0.08x₃, = 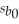3.2, 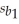= 0.03, 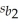= 0.062, 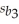= 0.17,R² = 0.46,and adjusted 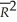= 0.41. -What should the null and alternative hypotheses be for β₂?

In a multiple regression problem involving 24 observations and three independent variables,the estimated regression equation is = 60 + 4.2x₁ + 1.8x₂ - 1.2x₃.For this model,SST = 800 and SSE = 245.The value of the F statistic for testing the significance of the model is 15.102.

In multiple regression analysis,b₀ and b_j are the sample statistics that estimate the population parameters β₀ and β_j (j = 1,2,…,K).

From the multiple coefficient of determination,we cannot detect the strength of the relationship between Y and any individual independent variable.

What is your conclusion regarding the hypothesis test for β₃?

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: The computer output for the multiple regression model,y = β₀ + β₁X₁ + β₂X₂ + ε is shown below.However,because of a printer malfunction some of the results are not shown.These are identified by asterisks. 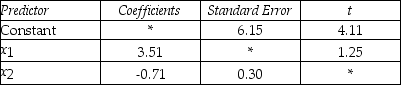S = * R-Sq = * ANALYSIS OF VARIANCE 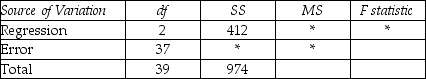-Calculate the standard error of the estimate.

In multiple regression analysis,it is possible that the multiple coefficient of determination will be equal to -0.50.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A real estate broker is interested in identifying the factors that determine the price of a house.She wants to run the following regression: Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + ε where Y = price of the house in $1,000s,X₁ = number of bedrooms,X₂ = square footage of living space,and X₃ = number of miles from the beach.Taking a sample of 30 houses,the broker runs a multiple regression and gets the following results: = 123.2 + 4.59x₁ + 0.125x₂ - 6.04x₃, = 103.2, 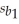= 2.13, 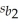= 0.062, 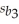= 4.17,R² = 0.47,and 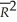= 0.45 (adjusted). -What should the null and alternative hypotheses be for β₃?

In a multiple regression model,there are six independent variables and 120 observations.What are the degrees of freedom associated with the mean square regression?

If a multiple regression analysis is based on five independent variables collected from a sample of 100 observations,what will be the value of the denominator in the calculation of the multiple standard error of estimate?

Determine SSR.

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: A loan officer is interested in examining the determinants of the total dollar value of residential loans made during a month.The officer used Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + ε to model the relationship,where Y is the total dollar value of residential loans in a month (in millions of dollars),X₁ is the number of loans,X₂ is the interest rate,and X₃ is the dollar value of expenditures of the bank on advertising (in thousands of dollars).Using data from the past 24 months,she obtained the following results: = 5.7 + 0.189x₁ - 1.3x₂ + 0.08x₃, = 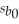3.2, 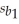= 0.03, 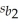= 0.062, 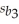= 0.17,R² = 0.46,and adjusted 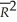= 0.41. -What should the null and alternative hypotheses be for β₁?

THE NEXT QUESTIONS ARE BASED ON THE FOLLOWING INFORMATION: Suppose you are interested in determining the factors that influence the time required to prepare a tax return,and developed the model: Y = β₀ + β₁X₁ + β₂X₂ + β₃X₃ + β₄X₄ + ε,where Y is the amount of time (in minutes),X₁ is the income of the individual (in thousands of dollars),X₂ is the age of the individual,X₃ is the number of people living in the household,and X₄ is a dummy variable that takes the value 1,if the individual owns his or her own home.After interviewing 40 accountants,you get the following results: = 17.2 + 3.8x₁ - 1.04x₂ + 2.15x₃ + 15.1x₄, = 5.3, 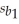= 0.13, 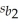= 0.33, 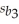= 1.51, 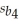= 4.7,SSR = 164.2,SSE = 200.7,and R² = 0.45. -Interpret the estimated regression coefficient b₁.

In multiple regression analysis involving 10 independent variables and 80 observations,the critical value of t for testing individual coefficients in the model will have:

In multiple regression analysis,a residual is the difference between the value of an independent variable X_i and its corresponding value of the dependent variable Y_i.