Exam 15: Nonparametric Methods: Nominal Level Hypothesis Tests

To compare the effect of weather on sales of soft drinks, a distributor surveyed people in two regions of the country and asked if they purchased soft drinks in the last month. The following are the results. The distributor would like to know if there is a difference in sales between the two regions. 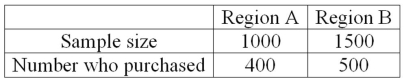Using the 1% level of significance, what is the critical value?

An electronics retailer believes that, at most, 40% of their cell phone inventory was sold during November. The retailer surveyed 80 dealers and found that 38% of the inventory was sold. Since 38% is less than 40%, is this difference of 2 percentage points sampling error or a significant difference? Test at the 0.05 level. The computed z = -0.37.

Six people have declared their intentions to run for a seat on the school board in the next local election. A poll is conducted during the campaign among 1,020 randomly sampled voters to determine if there is any clear preference among the voters. The responses are shown next. 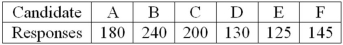What is the computed chi-square statistic?

A survey of property owners' opinions about a street-widening project was taken to determine if owners' opinions were related to the distance between their home and the street. A randomly selected sample of 100 property owners was contacted and the results are shown next. 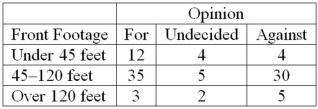What is the computed value of chi-square?

The chi-square test statistic used in a goodness-of-fit test has k - 1 degrees of freedom.

A distributor of personal computers has five locations in a city. In the year's first quarter, the sales in units were: 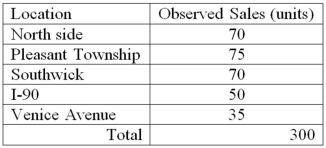For a goodness-of-fit test that sales were the same for all locations, what is the critical value at the 0.01 level of risk?

In a contingency table, the decision to reject the null hypothesis is based on a __________ test statistic.

The chi-square distribution is positively skewed.

If the computed value of a chi-square statistic is greater than the critical value, the null hypothesis is ________.

To compare the effect of weather on sales of soft drinks, a distributor surveyed people in two regions of the country and asked if they purchased soft drinks in the last month. The following are the results. The distributor would like to know if there is a difference in sales between the two regions. Test the hypothesis with a 0.01 significance level. What is our decision?

The number of trials and the population proportion are respectively represented by what symbols?

If we are testing for the difference between two population proportions, it is assumed that the two samples are large enough that the binomial distribution can be approximated by ______.

Suppose we test H₀: π₁ = π₂ at the 0.05 level of significance. If the z-test statistic is -1.07, what is our decision?

What is the decision regarding the differences between the observed and expected frequencies if the critical value of chi-square is 9.488 and the computed chi-square value is 6.079?

What does a significantly large chi-square statistic infer about the differences between the observed and expected frequencies in a goodness-of-fit test?

A random sample of 400 families who planned to buy a vacation residence revealed that 228 families want to buy a condominium in Florida. At the 0.01 significance level, test the statement that 55% of those families who plan to purchase a vacation residence in Florida want a condominium. To decide if the sample data support the 55%, state your decision in terms of the null hypothesis. Use a 0.01 level of significance.

If the decision is to reject the null hypothesis of no difference between two population proportions at the 5% level of significance, what are the alternative hypothesis and rejection region?

It is claimed that in a bushel of peaches, less than 10% are defective. A sample of 400 peaches is examined and 50 are found to be defective. What is the alternate hypothesis for a one-sided test?

Which chi-square distribution would be closest to a normal distribution?

A survey of property owners' opinions about a street-widening project was taken to determine if owners' opinions were related to the distance between their home and the street. A randomly selected sample of 100 property owners was contacted and the results are shown next. 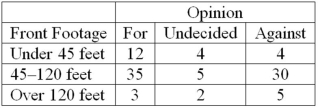What is the null hypothesis?