Exam 17: Regression Models With Dummy Variables
Exam 1: Statistics and Data102 Questions
Exam 2: Tabular and Graphical Methods123 Questions
Exam 3: Numerical Descriptive Measures152 Questions
Exam 4: Introduction to Probability148 Questions
Exam 5: Discrete Probability Distributions158 Questions
Exam 6: Continuous Probability Distributions143 Questions
Exam 7: Sampling and Sampling Distributions136 Questions
Exam 8: Interval Estimation131 Questions
Exam 9: Hypothesis Testing116 Questions
Exam 10: Statistical Inference Concerning Two Populations131 Questions
Exam 11: Statistical Inference Concerning Variance120 Questions
Exam 12: Chi-Square Tests120 Questions
Exam 13: Analysis of Variance120 Questions
Exam 14: Regression Analysis140 Questions
Exam 15: Inference With Regression Models125 Questions
Exam 16: Regression Models for Nonlinear Relationships118 Questions
Exam 17: Regression Models With Dummy Variables130 Questions
Exam 18: Time Series and Forecasting125 Questions
Exam 19: Returns, Index Numbers, and Inflation120 Questions
Exam 20: Nonparametric Tests120 Questions
Select questions type
An over-the-counter drug manufacturer wants to examine the effectiveness of a new drug in curing an illness most commonly found in older patients. Thirteen patients are given the new drug and 13 patients are given the old drug. To avoid bias in the experiment, they are not told which drug is given to them. To check how the effectiveness depends on the age of patients, the following data have been collected. 
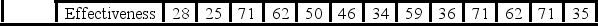

 The variables are Effectiveness = the response variable measured on a scale from 0 to 100,
Age = the age of a patient (in years),
Drug = a dummy variable that is 1 for the new drug and 0 for the old drug.
The regression model, Effectiveness = β0 + β1Age + β2Drug + β3Age × Drug, is estimated and the following results are obtained.
The variables are Effectiveness = the response variable measured on a scale from 0 to 100,
Age = the age of a patient (in years),
Drug = a dummy variable that is 1 for the new drug and 0 for the old drug.
The regression model, Effectiveness = β0 + β1Age + β2Drug + β3Age × Drug, is estimated and the following results are obtained. 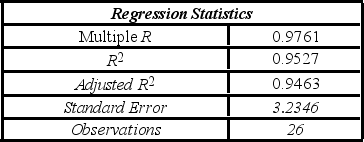
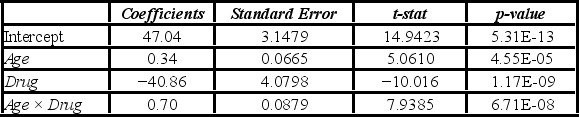 Which of the following is the percentage of variation in Effectiveness explained by the model?
Which of the following is the percentage of variation in Effectiveness explained by the model?
(Multiple Choice)
4.7/5  (24)
(24)
Consider the following regression model: Humidity = β0 + β1Temperature + β2Spring + β3Summer + β4Fall + β5Rain + ε, where the dummy variables Spring, Summer, and Fall represent the qualitative variable Season (spring, summer, fall, winter), and the dummy variable Rain is defined as Rain = 1 if rainy day, Rain = 0 otherwise.
Assuming the same temperature and precipitation condition, what is the difference between the predicted humidity for summer and fall days?
(Multiple Choice)
4.8/5  (27)
(27)
A medical researcher is interested in assessing the probability of some symptoms of coronary heart disease being present at a given age. For this reason, he uses the following data collected on 100 individuals. 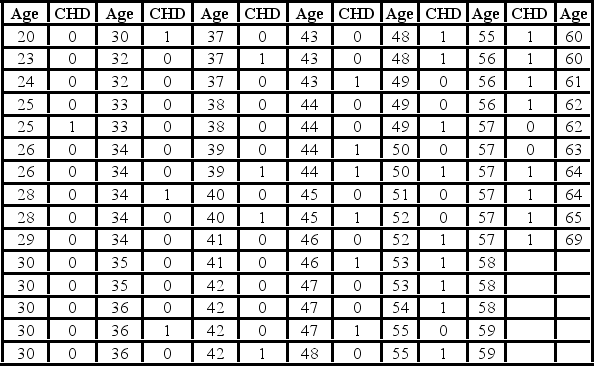 The response variable is CHD which is 1 if coronary heart disease is present and 0 otherwise. The following linear probability model and logit model have been estimated: Linear probability:
The response variable is CHD which is 1 if coronary heart disease is present and 0 otherwise. The following linear probability model and logit model have been estimated: Linear probability: 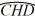 = - 0.53796 + 0.02181Age
Logit:
= - 0.53796 + 0.02181Age
Logit: 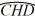 =
= 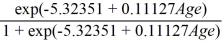 For the linear probability model, what is the range of values of Age for which
For the linear probability model, what is the range of values of Age for which 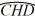 can be regarded as the estimated probability of coronary heart disease?
can be regarded as the estimated probability of coronary heart disease?
(Multiple Choice)
4.8/5  (39)
(39)
Consider the following regression model: Humidity = β0 + β1Temperature + β2Spring + β3Summer + β4Fall + β5Rain + ε, where the dummy variables Spring, Summer, and Fall represent the qualitative variable Season (spring, summer, fall, winter), and the dummy variable Rain is defined as Rain = 1 if rainy day, Rain = 0 otherwise.
What is the regression equation for the winter days?
(Multiple Choice)
4.9/5  (43)
(43)
A researcher wants to examine how the remaining balance on $100,000 loans taken 10 to 20 years ago depends on whether the loan was a prime or subprime loan. He collected a sample of 25 prime loans and 25 subprime loans and recorded the data in the following variables: Balance = the remaining amount of loan to be paid off (in $),
Time = the time elapsed from taking the loan,
Prime = a dummy variable assuming 1 for prime loans, and 0 for subprime loans.
The regression results obtained for the models:
Model A: Balance = β0 + β1Prime + ε
Model B: Balance = β0 + β1Time + β2Prime + β3Time × Prime + ε
Model C: Balance = β0 + β1Prime + β2Time × Prime + ε,
Are summarized in the following table. 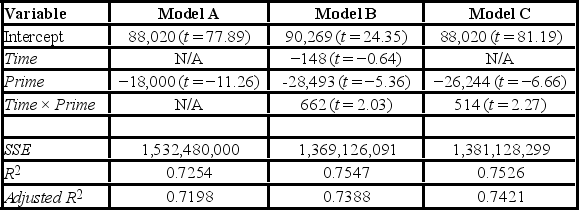 Note: The values of relevant test statistics are shown in parentheses below the estimated coefficients.
Using Model C, which of the following is the predicted balance on a $100,000 prime loan taken 15 years ago?
Note: The values of relevant test statistics are shown in parentheses below the estimated coefficients.
Using Model C, which of the following is the predicted balance on a $100,000 prime loan taken 15 years ago?
(Multiple Choice)
4.8/5  (35)
(35)
For the model y = β0 + β1x + β2d + β3xd + ε, in which d is a dummy variable, we can perform standard t tests for the individual significance of x, d, and xd.
(True/False)
4.8/5  (34)
(34)
A bank manager is interested in assigning a rating to the holders of credit cards issued by her bank. The rating is based on the probability of defaulting on credit cards and is as follows.  To estimate this probability, she decided to use the logit model,
P =
To estimate this probability, she decided to use the logit model,
P = 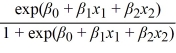 , where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained.
, where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained. 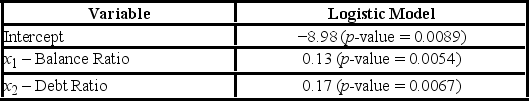 Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
Bob has a balance ratio of 10%, an annual income of $80,000, and $15,000 in total debt. Only applicants with excellent and good ratings qualify for a loan. Find the maximum amount of loan Bob can get if he is required to maintain his excellent or good rating after getting this amount.
Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
Bob has a balance ratio of 10%, an annual income of $80,000, and $15,000 in total debt. Only applicants with excellent and good ratings qualify for a loan. Find the maximum amount of loan Bob can get if he is required to maintain his excellent or good rating after getting this amount.
(Short Answer)
4.7/5  (44)
(44)
A bank manager is interested in assigning a rating to the holders of credit cards issued by her bank. The rating is based on the probability of defaulting on credit cards and is as follows.  To estimate this probability, she decided to use the logit model,
P =
To estimate this probability, she decided to use the logit model,
P = 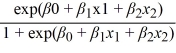 , where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained.
, where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained. 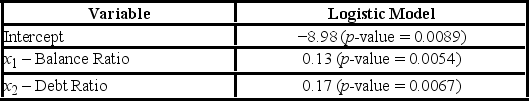 Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
Compute the predicted probability of default for a person whose balance ratio is 5% and debt ratio is 30%.
Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
Compute the predicted probability of default for a person whose balance ratio is 5% and debt ratio is 30%.
(Short Answer)
4.8/5  (35)
(35)
Including as many dummy variables as there are categories is referred to as the dummy variable ________.
(Short Answer)
4.9/5  (33)
(33)
The number of dummy variables representing a qualitative variable should be ________.
(Multiple Choice)
4.8/5  (37)
(37)
According to the Center for Disease Control and Prevention, life expectancy at age 65 in America is about 18.7 years. Medical researchers have argued that while excessive drinking is detrimental to health, drinking a little alcohol every day, especially wine, may be associated with an increase in life expectancy. Others have also linked longevity with income and gender. The data relating to the length of life after 65 (Life) were collected. Data also include: average income (in $1,000s) (Income), the average number of alcoholic drinks consumed per day (Drinks), and a dummy variable Woman. The following model was created: Life = β0 + β1Income + β2Drinks + β3Woman + ε. The Excel partial output corresponding to this model is shown below. 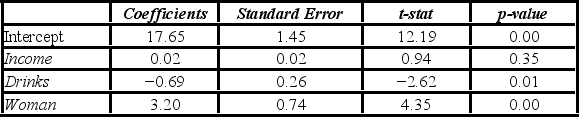 Which of the following is the estimated regression equation for the life expectancy?
Which of the following is the estimated regression equation for the life expectancy?
(Multiple Choice)
4.9/5  (29)
(29)
A realtor wants to predict and compare the prices of homes in three neighboring locations. She considers the following linear models:
Model A: Price = β0 + β1 Size + β2 Age + ε
Model B: Price = β0 + β1 Size + β3 Loc1 + β4 Loc2 + ε
Model C: Price = β0 + β1 Size + β2 Age + β3 Loc1 + β4 Loc2 + ε
where,
Price = the price of a home (in $1,000s)
Size = the square footage (in sq. feet)
Loc1 = a dummy variable taking on 1 for Location 1, and 0 otherwise
Loc2 = a dummy variable taking on 1 for Location 2, and 0 otherwise
After collecting data on 52 sales and applying regression, her findings were summarized in the following table. 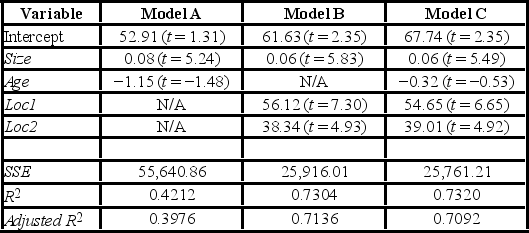 Note: The values of relevant test statistics are shown in parentheses below the estimated coefficients.
Using Model C, define the alternative hypothesis for testing the individual significance of Age.
Note: The values of relevant test statistics are shown in parentheses below the estimated coefficients.
Using Model C, define the alternative hypothesis for testing the individual significance of Age.
(Short Answer)
4.8/5  (35)
(35)
According to the Center for Disease Control and Prevention, life expectancy at age 65 in America is about 18.7 years. Medical researchers have argued that while excessive drinking is detrimental to health, drinking a little alcohol every day, especially wine, may be associated with an increase in life expectancy. Others have also linked longevity with income and gender. The data relating to the length of life after 65 (Life) were collected. Data were collected related to the length of life after 65 (Life) including the average income (in $1,000s) (Income), the average number of alcoholic drinks consumed per day (Drinks), and a dummy variable Woman which is 1 when the individual is a woman and 0 otherwise. The following model was created: Life = β0 + β1Income + β2Drinks + β3Woman + ε. Output corresponding to this model is shown below.  Which of the following are the competing hypotheses to determine if women live longer than men?
Which of the following are the competing hypotheses to determine if women live longer than men?
(Multiple Choice)
4.9/5  (35)
(35)
Consider the following regression model: Humidity = β0 + β1Temperature + β2Spring + β3Summer + β4Fall + β5Rain + ε, where the dummy variables Spring, Summer, and Fall represent the qualitative variable Season (spring, summer, fall, winter), and the dummy variable Rain is defined as Rain = 1 if rainy day, Rain = 0 otherwise.
Assuming the same temperature and precipitation condition, what is the difference between the predicted humidity for summer and winter days?
(Multiple Choice)
4.7/5  (37)
(37)
Consider the regression model y = β0 + β1x + β2d + β3xd + ε. If the dummy variable d changes from 0 to 1, the estimated changes in the intercept and the slope are b0 + b2 and b2, respectively.
(True/False)
4.8/5  (35)
(35)
An over-the-counter drug manufacturer wants to examine the effectiveness of a new drug in curing an illness most commonly found in older patients. Thirteen patients are given the new drug and 13 patients are given the old drug. To avoid bias in the experiment, they are not told which drug is given to them. To check how the effectiveness depends on the age of patients, the following data have been collected. 
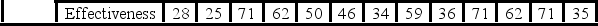

 The variables are Effectiveness = the response variable measured on a scale from 0 to 100,
Age = the age of a patient (in years),
Drug = a dummy variable that is 1 for the new drug and 0 for the old drug.
The regression model, Effectiveness = β0 + β1Age + β2Drug + β3Age × Drug, is estimated and the following results are obtained.
The variables are Effectiveness = the response variable measured on a scale from 0 to 100,
Age = the age of a patient (in years),
Drug = a dummy variable that is 1 for the new drug and 0 for the old drug.
The regression model, Effectiveness = β0 + β1Age + β2Drug + β3Age × Drug, is estimated and the following results are obtained. 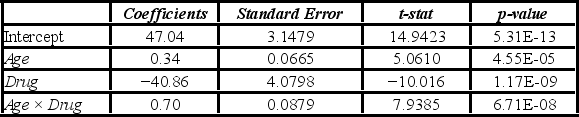 Which of the following is the predicted effectiveness of the new drug for a 62-year-old patient?
Which of the following is the predicted effectiveness of the new drug for a 62-year-old patient?
(Multiple Choice)
4.7/5  (25)
(25)
A researcher wants to examine how the remaining balance on $100,000 loans taken 10 to 20 years ago depends on whether the loan was a prime or subprime loan. He collected a sample of 25 prime loans and 25 subprime loans and recorded the data in the following variables: Balance = the remaining amount of loan to be paid off (in $),
Time = the time elapsed from taking the loan,
Prime = a dummy variable assuming 1 for prime loans, and 0 for subprime loans.
The regression results obtained for the models:
Model A: Balance = β0 + β1Prime + ε
Model B: Balance = β0 + β1Time + β2Prime + β3Time × Prime + ε
Model C: Balance = β0 + β1Prime + β2Time × Prime + ε,
Are summarized in the following table. 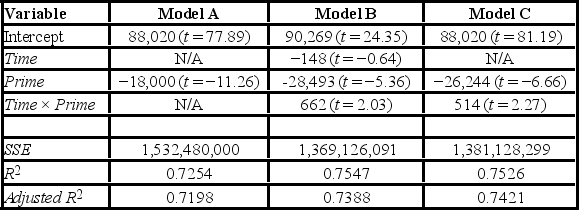 Note: The values of relevant test statistics are shown in parentheses below the estimated coefficients.
Using Model B, what is the null hypothesis for testing the joint significance of the variable Time and the interaction variable Time × Prime?
Note: The values of relevant test statistics are shown in parentheses below the estimated coefficients.
Using Model B, what is the null hypothesis for testing the joint significance of the variable Time and the interaction variable Time × Prime?
(Multiple Choice)
4.9/5  (40)
(40)
A bank manager is interested in assigning a rating to the holders of credit cards issued by her bank. The rating is based on the probability of defaulting on credit cards and is as follows.  To estimate this probability, she decided to use the logit model,
P =
To estimate this probability, she decided to use the logit model,
P = 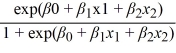 , where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained.
, where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained.  Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
Assuming the debt ratio 30%, compute the increase in the probability of defaulting when the balance ratio goes up from 5% to 15%.
Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
Assuming the debt ratio 30%, compute the increase in the probability of defaulting when the balance ratio goes up from 5% to 15%.
(Short Answer)
4.9/5  (37)
(37)
A bank manager is interested in assigning a rating to the holders of credit cards issued by her bank. The rating is based on the probability of defaulting on credit cards and is as follows.  To estimate this probability, she decided to use the logit model
P =
To estimate this probability, she decided to use the logit model
P = 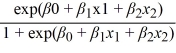 , where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained.
, where
y = a binary response variable which is 1 if the credit card is in default and 0 otherwise
x1 = the ratio of the credit card balance to the credit card limit (in %)
x2 = the ratio of the total debt to the annual income (in %)
The following output is obtained. 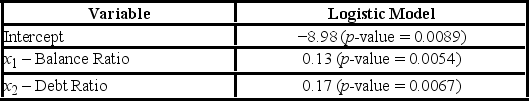 Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
What is the estimated logit model?
Note: The p-values of the corresponding tests are shown in parentheses below the estimated coefficients.
What is the estimated logit model?
(Essay)
4.8/5  (37)
(37)
Showing 41 - 60 of 130
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)