Exam 13: Simple Linear Regression Analysis
Exam 1: An Introduction to Business Statistics54 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Methods90 Questions
Exam 3: Descriptive Statistics: Numerical Methods149 Questions
Exam 4: Probability135 Questions
Exam 5: Discrete Random Variables128 Questions
Exam 6: Continuous Random Variables150 Questions
Exam 7: Sampling and Sampling Distributions116 Questions
Exam 8: Confidence Intervals144 Questions
Exam 9: Hypothesis Testing148 Questions
Exam 10: Statistical Inferences Based on Two Samples132 Questions
Exam 11: Experimental Design and Analysis of Variance115 Questions
Exam 12: Chi-Square Tests96 Questions
Exam 13: Simple Linear Regression Analysis148 Questions
Exam 14: Multiple Regression122 Questions
Exam 15: Model Building and Model Diagnostics102 Questions
Exam 16: Time Series Forecasting150 Questions
Exam 17: Process Improvement Using Control Charts122 Questions
Exam 18: Nonparametric Methods97 Questions
Exam 19: Decision Theory90 Questions
Select questions type
Consider the following partial computer output from a simple linear regression analysis.  S = 0.4862 R-Sq = ______ Analysis of Variance
S = 0.4862 R-Sq = ______ Analysis of Variance  What is the predicted value of y when x = 9.00?
What is the predicted value of y when x = 9.00?
(Multiple Choice)
4.9/5  (32)
(32)
A local tire dealer wants to predict the number of tires sold each month.He believes that the number of tires sold is a linear function of the amount of money invested in advertising.He randomly selects 6 months of data consisting of tire sales (in thousands of tires)and advertising expenditures (in thousands of dollars).Based on the data set with 6 observations,the simple linear regression model yielded the following results.  = 24
= 24 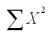 = 124
= 124 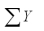 = 42
= 42 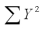 = 338
= 338 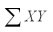 = 196 Determine the value of the F statistic.
= 196 Determine the value of the F statistic.
(Multiple Choice)
4.9/5  (32)
(32)
The strength of the relationship between two quantitative variables can be measured by:
(Multiple Choice)
4.8/5  (35)
(35)
When there is positive autocorrelation,over time,negative error terms are followed by positive error terms and positive error terms are followed by negative error terms.
(True/False)
4.8/5  (32)
(32)
For the same value X (independent variable),the confidence interval for the average value of Y (dependent variable)is __________________ the prediction interval for the individual value of Y.
(Multiple Choice)
4.8/5  (32)
(32)
A data set with 7 observations yielded the following.Use the simple linear regression model.  = 21.57
= 21.57 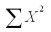 = 68.31
= 68.31 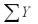 = 188.9
= 188.9  = 5,140.23
= 5,140.23 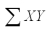 = 590.83 SSE = 1.06
Calculate the correlation coefficient.
= 590.83 SSE = 1.06
Calculate the correlation coefficient.
(Multiple Choice)
4.7/5  (41)
(41)
Consider the following partial computer output from a simple linear regression analysis.  S = 0.4862 R-Sq = ______ Analysis of Variance
S = 0.4862 R-Sq = ______ Analysis of Variance  What is the coefficient of determination?
What is the coefficient of determination?
(Multiple Choice)
4.7/5  (35)
(35)
In simple linear regression analysis,we assume that the variance of the independent variable (X)is equal to the variance of the dependent variable (Y).
(True/False)
4.8/5  (41)
(41)
When the constant variance assumption holds,a plot of the residual versus x:
(Multiple Choice)
4.8/5  (43)
(43)
Consider the following partial computer output from a simple linear regression analysis.  What is the estimated slope?
What is the estimated slope?
(Multiple Choice)
4.8/5  (37)
(37)
Consider the following partial computer output from a simple linear regression analysis.  S = 0.4862 R-Sq = ______ Analysis of Variance
S = 0.4862 R-Sq = ______ Analysis of Variance  Calculate the SSE
Calculate the SSE
(Multiple Choice)
4.8/5  (31)
(31)
An experiment was performed on a certain metal to determine if the strength is a function of heating time.The simple linear regression equation is 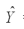 = 1 + 1X.The time is in minutes and the strength is measured in pounds per square inch. The 95% confidence interval for the slope is from .564 to 1.436.Can we reject 1 = 0?
= 1 + 1X.The time is in minutes and the strength is measured in pounds per square inch. The 95% confidence interval for the slope is from .564 to 1.436.Can we reject 1 = 0?
(Multiple Choice)
4.8/5  (37)
(37)
Use the following results obtained from a simple linear regression analysis with 12 observations. 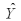 = 37.2895 - (1.2024)X r2 = .6744 sb = .2934
Test to determine if there is a significant negative relationship between the independent and dependent variable at = .05.Give the test statistic and the resulting conclusion.
= 37.2895 - (1.2024)X r2 = .6744 sb = .2934
Test to determine if there is a significant negative relationship between the independent and dependent variable at = .05.Give the test statistic and the resulting conclusion.
(Multiple Choice)
4.9/5  (35)
(35)
Consider the following partial computer output from a simple linear regression analysis.  S = 0.4862 R-Sq = ______ Analysis of Variance
S = 0.4862 R-Sq = ______ Analysis of Variance  Test H0:
Test H0:  1 = 0 versus Ha:
1 = 0 versus Ha: 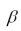 1 0 by setting
1 0 by setting 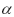 = .001.What do you conclude about the relationship between y and x?
= .001.What do you conclude about the relationship between y and x?
(Multiple Choice)
4.7/5  (30)
(30)
Regression Analysis 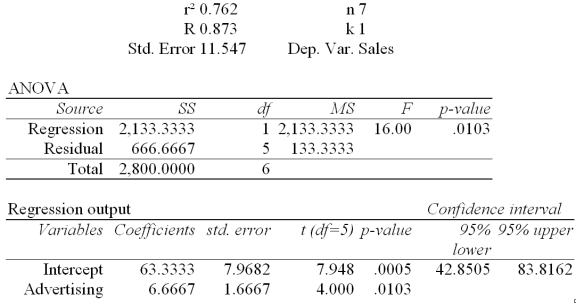 The local grocery store wants to predict the daily sales in dollars.The manager believes that the amount of newspaper advertising significantly affects the store sales.He randomly selects 7 days of data consisting of daily grocery store sales (in thousands of dollars)and advertising expenditures (in thousands of dollars).The Excel/Mega-Stat output given above summarizes the results of the regression model. What are the limits of the 95% confidence interval for the population slope?
The local grocery store wants to predict the daily sales in dollars.The manager believes that the amount of newspaper advertising significantly affects the store sales.He randomly selects 7 days of data consisting of daily grocery store sales (in thousands of dollars)and advertising expenditures (in thousands of dollars).The Excel/Mega-Stat output given above summarizes the results of the regression model. What are the limits of the 95% confidence interval for the population slope?
(Multiple Choice)
4.8/5  (37)
(37)
The following results were obtained from a simple regression analysis:  = 37.2895 - (1.2024)X r2= .6744 sb = .2934
When X (independent variable)is equal to zero,the estimated value of Y (dependent variable)is equal to:
= 37.2895 - (1.2024)X r2= .6744 sb = .2934
When X (independent variable)is equal to zero,the estimated value of Y (dependent variable)is equal to:
(Multiple Choice)
4.7/5  (36)
(36)
An experiment was performed on a certain metal to determine if the strength is a function of heating time.Results based on 10 metal sheets are given below.Use the simple linear regression model. 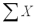 = 30
= 30 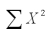 = 104
= 104  = 40
= 40 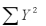 = 178
= 178 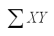 = 134 Find the t statistic and test H0: B1 0 vs.Ha: B1 > 0 at = .05.
= 134 Find the t statistic and test H0: B1 0 vs.Ha: B1 > 0 at = .05.
(Multiple Choice)
4.7/5  (36)
(36)
In simple regression analysis,if the correlation coefficient is a positive value,then
(Multiple Choice)
4.8/5  (32)
(32)
If r = -1,then we can conclude that there is a perfect relationship between X and Y.
(True/False)
4.7/5  (33)
(33)
A data set with 7 observations yielded the following.Use the simple linear regression model.  = 21.57
= 21.57 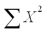 = 68.31
= 68.31 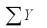 = 188.9
= 188.9 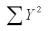 = 5,140.23
= 5,140.23  = 590.83 SSE = 1.06
Find the rejection point for the t statistic (
= 590.83 SSE = 1.06
Find the rejection point for the t statistic ( 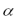 = .05).Test H0: 1 0 vs.Ha: 1 > 0.
= .05).Test H0: 1 0 vs.Ha: 1 > 0.
(Multiple Choice)
4.7/5  (41)
(41)
Showing 81 - 100 of 148
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)