Question 1

The ________ of a waiting line and the probability that the queue is empty add to one.

Accepted Answer

utilization

Question 2

A crew of mechanics at the Highway Department garage repair vehicles that break down at an average of λ = 7.5 vehicles per day (approximately Poisson in nature).The mechanic crew can service an average of μ = 10 vehicles per day with a repair time distribution that approximates an exponential distribution.
a.What is the utilization rate for this service system?
b.What is the average time before the facility can return a breakdown to service?
c.How much of that time is spent waiting for service?
d.How many vehicles are likely to be in the system at any one time?

Accepted Answer

(a)Utilization is ρ = 7.5 / 10 = .75 or 75%; (b)Ws = 1 / (10 - 7.5)= 1 / 2.5 = 0.4 days; (c)Wq = 7.5 / 10∗(10 - 7.5)= 0.3 days; (d) 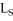  = 7.5 / (10 - 7.5)= 7.5 / 2.5 = 3 units.

Question 3

The sign at the bank that reads "Wait here for the first available teller" suggests the use of a ________ waiting line system.

Accepted Answer

A) dynamic-channel 
B) random-channel 
C) single channel 
D) multi-channel 
E) multiple line 
A) dynamic-channel 
B) random-channel 
C) single channel 
D) multi-channel 
E) multiple line D

Question 4

In queuing problems,arrival rates are generally described by the normal probability distribution.

Accepted Answer

A) True 
 B)False

Question 5

A bank office with five tellers,each with a separate line of customers,exhibits the characteristics of a multi-phase queuing system.

Accepted Answer

A) True 
 B)False

Question 6

A waiting-line system that meets the assumptions of M/M/1 has λ = 1,μ = 4.For this system,Po is ________ and utilization is ________.

Accepted Answer

A) 0.75;0.25 
B) 0.80;.20 
C) -3;-4 
D) 3;4 
E) none of these 
A) 0.75;0.25 
B) 0.80;.20 
C) -3;-4 
D) 3;4 
E) none of these

Question 7

Provide an example of a limited or finite population for a queue.

Accepted Answer

Answers will vary,but the text

Question 8

A university has only one technician in the repair station to care for the computers in the student labs.This system is most likely

Accepted Answer

A) a single channel,limited queue system. 
B) a single channel,limited population system. 
C) a multi-channel,limited queue system. 
D) a multi-channel,limited population system. 
E) a multi-channel,unlimited population system. 
A) a single channel,limited queue system. 
B) a single channel,limited population system. 
C) a multi-channel,limited queue system. 
D) a multi-channel,limited population system. 
E) a multi-channel,unlimited population system.

Question 9

Which of the following is not a common queuing situation?

Accepted Answer

A) grocery shoppers being served by checkout clerks 
B) commuters slowing or stopping at toll plazas to pay highway tolls 
C) machinery waiting to be repaired or maintained 
D) parcel delivery truck following its computer-generated route 
E) patients in a health clinic waiting to see one of several doctors 
A) grocery shoppers being served by checkout clerks 
B) commuters slowing or stopping at toll plazas to pay highway tolls 
C) machinery waiting to be repaired or maintained 
D) parcel delivery truck following its computer-generated route 
E) patients in a health clinic waiting to see one of several doctors

Question 10

A finite population waiting line model has an average service time T of 100 minutes and an average time between service requirements U of 400 minutes;the service factor X is

Accepted Answer

A) 0.20. 
B) 0.25. 
C) 4. 
D) 5. 
E) 300 minutes. 
A) 0.20. 
B) 0.25. 
C) 4. 
D) 5. 
E) 300 minutes.

Question 11

Describe the important operating characteristics of a queuing system.

Accepted Answer

The operating characteristics usually co

Question 12

Which one of the following is not a characteristic of a Model A or M/M/1 system?

Accepted Answer

A) exponential service time pattern 
B) single number of channels 
C) single number of phases 
D) Poisson arrival rate pattern 
E) limited population size 
A) exponential service time pattern 
B) single number of channels 
C) single number of phases 
D) Poisson arrival rate pattern 
E) limited population size

Question 13

A queuing model which follows the M/M/1 assumptions has λ = 2 and μ = 3.The average waiting time in the system is

Accepted Answer

A) 2/3. 
B) 1. 
C) 1.5. 
D) 2. 
E) 6. 
A) 2/3. 
B) 1. 
C) 1.5. 
D) 2. 
E) 6.

Question 14

A finite population waiting line model with a single server has an average service time T of 200 minutes and an average time between service requirements U of 300 minutes.Calculate the service factor X.If the population consists of 5 elements,what are the average number waiting,the average number being serviced,and the average number running? Refer to Table D.8.

Accepted Answer

The service factor is X = T / (T + U)= 2

Question 15

A waiting-line system that meets the assumptions of M/M/1 has λ = 1,μ = 4.For this system,the probability of more than two units in the system is approximately

Accepted Answer

A) zero. 
B) 0.015625. 
C) 0.0625. 
D) 0.25. 
E) 0.9375. 
A) zero. 
B) 0.015625. 
C) 0.0625. 
D) 0.25. 
E) 0.9375.

Question 16

Why does it matter whether a population of arrivals is limited or unlimited? Compose your answer in a well-organized,convincing paragraph.

Accepted Answer

If an arrival population is unlimited,th

Question 17

A waiting line has a(n)________ population if,as arrivals take place,the likelihood of additional arrivals is decreased.

Accepted Answer

The answer of A waiting line has a(n)________ population if,as...

Question 18

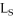A queuing model which follows the M/M/1 assumptions has λ = 2 and μ = 8.The average number in the system   is ________ and the utilization of the system is ________.

Accepted Answer

A) 3;100% 
B) 0.33;25% 
C) 4;33% 
D) 6;25% 
E) 4;25% 
A) 3;100% 
B) 0.33;25% 
C) 4;33% 
D) 6;25% 
E) 4;25%

Question 19

Suppose that a service facility has an average line of 2 customers that must wait,on average,5 minutes for service.How many customers are arriving per hour?

Accepted Answer

Use Little's Law that 2 custom

Question 20

A finite population waiting line model has an average service time T of 200 minutes and an average time between service requirements U of 300 minutes;the service factor X is

Accepted Answer

A) 0.20. 
B) 0.40. 
C) 0.60. 
D) 0.67. 
E) 2.5. 
A) 0.20. 
B) 0.40. 
C) 0.60. 
D) 0.67. 
E) 2.5.

Exam 24: Waiting-Line Models

The ________ of a waiting line and the probability that the queue is empty add to one.

The sign at the bank that reads "Wait here for the first available teller" suggests the use of a ________ waiting line system.

In queuing problems,arrival rates are generally described by the normal probability distribution.

A bank office with five tellers,each with a separate line of customers,exhibits the characteristics of a multi-phase queuing system.

A waiting-line system that meets the assumptions of M/M/1 has λ = 1,μ = 4.For this system,Po is ________ and utilization is ________.

Provide an example of a limited or finite population for a queue.

A university has only one technician in the repair station to care for the computers in the student labs.This system is most likely

Which of the following is not a common queuing situation?

A finite population waiting line model has an average service time T of 100 minutes and an average time between service requirements U of 400 minutes;the service factor X is

Describe the important operating characteristics of a queuing system.

Which one of the following is not a characteristic of a Model A or M/M/1 system?

A queuing model which follows the M/M/1 assumptions has λ = 2 and μ = 3.The average waiting time in the system is

A waiting-line system that meets the assumptions of M/M/1 has λ = 1,μ = 4.For this system,the probability of more than two units in the system is approximately

Why does it matter whether a population of arrivals is limited or unlimited? Compose your answer in a well-organized,convincing paragraph.

A waiting line has a(n)________ population if,as arrivals take place,the likelihood of additional arrivals is decreased.

A queuing model which follows the M/M/1 assumptions has λ = 2 and μ = 8.The average number in the system is ________ and the utilization of the system is ________.

Suppose that a service facility has an average line of 2 customers that must wait,on average,5 minutes for service.How many customers are arriving per hour?

A finite population waiting line model has an average service time T of 200 minutes and an average time between service requirements U of 300 minutes;the service factor X is

Operations and Productivity

Operations Strategy in a Global Environment

Project Management

Forecasting

Design of Goods and Services

Managing Quality

Statistical Process Control

Process Strategy and Sustainability

Capacity and Constraint Management

Location Strategies

Layout Strategies

Human Resources,job Design,and Work Measurement

Supply-Chain Management

Outsourcing As a Supply-Chain Strategy

Inventory Management

Aggregate Planning

Material Requirements Planning Mrpand Erp

Short-Term Scheduling

Jit and Lean Operations

Maintenance and Reliability

Decision-Making Tools

Linear Programming

Transportation Models

Learning Curves

Simulation

Filters

A waiting-line system that meets the assumptions of M/M/1 has λ = 1,μ = 4.For this system,Po is and utilization is .

A queuing model which follows the M/M/1 assumptions has λ = 2 and μ = 8.The average number in the system is and the utilization of the system is .