Exam 16: A: Simple Linear Regression and Correlation

Rock Concert Revenues A financier whose specialty is investing in rock concerts has observed that,in general,concerts with "big-name" stars seem to generate more revenue than those concerts whose stars are less well known.To examine his belief he records the gross revenue and the payment (in $ millions)given to the two highest-paid performers in the concert for ten concert tours. Concert Cost of Twa Highest Paid Perfarmers ( \mil ) Grass Revenue () 1 5.3 48 2 7.2 65 3 1.3 18 4 1.8 20 5 3.5 31 6 2.6 26 7 8.0 73 8 2.4 23 9 4.5 39 10 0.7 58 -{Rock Concert Revenues Narrative} Determine the coefficient of determination and discuss what its value tells you about the two variables.

The confidence interval estimate of the expected value of y will be narrower than the prediction interval for the same given value of x and confidence level.This is because there is less error in estimating a mean value as opposed to predicting an individual value.

U V's and Skin Cancer A medical statistician wanted to examine the relationship between the amount of UV's (x)and incidence of skin cancer (y).As an experiment he found the number of skin cancers detected per 100,000 of population and the average daily sunshine in eight states around the country.These data are shown below. Average Daily UV's 5 7 6 7 8 6 4 3 Skin Cancer per 100,000 7 11 9 12 15 10 7 5 -(Sales and Experience Narrative} Determine the coefficient of determination and discuss what its value tells you about the two variables.

Wayne Newton Concert At a recent Wayne Newton concert,a survey was conducted that asked a random sample of 20 people their age and how many concerts they have attended since the first of the year.The following data were collected: Age 62 57 40 49 67 54 43 65 54 41 Number af Concerts 6 5 4 3 5 5 2 6 3 1 Age 44 48 55 60 59 63 69 40 38 52 Number af Concerts 3 2 4 5 4 5 4 2 1 3 An Excel output follows: 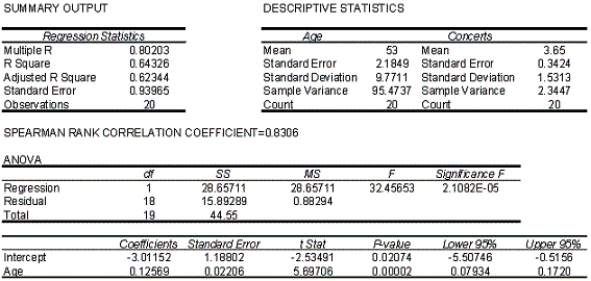-{Oil Quality and Price Narrative} Predict with 95% confidence the oil price per barrel for an API degree of 35.

Speed vs Gas Mileage An economist wanted to analyze the relationship between the speed of a car (x)and its gas mileage (y).As an experiment a car is operated at several different speeds and for each speed the gas mileage is measured.These data are shown below. Speed 25 35 45 50 60 65 70 Gas Mileage 40 39 37 33 30 27 25 -{Car Speed and Gas Mileage Narrative} Determine the least squares regression line.

A regression analysis between sales (in $)and advertising (in $)resulted in the following least squares line: $\hat { y } = 60 + 5 x$ .This implies that an increase of $1 in advertising is associated with an increase of $60 in sales.

Trivia Games & Education An ardent fan of television game shows has observed that,in general,the more educated the contestant,the less money he or she wins.To test her belief she gathers data about the last eight winners of her favorite game show.She records their winnings in dollars and the number of years of education.The results are as follows. Contestant Years of Education Winnings 1 11 750 2 15 400 3 12 600 4 16 350 5 11 800 0 16 300 7 13 650 8 14 400 -{Trivia Games & Education Narrative} Determine the least squares regression line.

If the standard error of estimate is zero,then:

Sunshine and Melanoma A medical researcher wanted to examine the relationship between the amount of sunshine (x)in hours,and incidence of melanoma,a type of skin cancer (y).As an experiment he found the number of melanoma cases detected per 100,000 of population and the average daily sunshine in eight counties around the country.These data are shown below. Average Daily Sunshine 5 7 6 7 8 6 4 3 Melanoma per 100,000 7 11 9 12 15 10 7 5 -{Sunshine and Melanoma Narrative} Draw a scatter diagram of the data and plot the least squares regression line on it.

If you take a particular x value and plug it into a regression line equation,the result is a(n)____________________ estimate for y.

If an estimated regression line has a y-intercept of 10 and a slope of 4,then when x = 2 the actual value of y is:

If the coefficient of correlation is -0.60,then the coefficient of determination is:

Truck Speed and Gas Mileage An economist wanted to analyze the relationship between the speed of a truck (x)and its gas mileage (y).As an experiment a truck is operated at several different speeds and for each speed the gas mileage is measured.These data are shown below. Speed 25 35 45 50 60 65 70 Gas Mileage 40 39 37 33 30 27 25 -{Truck Speed and Gas Mileage Narrative} Calculate the standard error of estimate,and describe what this statistic tells you about the regression line.

Theatre Revenues A financier whose specialty is investing in stage productions has observed that,in general,movies with "big-name" stars seem to generate more revenue than those plays whose stars are less well known.To examine his belief he records the gross revenue and the payment (in $ millions)given to the two highest-paid performers in the play for ten recently staged plays. Play Cost af Twa Highest Paid Perfarmers (4mil) Grass Revenue () 1 5.3 48 2 7.2 65 3 1.3 18 4 1.8 20 5 3.5 31 6 2.6 26 7 8.0 73 8 2.4 23 9 4.5 39 10 0.7 58 -{Theatre Revenues Narrative} Estimate the gross revenue of a play if the two highest paid performers received 6 million dollars.

Oil Quality and Price Quality of oil is measured in API gravity degrees--the higher the degrees API,the higher the quality.The table shown below is produced by an expert in the field who believes that there is a relationship between quality and price per barrel. $\begin{array} { | c | c | } \hline \text { Oi degrees API } & \text { Price per barrel (in \$) } \\\hline 27.0 & 12.02 \\28.5 & 12.04 \\30.8 & 12.32 \\31.3 & 12.27 \\31.9 & 12.49 \\34.5 & 12.70 \\34.0 & 12.80 \\34.7 & 13.00 \\37.0 & 13.00 \\41.0 & 13.17 \\41.0 & 13.19 \\38.8 & 13.22 \\39.3 & 13.27 \\\hline\end{array}$ A partial Minitab output follows: Dascriptive atafistics Variable Mear StDev SE Mear Degrees 13 34.60 4.613 1.280 Price 13 1270 0.757 0.127 Covariances Degeres Price Degeres 21.281667 Price 2.026750 0.208933 Analysis of Variance Source DF SS MS F p Regeression 1 2.3162 2.3162 134.24 0.000 Resichul Entar 11 0.1898 0.0173 Total 12 2.5060 -If the coefficient of correlation is 1.0,then the coefficient of determination must be 1.0.

In a regression problem the following pairs of (x,y)are given: (3,1), (3,-1), (3,0), (3,-2)and (3,2).That indicates that the:

To create a deterministic model,we start with a probabilistic model that approximates the relationship we want to model.

Sales and Experience The general manager of a chain of department stores believes that experience is the most important factor in determining the level of success of a salesperson.To examine this belief she records last month's sales (in $1,000s)and the years of experience of 10 randomly selected salespeople.These data are listed below. Salesperson Years of Exgperience Sales 1 0 7 2 2 9 3 10 20 4 3 15 5 8 18 6 5 14 7 12 20 8 7 17 9 20 30 10 15 25 -{Sales and Experience Narrative} Estimate the monthly sales for a salesperson with 16 years of experience.

U V's and Skin Cancer A medical statistician wanted to examine the relationship between the amount of UV's (x)and incidence of skin cancer (y).As an experiment he found the number of skin cancers detected per 100,000 of population and the average daily sunshine in eight states around the country.These data are shown below. Average Daily UV's 5 7 6 7 8 6 4 3 Skin Cancer per 100,000 7 11 9 12 15 10 7 5 -{Sales and Experience Narrative} Conduct a test of the population coefficient of correlation to determine at the 5% significance level whether more experience is related to higher sales,as the manager speculates.

Income and Education A professor of economics wants to study the relationship between income (y in $1000s)and education (x in years).A random sample eight individuals is taken and the results are shown below. Education 16 11 15 8 12 10 13 14 Income 58 40 55 35 43 41 52 49 -{Income and Education Narrative} Determine the least squares regression line.