Exam 17: Multiple Regression
Exam 1: What Is Statistics43 Questions
Exam 2: Graphical Descriptive Techniques I93 Questions
Exam 3: Graphical Descriptive Techniques II183 Questions
Exam 4: Numerical Descriptive Techniques274 Questions
Exam 5: Data Collection and Sampling82 Questions
Exam 6: Probability234 Questions
Exam 7: Random Variables and Discrete Probability Distributions274 Questions
Exam 8: Continuous Probability Distributions215 Questions
Exam 9: Sampling Distributions153 Questions
Exam 10: Introduction to Estimation152 Questions
Exam 11: Introduction to Hypothesis Testing182 Questions
Exam 12: Inference About a Population147 Questions
Exam 13: Inference About Comparing Two Populations170 Questions
Exam 14: Analysis of Variance153 Questions
Exam 15: Chi-Squared Tests175 Questions
Exam 16: Simple Linear Regression and Correlation298 Questions
Exam 17: Multiple Regression157 Questions
Select questions type
Consider the following statistics of a multiple regression model: Total variation in y = 1000, SSE = 300, n = 50, and k = 4.
a.
Determine the standard error of estimate.
b.
Determine the coefficient of determination.
c.
Determine the F-statistic.
(Essay)
4.9/5  (42)
(42)
If the value of the Durbin-Watson statistic d is large (d > 2), this indicates a(n) ____________________ (positive/negative) first-order autocorrelation exists.
(Short Answer)
4.9/5  (28)
(28)
The range of the values of the Durbin-Watson statistic, d, is 0 d 4.
(True/False)
4.8/5  (41)
(41)
From the coefficient of determination, we cannot detect the strength of the relationship between the dependent variable y and any individual independent variable.
(True/False)
4.8/5  (42)
(42)
Real Estate Builder
A real estate builder wishes to determine how house size is influenced by family income, family size, and education of the head of household.House size is measured in hundreds of square feet, income is measured in thousands of dollars, and education is measured in years.A partial computer output is shown below.
SUMMARY OUTPUT
 ANOVA
ANOVA

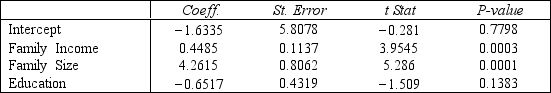 -{Real Estate Builder Narrative} Which of the following values for the level of significance is the smallest for which the regression model as a whole is significant: = .00005, .001, .01, and .05?
-{Real Estate Builder Narrative} Which of the following values for the level of significance is the smallest for which the regression model as a whole is significant: = .00005, .001, .01, and .05?
(Short Answer)
4.9/5  (26)
(26)
Consider the following statistics of a multiple regression model: n = 25, k = 5, b1 = -6.31, and s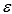 = 2.98.Can we conclude at the 1% significance level that x1 and y are linearly related?
= 2.98.Can we conclude at the 1% significance level that x1 and y are linearly related?
(Essay)
4.9/5  (36)
(36)
A multiple regression model is assessed to be poor if the error sum of squares SSE and the standard error of estimate 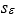 are both large, the coefficient of determination R2 is close to 0, and the value of the test statistic F is large.
are both large, the coefficient of determination R2 is close to 0, and the value of the test statistic F is large.
(True/False)
4.9/5  (46)
(46)
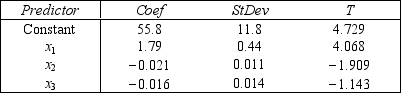
Life Expectancy
An actuary wanted to develop a model to predict how long individuals will live.After consulting a number of physicians, she collected the age at death (y), the average number of hours of exercise per week (x1), the cholesterol level (x2), and the number of points that the individual's blood pressure exceeded the recommended value (x3).A random sample of 40 individuals was selected.The computer output of the multiple regression model is shown below.
THE REGRESSION EQUATION IS
y = 55.8 + 1.79x1 - 0.021x2 -0.061x3
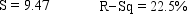 ANALYSIS OF VARIANCE
ANALYSIS OF VARIANCE
 -{Life Expectancy Narrative} What is the coefficient of determination? What does this statistic tell you?
-{Life Expectancy Narrative} What is the coefficient of determination? What does this statistic tell you?
(Essay)
4.7/5  (38)
(38)
When there is more than one independent variable in a regression model, we refer to the graphical depiction of the equation as a(n) ____________________ rather than as a straight line.
(Short Answer)
4.8/5  (40)
(40)
Multicollinearity will result in excessively low standard errors of the parameter estimates reported in the regression output.
(True/False)
4.9/5  (32)
(32)
Student's Final Grade
A statistics professor investigated some of the factors that affect an individual student's final grade in her course.She proposed the multiple regression model 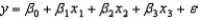 , where y is the final grade (out of 100 points), x1 is the number of lectures skipped, x2 is the number of late assignments, and x3 is the midterm exam score (out of 100).The professor recorded the data for 50 randomly selected students.The computer output is shown below.
THE REGRESSION EQUATION IS
, where y is the final grade (out of 100 points), x1 is the number of lectures skipped, x2 is the number of late assignments, and x3 is the midterm exam score (out of 100).The professor recorded the data for 50 randomly selected students.The computer output is shown below.
THE REGRESSION EQUATION IS 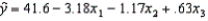

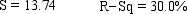 ANALYSIS OF VARIANCE
ANALYSIS OF VARIANCE
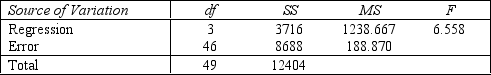 -{Student's Final Grade Narrative} Does this data provide enough evidence to conclude at the 5% significance level that the model is useful in predicting the final grade?
-{Student's Final Grade Narrative} Does this data provide enough evidence to conclude at the 5% significance level that the model is useful in predicting the final grade?
(Essay)
4.8/5  (32)
(32)
In calculating the standard error of the estimate, 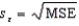 , there are (n - k-1) degrees of freedom, where n is the sample size and k is the number of independent variables in the model.
, there are (n - k-1) degrees of freedom, where n is the sample size and k is the number of independent variables in the model.
(True/False)
4.8/5  (38)
(38)
Real Estate Builder
A real estate builder wishes to determine how house size is influenced by family income, family size, and education of the head of household.House size is measured in hundreds of square feet, income is measured in thousands of dollars, and education is measured in years.A partial computer output is shown below.
SUMMARY OUTPUT
 ANOVA
ANOVA

 -{Real Estate Builder Narrative} Which of the independent variables in the model are significant at the 2% level?
-{Real Estate Builder Narrative} Which of the independent variables in the model are significant at the 2% level?
(Short Answer)
4.9/5  (42)
(42)
Multicollinearity is present if the dependent variable is linearly related to one of the explanatory variables.
(True/False)
4.9/5  (28)
(28)
We test an individual coefficient in a multiple regression model using a(n) _________ test.
(Short Answer)
4.8/5  (34)
(34)
If the value of the Durbin-Watson test statistic, d, satisfies the inequalities d < dL or d > 4 - dL, where dL and dU are the critical values of d, we conclude that autocorrelation exists.
(True/False)
4.8/5  (33)
(33)
Multicollinearity is a situation in which two or more of the independent variables are highly correlated with each other.
(True/False)
4.8/5  (23)
(23)
Student's Final Grade
A statistics professor investigated some of the factors that affect an individual student's final grade in her course.She proposed the multiple regression model 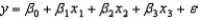 , where y is the final grade (out of 100 points), x1 is the number of lectures skipped, x2 is the number of late assignments, and x3 is the midterm exam score (out of 100).The professor recorded the data for 50 randomly selected students.The computer output is shown below.
THE REGRESSION EQUATION IS
, where y is the final grade (out of 100 points), x1 is the number of lectures skipped, x2 is the number of late assignments, and x3 is the midterm exam score (out of 100).The professor recorded the data for 50 randomly selected students.The computer output is shown below.
THE REGRESSION EQUATION IS 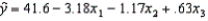

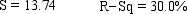 ANALYSIS OF VARIANCE
ANALYSIS OF VARIANCE
 -{Student's Final Grade Narrative} Interpret the coefficient b3.
-{Student's Final Grade Narrative} Interpret the coefficient b3.
(Essay)
4.7/5  (29)
(29)
Showing 81 - 100 of 157
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)