Exam 5: Discrete Probability Distributions

A package delivery service claims that no more than 5 percent of all packages arrive at the address late. Assuming that the conditions for the binomial hold, if a sample of size 10 packages is randomly selected and the 5 percent rate holds, what is the probability that more than 2 packages will be delivered late?

The time required to assemble two components into a finished part is recorded for each employee at the plant. The resulting random variable is an example of a continuous random variable.

The roll of a pair of dice has the following probability distribution, where the random variable is the sum of the values produced by each die: 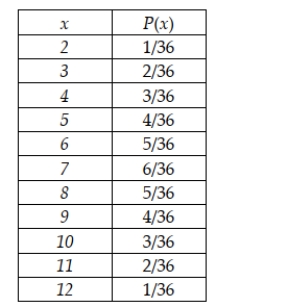Calculate the standard deviation of x.

Assuming that potholes occur randomly along roads, the number of potholes per mile of road could best be described by the:

For a binomial distribution with a sample size equal to 10 and a probability of a success equal to 0.30, what is the probability that the sample will contain exactly three successes? Use the binomial formula to determine the probability.

John Thurgood founded a company that translates Chinese books into English. His company is currently testing a computer-based translation service. Since Chinese symbols are difficult to translate, John assumes the computer program will make some errors, but then so do human translators. The computer error rate is supposed to be an average of 3 per 400 words of translation. Suppose John randomly selects a 1,200-word passage. Assuming that the Poisson distribution applies, if the computer error rate is actually 3 errors per 400 words, determine the probability that no errors will be found.

The Argon Construction Company has the opportunity to enter into a contract to remodel a building. The following table shows the probability distribution for the profit that could occur if it takes the contract: Based on this information, the expected profit for the company if it takes the contract is $67,000.

College-Pro Painting does home interior and exterior painting. The company uses inexperienced painters that do not always do a high-quality job. It believes that its painting process can be described by a Poisson distribution with an average of 4.8 defects per 400 square feet of painting. What is the probability that a 400-square-foot painted section will have fewer than 6 blemishes?

The Ace Construction Company has entered into a contract to widen a street in Boston. The possible payoffs for this project have been determined by management. The probabilities for these payoffs could be determined using a binomial distribution.

The following probability distribution has been assessed for the number of accidents that occur in a Midwestern city each day: 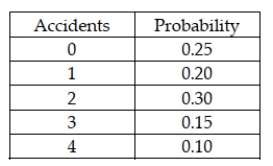This distribution is an example of:

A sales rep for a national clothing company makes 4 calls per day. Based on historical records, the following probability distribution describes the number of successful calls each day: 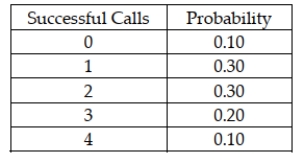The expected number of successful sales calls per day is:

A random variable is generated when a variable's value is determined by using classical probability.

If cars arrive to a service center randomly and independently at a rate of 5 per hour on average, what is the probability of 0 cars arriving in a given hour?

The Colbert Real Estate Agency has determined the number of home showings given by its agents is the same each day of the week. Then the variable, number of showings, is a continuous distribution.

A population of 10 items contains 3 that are red and 7 that are green. What is the probability that in a random sample of 3 items selected without replacement, 2 red and 1 green items are selected?

If a binomial distribution applies with a sample size of n = 20, find the expected value, n = 20, p = 0.20.

Beacon Hill Trees & Shrubs currently has an inventory of 10 fruit trees, 8 pine trees, and 14 maple trees. It plans to give 4 trees away at next Saturday's lawn and garden show in the city park. The 4 winners can select which type of tree they want. Assume they select randomly. What is the probability that no fruit trees and 2 of each of the others will be selected?

When a single value is randomly chosen from a discrete distribution, the different possible values are mutually exclusive.

Many people believe that they can tell the difference between Coke and Pepsi. Other people say that the two brands can't be distinguished. To test this, a random sample of 20 adults was selected to participate in a test. After being blindfolded, each person was given a small taste of either Coke or Pepsi and asked to indicate which brand soft drink it was. If people really can't tell the difference, the expected number of correct identifications in the sample would be:

The city council consists of 3 Democrats, 5 Republicans and 3 independents. Subcommittees are supposed to be randomly assigned from the council. Suppose the 5-member planning and zoning subcommittee is composed of 3 Democrats and 2 Republicans. The probability of this happening by chance alone is approximately .4545.