Exam 6: Matrices and Determinants

Find the product AB, if possible. - $A = \left[ \begin{array} { r r r } 1 & 3 & - 2 \\2 & 0 & 5\end{array} \right] , B = \left[ \begin{array} { r r } 3 & 0 \\- 2 & 1 \\0 & 5\end{array} \right]$

Write the augmented matrix for the system of equations. - 4x+3z=33 9y+5z=42 6x-2y+8z=54

Solve Problems Involving Systems Without Unique Solutions Solve the problem using matrices. -The nutritional content per ounce for three foods is given in the table below. 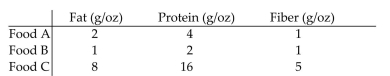What combination of these foods can provide exactly 14 grams of fat, 27 grams of protein, and 10 grams of fiber?

Find the products AB and BA to determine whether B is the multiplicative inverse of A. - $A = \left[ \begin{array} { r r r r } 1 & 0 & 0 & - 1 \\2 & 1 & 0 & 0 \\1 & 1 & 1 & - 2 \\0 & 0 & 0 & 1\end{array} \right] , \quad B = \left[ \begin{array} { r r r r } 1 & 0 & 0 & 1 \\- 2 & 1 & 0 & - 2 \\1 & - 1 & 1 & 3 \\0 & 0 & 0 & 1\end{array} \right]$

Solve the matrix equation for X. -Let $\mathrm { A } = \left[ \begin{array} { r r } 3 & 3 \\ - 3 & 1 \end{array} \right]$ and $\mathrm { B } = \left[ \begin{array} { r r } - 3 & 2 \\ 3 & - 2 \end{array} \right] ; \quad \mathrm { X } + \mathrm { A } = \mathrm { B }$

Find the products AB and BA to determine whether B is the multiplicative inverse of A. - $A = \left[ \begin{array} { r r } - 5 & - 1 \\ 6 & 0 \end{array} \right] , \quad B = \left[ \begin{array} { c } 0 \frac { 1 } { 6 } \\ - 1 \frac { 5 } { 6 } \end{array} \right]$

Apply Gaussian Elimination to Systems with More Variables than Equations Use Gaussian elimination to find the complete solution to the system of equations, or state that none exists. - 3x+y+z-2w=10 2x+3y+3z+w=-5 2x+y+4z+11w=11

Solve the system using the inverse that is given for the coefficient matrix. - $x + 2 y + 3 z = 2$ $x + y + z = 1$ $- x + y + 2 z = - 1$$\quad$ The inverse of $\left[ \begin{array} { r r r } 1 & 2 & 3 \\ 1 & 1 & 1 \\ - 1 & 1 & 2 \end{array} \right]$ is $\left[ \begin{array} { r r r } 1 & - 1 & - 1 \\ - 3 & 5 & 2 \\ 2 & - 3 & - 1 \end{array} \right]$

Write the system of linear equations represented by the augmented matrix. Use x, y, z, and, if necessary, w for the variables. - 7x+8y-12z+w=9 4y+z=10 x-y-3z=-1 5x-5y+2z=2

Model Applied Situations with Matrix Operations The $\perp$ shape in the figure below is shown using 9 pixels in a $3 \times 3$ grid. The color levels are given to the right of the figure. Use the matrix $\left[ \begin{array} { l l l } 1 & 3 & 1 \\ 1 & 3 & 1 \\ 3 & 3 & 3 \end{array} \right]$ that represents a digital photograph of the $\perp$ shape to solve the problem. -Adjust the contrast by changing the black to light grey and the light grey to black. Use matrix addition to accomplish this.

Evaluate the determinant. - $\left| \begin{array} { l l l } 2 & 0 & 0 \\ 3 & 7 & 8 \\ 7 & 9 & 8 \end{array} \right|$

Write the system of linear equations represented by the augmented matrix. Use x, y, z, and, if necessary, w for the variables. Then use back-substitution to find the solution. - $\left[ \begin{array} { r r r | r } 1 & 6 & 7 & - 3 \\ 0 & 1 & 9 & 3 \\ 0 & 0 & 1 & - 8 \end{array} \right]$

Find the product AB, if possible. - $A = \left[ \begin{array} { l l l } - 3 & 2 & 9 \end{array} \right] , \quad B = \left[ \begin{array} { r } 7 \\ 0 \\ - 3 \end{array} \right]$

Use Matrices and Gaussian Elimination to Solve Systems Solve the system of equations using matrices. Use Gaussian elimination with back-substitution. - 3x+5y-2w= -13 2x+7z-w= -1 4y+3z+3w= 1 -x+2y+4z= -5

Use Inverses to Solve Matrix Equations Write the linear system as a matrix equation in the form AX = B, where A is the coefficient matrix and B is the constant matrix. - 8x+4y=20 3x+5y=4

Use Matrices and Gaussian Elimination to Solve Systems Solve the system of equations using matrices. Use Gaussian elimination with back-substitution. - -5x-y+6z =-29 -8x-9z =-99 2y+z =7

Use Cramer's rule to determine if the system is inconsistent system or contains dependent equations. - 6x+9y=15 12x+18y=13

Apply Gaussian Elimination to Systems Without Unique Solutions Use Gaussian elimination to find the complete solution to the system of equations, or state that none exists. - x+3y+2z=11 4y+9z=-12 x+7y+11z=-1

Understand What is Meant by Equal Matrices Find values for the variables so that the matrices are equal. - $\left[\begin{array}{rr}5 & -6 \\ -2 & 3\end{array}\right]=\left[\begin{array}{r}x y \\ -2 z\end{array}\right]$

Use Matrices and Gauss-Jordan Elimination to Solve Systems Solve the system of equations using matrices. Use Gauss-Jordan elimination. - x+y-z+w =-5 3x-y+3z-2w =7 -2x+2y+z-w =16 -x-2y-3z+3w =-22