Exam 1: Functions and Graphs
Exam 1: Functions and Graphs362 Questions
Exam 2: Polynomial, Power, and Rational Functions494 Questions
Exam 3: Exponential, Logistic, and Logarithmic Functions350 Questions
Exam 4: Trigonometric Functions522 Questions
Exam 5: Analytic Trigonometry313 Questions
Exam 6: Applications of Trigonometry333 Questions
Exam 7: Systems and Matrices354 Questions
Exam 8: Analytic Geometry in Two and Three Dimensions167 Questions
Exam 9: Discrete Mathematics154 Questions
Exam 10: Statistics and Probability147 Questions
Exam 11: An Introduction to Calculus: Limits, Derivatives, and Integrals167 Questions
Exam 12: Prerequisites382 Questions
Select questions type
Consider the functions f and g as shown in the graph. Sketch the graph of the indicated sum or difference of functions.
-Graph - f.

(Multiple Choice)
4.8/5  (33)
(33)
Graph the function on your calculator to determine the domain and range from the graph.
-
(Multiple Choice)
4.7/5  (33)
(33)
Solve the problem.
-Determine graphically the local maximum and local minimum of 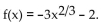
(Multiple Choice)
4.8/5  (36)
(36)
Determine if the function is bounded above, bounded below, bounded on its domain, or unbounded on its domain.
-
(Multiple Choice)
4.7/5  (34)
(34)
Choose the one alternative that best completes the statement or answers the question.
Solve the problem.
-Let compute the time in hours to travel miles at 36 miles per hour. What is the interpretation the solution of ?
(Multiple Choice)
4.8/5  (30)
(30)
Find the (x,y) pair for the value of the parameter.
- and for
(Multiple Choice)
4.8/5  (27)
(27)
Perform the requested operation or operations. Find the domain of each.
- Find
(Multiple Choice)
4.8/5  (29)
(29)
Use an equation to solve the problem.
-Joe Pearlman received a pay decrease. His salary after the decrease was . What was his salary before the decrease?
(Multiple Choice)
4.9/5  (45)
(45)
Find the asymptote(s) of the given function.
- horizontal asymptotes(s)
(Multiple Choice)
4.8/5  (36)
(36)
Describe how to transform the graph of f into the graph of g.
-
(Multiple Choice)
4.8/5  (33)
(33)
Write the specified quantity as a function of the specified variable.
-The base of an isosceles triangle is a fourth as long as the two equal sides. Write the area of the triangle as a function of the length of the base.
(Multiple Choice)
4.9/5  (36)
(36)
Determine algebraically whether the function is even, odd, or neither even nor odd.
-
(Multiple Choice)
4.8/5  (40)
(40)
Fill in the blanks to complete the statement.
-The graph of can be obtained from the graph of by shifting horizontally![Fill in the blanks to complete the statement. -The graph of y = - \frac { 1 } { 7 } \sqrt [ 3 ] { x + 9 } can be obtained from the graph of y = \sqrt [ 3 ] { x } by shifting horizontally units to the , vertically shrinking by a factor of , and then reflecting across the -axis.](https://storage.examlex.com/TB8181/11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00.jpg) units to the11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00 , vertically shrinking by a factor of11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00 , and then reflecting across the11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00 -axis.
units to the11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00 , vertically shrinking by a factor of11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00 , and then reflecting across the11ecc462_eee5_8183_841e_b1b874fd7cdc_TB8181_00 -axis.
(Multiple Choice)
4.8/5  (24)
(24)
Identify intervals on which the function is increasing, decreasing, or constant.
-
(Multiple Choice)
4.8/5  (37)
(37)
Use an equation to solve the problem.
-A construction company builds a swimming pool with a perimeter of . The length is more than the width. Find the dimensions of the swimming pool?
(Multiple Choice)
4.9/5  (24)
(24)
Determine algebraically whether the function is even, odd, or neither even nor odd.
-
(Multiple Choice)
4.9/5  (34)
(34)
Showing 121 - 140 of 362
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)