Exam 5: Operational Amplifier Circuits
Exam 1: Voltage, Current, Power, and Sources10 Questions
Exam 2: Circuit Laws10 Questions
Exam 3: Circuit Analysis Methods20 Questions
Exam 4: Circuit Theorems13 Questions
Exam 5: Operational Amplifier Circuits7 Questions
Exam 6: Capacitors and Inductors10 Questions
Exam 7: RL and RC Circuits14 Questions
Exam 8: RLC Circuits8 Questions
Exam 9: Phasors and Impedances8 Questions
Exam 10: Analysis of Phasor Transformed Circuits15 Questions
Exam 11: AC Power5 Questions
Exam 12: Three-Phase Systems6 Questions
Exam 13: Magnetically Coupled Circuits6 Questions
Exam 14: The Laplace Transform19 Questions
Exam 15: Circuits Analysis in the S-Domain26 Questions
Select questions type
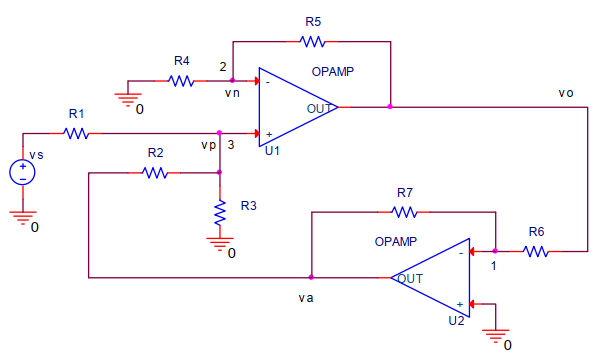
the circuit shown below, let
(a) write a node equation at node 1 by summing the currents leaving node 1 . Express as a function of .
(b) write a node equation at node 2 by summing the currents leaving node 2 . Express as a function of .
(c) write a node equation at node 3 by summing the currents leaving node 3 .
(d) find the numerical value of .
Free
(Essay)
4.9/5  (32)
(32)
Correct Answer:
(a)
(b)
(c)
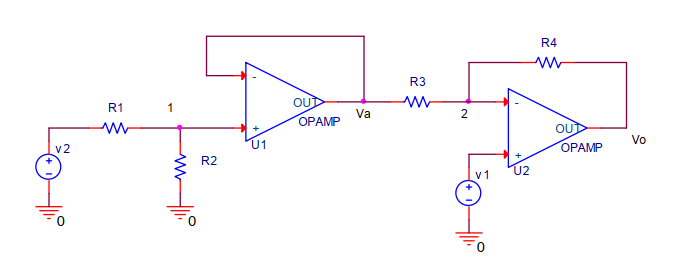
circuit shown below generates output signal vo given by
where and are two input signals. Find , and .
Free
(Essay)
4.8/5  (40)
(40)
Correct Answer:
One solution: There are many solutions. Superposition principle can be used to show that the answer is correct.
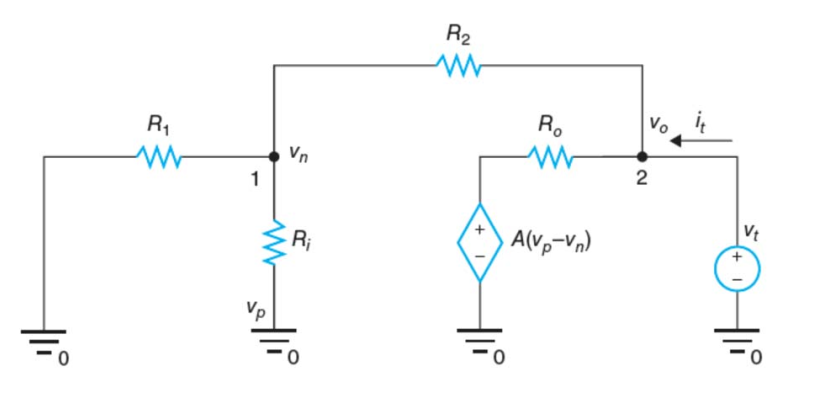
the circuit shown below, let
Find the value of and the output resistance .
Free
(Essay)
4.9/5  (42)
(42)
Correct Answer:
clear all;
syms vn
Rout =vt/uti
vh=vpa (vn, 1l)
it=vpa
Rout=vpa (Rout, 11)
Answers:
it
1.6819959677
Rout
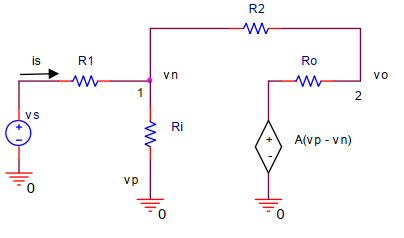
In the circuit shown below, let
(a) Write a node equation at node 1 by summing currents leaving node 1 .
(b) Write a node equation at node 2 by summing currents leaving node 2
(c) Solve the two node equations to find numerical values of and .
(d) Find the value of through . Find the input impedance .
(Essay)
4.8/5  (34)
(34)
the circuit shown below, let
(a) Write a node equation at node 1 by summing currents away from node 1 .
(b) Write a node equation at node 2 by summing currents away from node 2 .
(c) Solve the two node equations to find numerical values of and .
(d) Find the value of through . Find the input impedance .
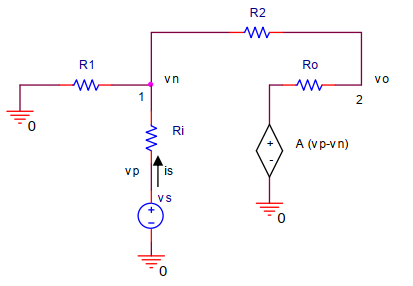
(Essay)
4.8/5  (41)
(41)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)